An ideal monoatomic gas is confined in a horizontal cylinder by a spring loaded piston (as shown in the figure). Initially the gas is at temperature T1, pressure P1 and volume V1and the spring is in its relaxed state. The gas is tehn heated very slowly to temperature T2,pressure P2and volume V2. During this process the piston moves out by a distance x. Ignoring the friction between the piston and the cylinder, the correct statement (s) is (are)
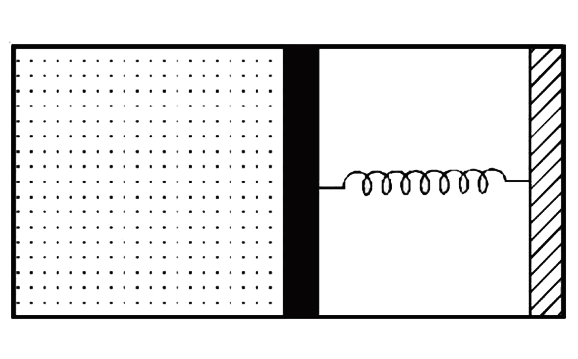
If V2=2V1andT2=3T1, then the o the energy stored in the spiring is 14P1V1
If V2=2V1andT2=3T1, then the change in internal energy is 3P1V1
If V2=3V1andT2=4T1, then the work done by the gas is 73P1V1
If V2=3V1andT2=4T1, then the heat supplied to the gas is 176P1V1
The correct Answer is:B, C
(b,c)
Applying combined gas law
P1V1T1=P2V2T2
If V2=2V1andT2=3T1 then
P1V1T1=P2×2V13T1⇒P1=23P2
Now change in internal energy
ΔU=f2[nR(T2−T1)]=f2[P2V2−P1V1]
For monoatomic gas f=3
ΔU=32[32P1×2V1−PV_1]=3P1V1
∴(b)isthecorrectoption.<br>Nowas∑∈gtˆthepressureontheπs→nontheright<br>hands¬consr∈gtheaffectofsπr∈grema∈sthe<br>samethroughoutthemotionoftheπs→nthen,<br>Pressure of gas=P_1+(kx)/A rArr P_2=P_1+(kx)/A<br>Wherekisspr∈gconstantandA = area of piston<br>Energy stored=1/2kx^2<br>P_2=P_1+(kx)/A rArr 3/2P_1=P_1+(kx)/A<br>(P_1)/2=(kx)/2<br>:. kx=(P_1A)/2<br>Also,<br>V_2=V_1+Ax<br>V_1=Ax<br>:. x=(V_1)/A<br>:. Energy =1/2(P_1A)/2xx(V_1)/A=1/4P_1V_1<br>:. A is correct <br>Now<br>w=int pdV=int(P_1+(kx)/A)dV=intP_1dV+int(kx)/adV<br>:. W=intP_1dV+int(kx)/Axx(dx)A<br>:. W=P_1(V_2-V_1)+(kx^2)/2<br>[Here on applying P1V1T1=P2V2T2we≥tP2=4P13<br>andV_2=V_1+Ax rArr x=(2V_1)/A[:' V_2=3V_1]]<br>:. W=2P_1V_1+1/2xx(P_1A)/3xx(2V_1)/A=7/3P_1V_1<br>Ciscorrectoption<br>Heat⊃plied<br>Q=W+DeltaU<br>=(7P_1V_1)/3+3/2(P_2V_2-P_1V_1)<br>=(7P_1V_1)/3+3/2[4/3P_13V_1-P_1V_1]=41/4P_1V_1
Topper's Solved these Questions
Similar Questions
An ideal monatomic gas is confined in a cylinder by a spring-loaded piston of cross-section 8.0×10−3m2. Initially the gas is at 300K and occupies a volume of 2.4×10−3m3 and the spring is in its relaxed (unstretched, unompressed) state, fig. The gas is heated by a small electric heater until the piston moves out slowly by 0.1m. Calculate the final temperature of the gas and the heat supplied (in joules) by the heater. The force constant of the spring is 8000N/m, atmospheric pressure is 1.0×105Nm−2. The cylinder and the piston are thermally insulated. The piston is massless and there is no friction between the piston and the cylinder. Neglect heat loss through lead wires of the heater. The heat capacity of the heater coil is negligible. Assume the spring to be massless.
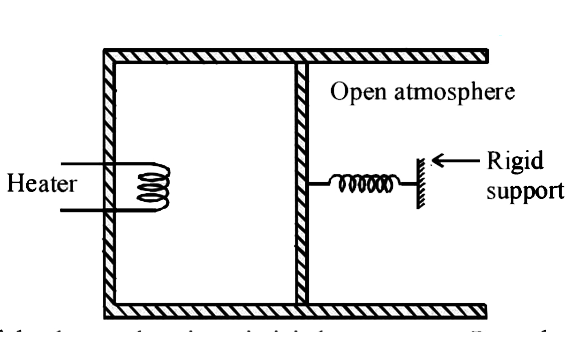
View SolutionAn ideal monatonic gas is confined in a cylinder by a spring-loaded piston of cross section 8.0×10−3m2.initially the gas is at 300K and occupies a volume of 2.4×10−3m−3 and the sprinng is in its relaxed state. The gas is heated by a small heater until the piston moves out slowly by 0.1m. Calculate the final tenoerature of the gas. The force constant if the spring is 8000Nm−1,and the atmospheric pressure is 1.0×105Nm−2.Htecyl∈derandtheπs→narethermally∈sylated.Theπs→nandthespr∈garemass≤ssandthereisnoictionbetweentheπs→nandthecyl∈der.Neg≤ctanyheat−lossthroughthe≤adwiresoftheheater.Theheat∩acityoftheheatercoilis¬ligib≤.

View Solution
Knowledge Check
One mole of an ideal monotomic gas undergoes a linear process from A to B in which its pressure p and its volume V change as shown in figure.
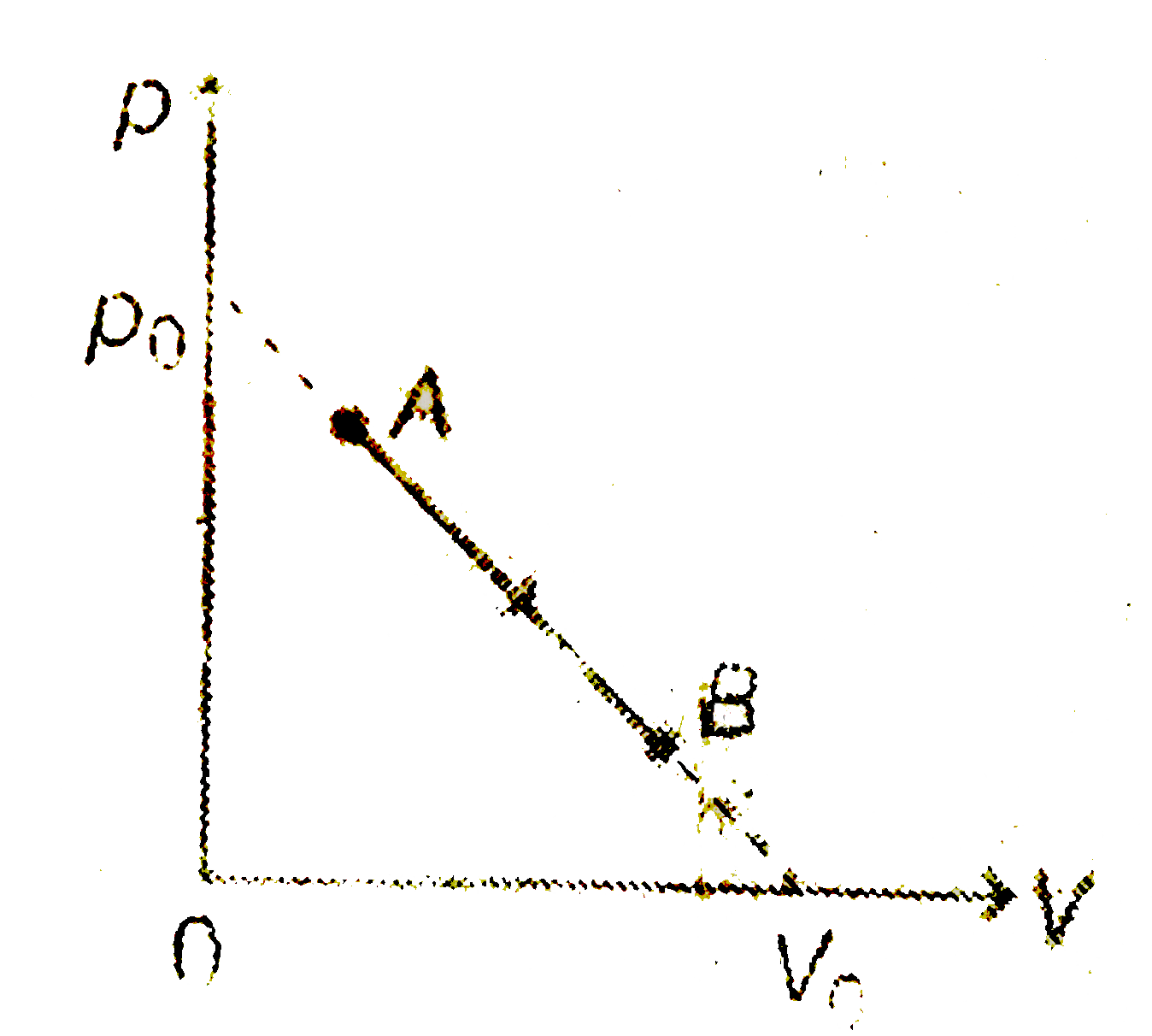
The absolute temperature T versus volume V for the given process isA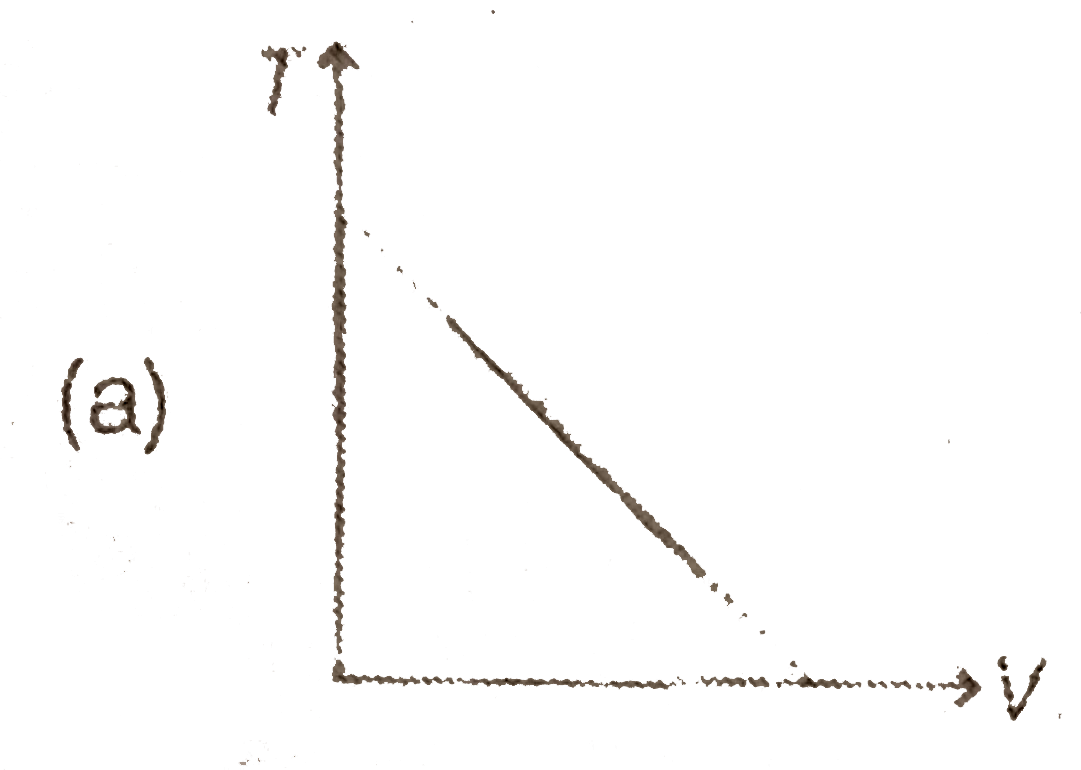 B
B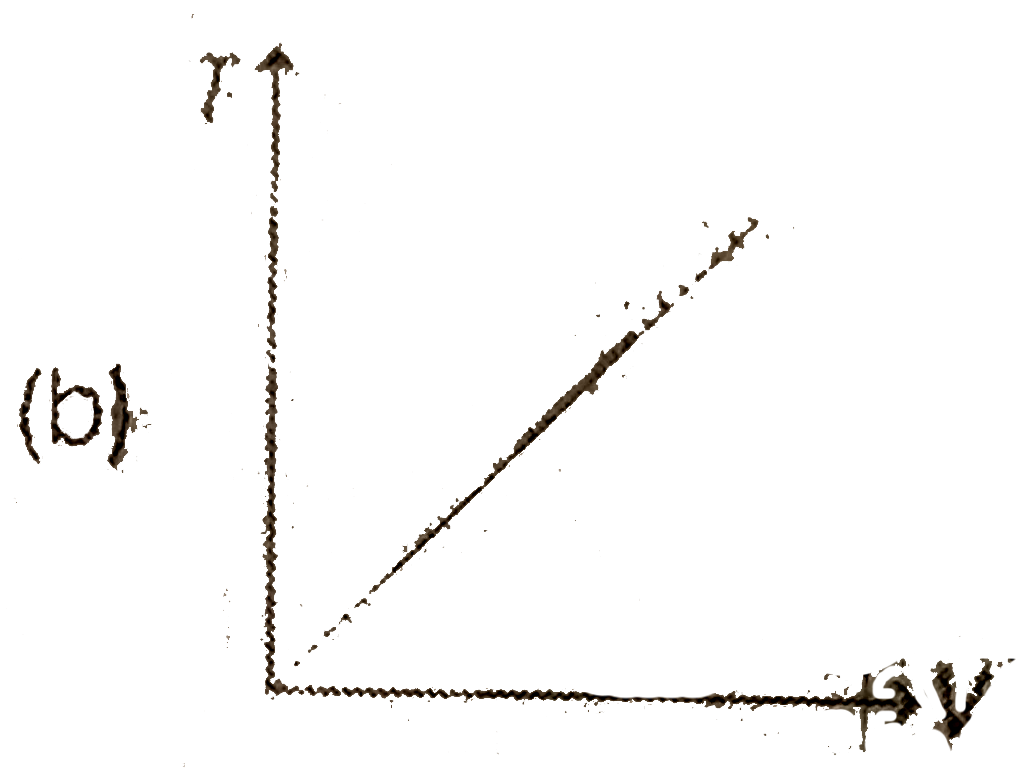 C
C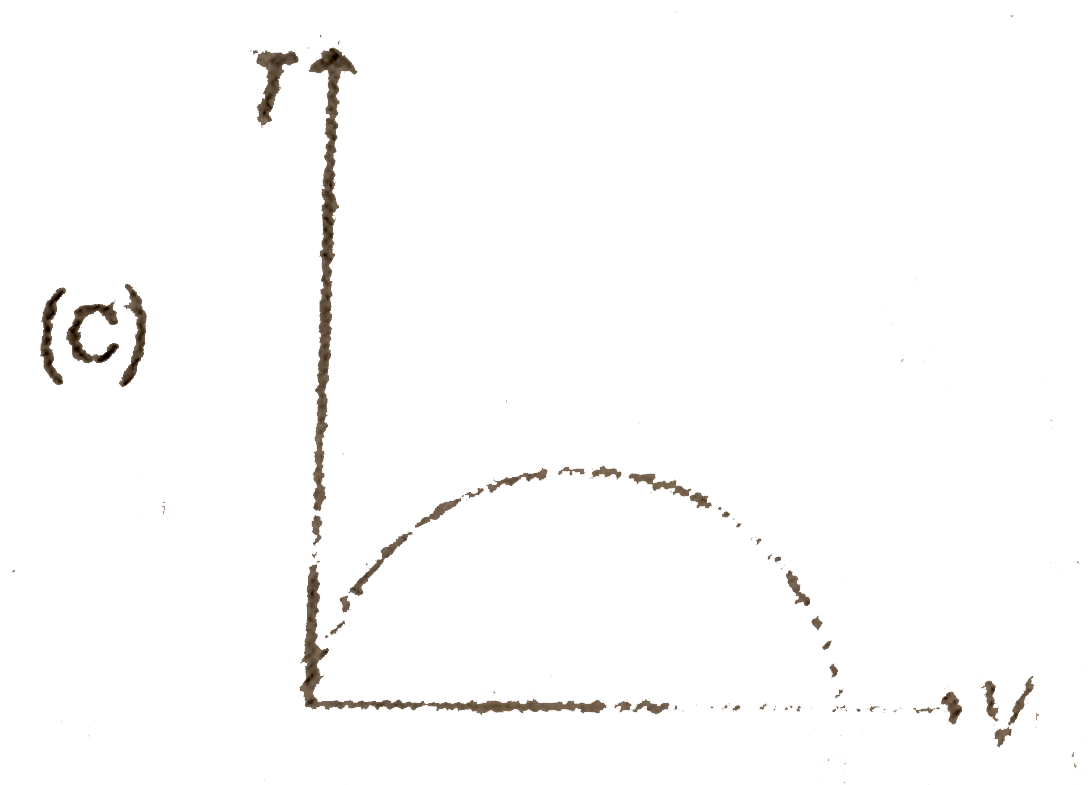 D
DPV Diagram for ideal gas in piston cylinder assembly undergoing a thermodynamic process is shown in figure. Process is
 AIsothermalBBasicCIsobaricDAdiabatic
AIsothermalBBasicCIsobaricDAdiabaticDuring an experiment, an ideal gas is found to obey a condition Vp2= constant. The gas is initially at a temperature (T), pressure (p) and volume (V). The gas expands to volume (4V).
AThe pressure of gas changes to p2BThe temperature of the gas changes to 4TCThe graph of the above process on (p - T) diagram is parabola.DThe graph of the above process on (p - T) diagram is hyperbola.
Two moles of an ideal monoatomic gas are confined within a cylinder by a massless spring loaded with a frictionless piston of negligible mass and of cross-sectional area 4×10−3m2. The springis initially in its relaxed state. Now the gas is heated by a heater for some time. During this time the gas expands and does 50 J of work in moving the piston through a distance of 0.1 m. The temperature of the gas increases by 50 K. Calculate the spring constant and the heat supplied by the heater.
View SolutionA monoatomic gas is enclosed in a nonconducting cylinder having a piston which can move freely. Suddenly gas is compressed to 1/8 of its initial volume. Find the final pressure and temperature if initial pressure and temperature are P0 and T0 respectively.

View SolutionTwo moles of an ideal monoatomic gas are confined within a cylinder by a massless and frictionless spring loaded piston of cross-sectional area 4×10−3m2. The spring is, initially in its relaxed state. Now the gas is heated by an electric heater, placed inside the cylinder, for some time. During this time, the gas expands and does 50J of work in moving the piston through a distance 0.10m. The temperature of the gas increases by 50K. Calculate the spring constant and the heat supplied by the heater. Patm=1×105N/m2R=8.314J/mol−K
View SolutionOne mole of an ideal gas is contained with in a cylinder by a frictionless piston and is initially at temperature T. The pressure of the gas is kept constant while it is heated and its volume doubles. If R is molar gas constant, the work done by the gas in increasing its volume is
View SolutionOne mole of an ideal monatomic gas undergoes a linear process from A to B , in which is pressure P and its volume V change as shown in figure .
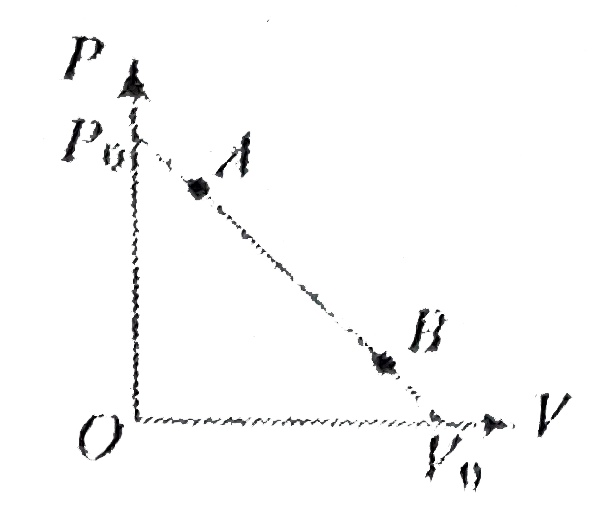
The absolute temperature T versus volume V for the given process is
View Solution
