Two plates eachb of area A, thickness L1andL2 thermal conductivities K1andK2 respectively are joined to form a single plate of thickness (L1+L2). If the temperatures of the free surfaces are T1andT2. Calculate.
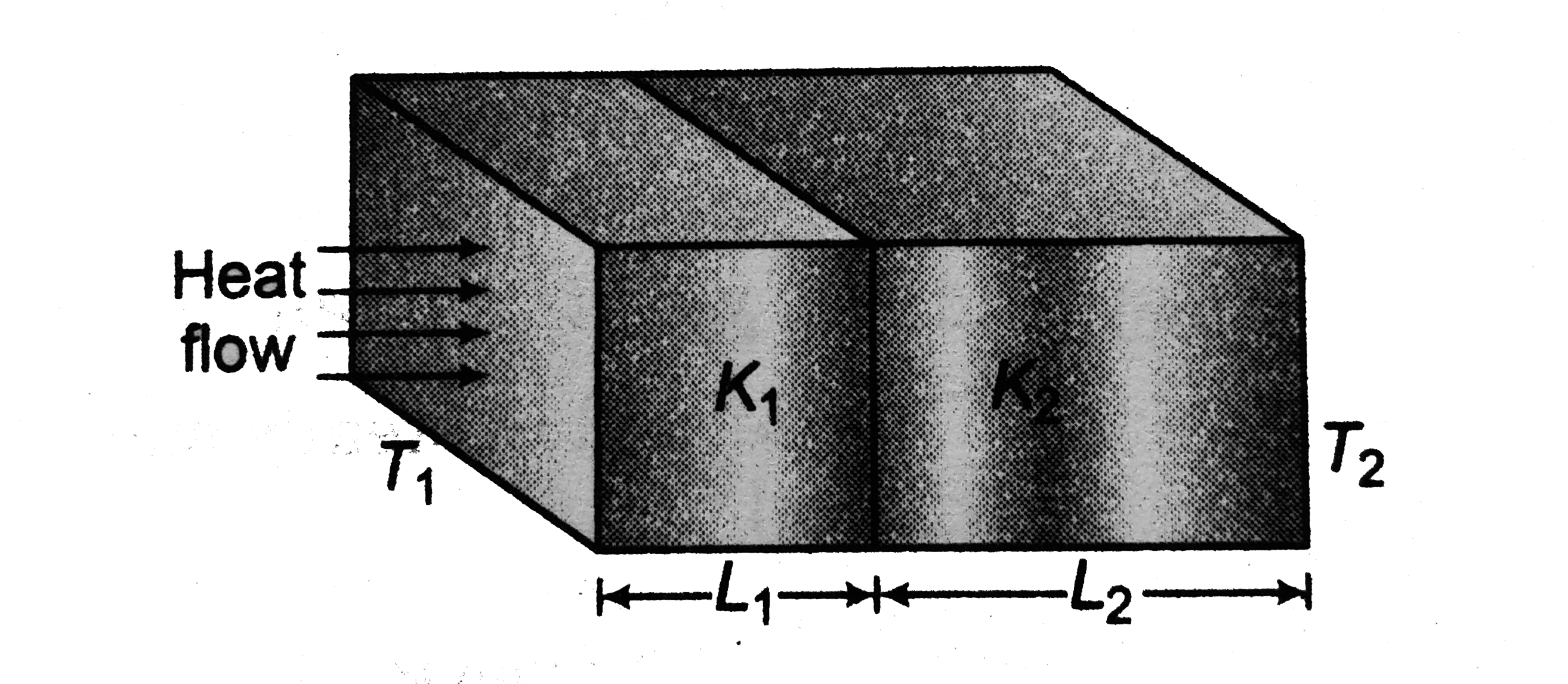
(a) rate of flow of heat
(b) temperature of interface and
(c) equivalent thermal conductivity.
The correct Answer is:A, B
(a) If the thermal resistance of the two plates are R1andR2 respectively. Plates are
in series.
Rs=R1+R2=(L1AK1)+(L2AK2)
as R=LKA
and so H=dQdt=ΔTR
=(T1−T2R1+R2)=A(T1−T2)(L1K1)+(L2K2)
(b) If T is the common temperature of interface, then as in series rate of flow of heat remains
same, i.e. H=H1(=H2)
(T1−T2R1+R2)=T1−TR1
i.e. T=(T1R2+T2R2R1+R2)
or T=[T1L2K2]+T2[L1K1][L1K1+L2K2](asR=LKA)
(c) If K is the equivalent conductivity of composite slab, i.e. slab of thickness L1+L2 and cross - sectional area A, then as in series.
RS=R1+R2orL1+L2AKeq=R1+R2
i.e. Keq=L1+L2A(R1+R2)=L1+L2[L1K1+L2K2] (as R=LKA ).
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - CALORIMETRY & HEAT TRANSFEREXERCISE - Exercise 22.17videos
CALORIMETRY & HEAT TRANSFER
- BOOK - DC PANDEYCHAPTER - CALORIMETRY & HEAT TRANSFEREXERCISE - Exercise 22.27videos
CALORIMETRY & HEAT TRANSFER
- BOOK - DC PANDEYCHAPTER - CALORIMETRY & HEAT TRANSFEREXERCISE - Example Type 44videos
CALORIMETRY & HEAT TRANSFER
- BOOK - DC PANDEYCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise13videos
BASIC MATHEMATICS
- BOOK - DC PANDEYCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery38videos
CALORIMETRY AND HEAT TRANSFER
Similar Questions
Two plates each of area A thickness L1 and L2 thermal conductivities K1 and K2 respectively are joined to from a single plate of thickness (L1+L2) If the temperatures of the free surfaces are θ1 and θ2 Calculate
(a) Rate of flow of heat
(b) Temperature of interface
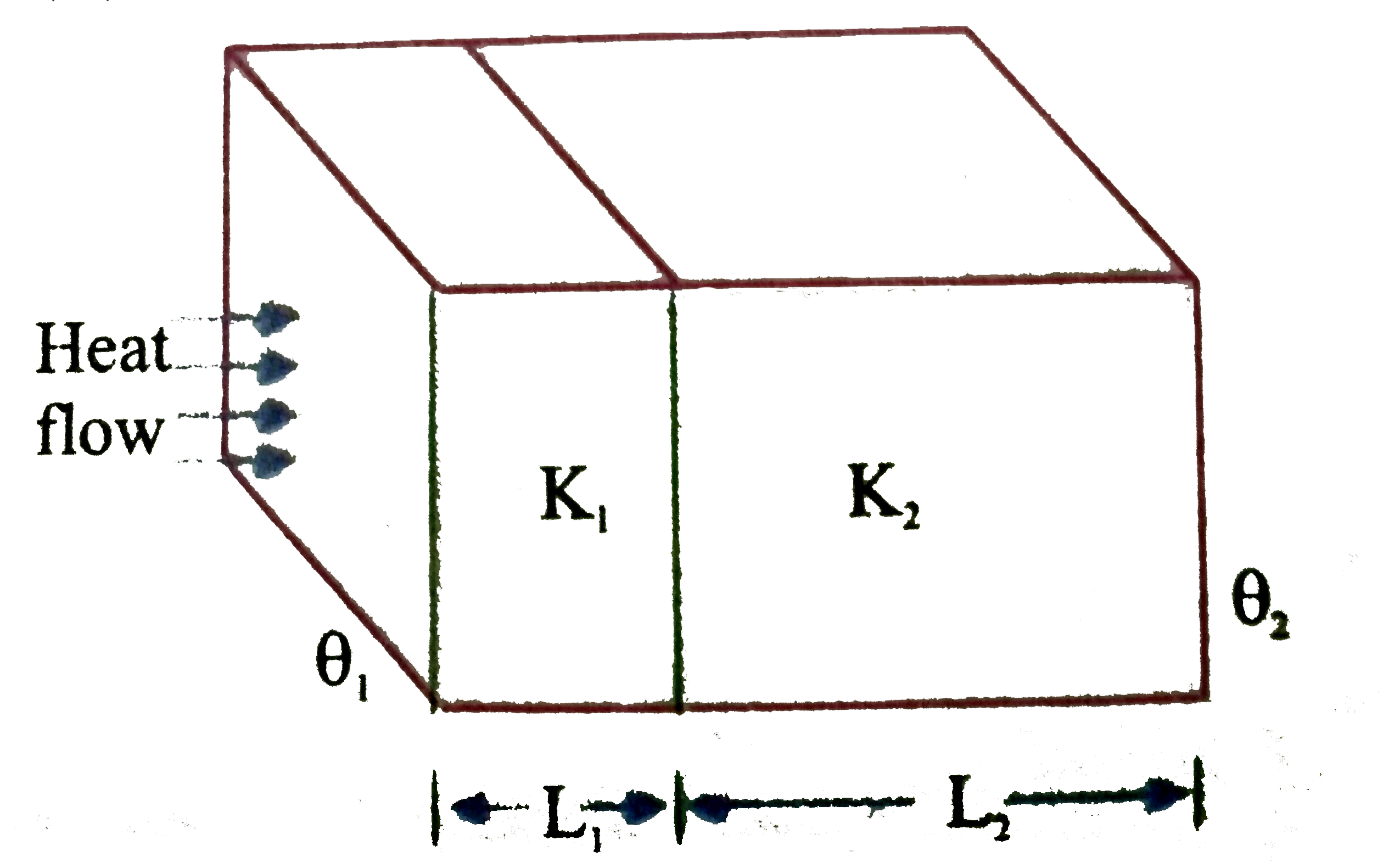 .View Solution
.View SolutionTwo walls of thicknes l1 and l2 and ther-mal condctivities K1 and K2 are in contact In the steady state, if the temperature at the outer faces are T1 and T2 find the temperature at the common wall .
View Solution
Knowledge Check
- Question 1 - Select One
If two conducting slabs of thickness d1 and d2, and thermal conductivity K1 and K2 are placed together face to face as shown in figure in the steady state temperature of outer surfaces are θ1 and θ2. The temperature of common surface is-
 AK1θ1d1+K2θ2d2K1d1+K2d2BK1θ1+K2θ2K1+K2CK1θ1+K2θ2θ1+θ2DK1θ1d2+K2θ2d1K1d2+K2d1
AK1θ1d1+K2θ2d2K1d1+K2d2BK1θ1+K2θ2K1+K2CK1θ1+K2θ2θ1+θ2DK1θ1d2+K2θ2d1K1d2+K2d1 - Question 2 - Select One
Two walls of thickness d and d and thermal conductivities k and k are in contact. In the steady state, if the temperature at the outer T1 and T2 , the temperature at the common wall is
Ak1T1d2+k2T2d1k1d2+k2d1Bk1T1+k2d2d1+d2C(k1d1+k2d2T1+T2)T1T2Dk1d1T1+k2d2T2k1d1+k2d2 - Question 3 - Select One
If two conducting slabs of thickness d1 and d2, and thermal conductivity K1 and K2 are placed together face to face as shown in figure in the steady state temperature of outer surfaces are θ1 and θ2. The temperature of common surface is-
 AK1θ1d1+K2θ2d2K1d1+K2d2BK1θ1+K2θ2K1+K2CK1θ1+K2θ2θ1+θ2DK1θ1d2+K2θ2d1K1d2+K2d1
AK1θ1d1+K2θ2d2K1d1+K2d2BK1θ1+K2θ2K1+K2CK1θ1+K2θ2θ1+θ2DK1θ1d2+K2θ2d1K1d2+K2d1
Two materials having coefficients of thermal conductivity ‘3 K’ and ‘K’ and thickness ‘d’ and ‘3 d’, respectively, are joined to form a slab as shown in the figure. The temperatures of the outer surfaces are θ2 and θ1 respectively, (θ2>θ1) The temperature at the interface is:
 View Solution
View SolutionIf two metallic plates of equal thickness and thermal conductivities K1 and K2 are put together face to face and a common plate is constructed, then the equivalent thermal conductivity of this plate will be
 View Solution
View SolutionIf layer thickness L2 is 1.4cm, then its thermal conductivity K2 will have value (in W/mK)
View SolutionIf two metallic plates of equal thickness and equal length and thermal conductivities K1andK2 are put together face to face a common plate is constructed, then the equivalent thermal conductivity of this plate will be-
View SolutionTwo identical plates with thermal conductivities K and 3K are joined together to form a single plate of double thickness. The equivalent thermal conductivity of one composite plate so formed for the flow of heat through its thickness is.
View Solution

