Draw E−r and V−r graphs due to two point charges +q and −2q kept at some along the line joining these two charges.
To draw the E−r (Electric Field vs. Distance) and V−r (Electric Potential vs. Distance) graphs due to two point charges +q and −2q, we will follow these steps:
Step 1: Understand the Setup
We have two point charges:
- Charge +q located at point A.
- Charge −2q located at point B.
We will analyze the electric field and potential along the line joining these two charges.
Step 2: Define the Regions
We can divide the space into three regions based on the positions of the charges:
1. Region 1: To the left of charge +q (from −∞ to the position of +q).
2. Region 2: Between the charges +q and −2q.
3. Region 3: To the right of charge −2q (from the position of −2q to +∞).
Step 3: Electric Field Calculation
The electric field E due to a point charge is given by the formula:
\( E = \frac{k \cdot q}{r^2} \)
where k is Coulomb's constant and r is the distance from the charge.
- In Region 1 (left of +q):
- The electric field due to +q is directed away from it (positive direction).
- The electric field due to −2q is directed towards it (negative direction).
- As we move away from +q, the electric field decreases and approaches zero as r approaches −∞.
- In Region 2 (between the charges):
- The electric field due to +q is still directed away from it.
- The electric field due to −2q is directed towards it.
- The net electric field will depend on the relative distances to each charge. At some point, the electric field will be zero (let's call this point P).
- In Region 3 (right of −2q):
- The electric field due to −2q dominates and is directed towards it.
- As we move away from −2q, the electric field decreases and approaches zero as r approaches +∞.
Step 4: Electric Potential Calculation
The electric potential V due to a point charge is given by:
\( V = \frac{k \cdot q}{r} \)
- In Region 1:
- As r approaches 0 (close to +q), V approaches +∞.
- As r approaches −∞, V approaches 0.
- In Region 2:
- At point P (where the electric field is zero), the potential will be a finite negative value due to the influence of −2q.
- As we approach −2q, V approaches −∞.
- In Region 3:
- As r approaches 0 (close to −2q), V approaches −∞.
- As r approaches +∞, V approaches 0.
Step 5: Draw the Graphs
- E-r Graph:
- Start from 0 at −∞, rise to a maximum positive value near +q, drop to zero at point P, and then drop to a negative value as we approach −2q, finally approaching zero again at +∞.
- V-r Graph:
- Start from 0 at −∞, rise to +∞ near +q, drop to a negative value at point P, and then drop to −∞ as we approach −2q, finally approaching 0 again at +∞.
Final Graphs
- The E−r graph will show a curve that starts from zero, peaks, and then goes negative.
- The V−r graph will start from zero, rise to positive infinity, drop to negative infinity, and return to zero.
We have two point charges:
- Charge +q located at point A.
- Charge −2q located at point B.
We can divide the space into three regions based on the positions of the charges:
1. Region 1: To the left of charge +q (from −∞ to the position of +q).
2. Region 2: Between the charges +q and −2q.
3. Region 3: To the right of charge −2q (from the position of −2q to +∞).
The electric field E due to a point charge is given by the formula:
\( E = \frac{k \cdot q}{r^2} \)
where k is Coulomb's constant and r is the distance from the charge.
- The electric field due to +q is directed away from it (positive direction).
- The electric field due to −2q is directed towards it (negative direction).
- As we move away from +q, the electric field decreases and approaches zero as r approaches −∞.
- The electric field due to +q is still directed away from it.
- The electric field due to −2q is directed towards it.
- The net electric field will depend on the relative distances to each charge. At some point, the electric field will be zero (let's call this point P).
- The electric field due to −2q dominates and is directed towards it.
- As we move away from −2q, the electric field decreases and approaches zero as r approaches +∞.
The electric potential V due to a point charge is given by:
\( V = \frac{k \cdot q}{r} \)
- In Region 1:
- As r approaches 0 (close to +q), V approaches +∞.
- As r approaches −∞, V approaches 0.
- At point P (where the electric field is zero), the potential will be a finite negative value due to the influence of −2q.
- As we approach −2q, V approaches −∞.
- As r approaches 0 (close to −2q), V approaches −∞.
- As r approaches +∞, V approaches 0.
- E-r Graph:
- Start from 0 at −∞, rise to a maximum positive value near +q, drop to zero at point P, and then drop to a negative value as we approach −2q, finally approaching zero again at +∞.
- Start from 0 at −∞, rise to +∞ near +q, drop to a negative value at point P, and then drop to −∞ as we approach −2q, finally approaching 0 again at +∞.
- The E−r graph will show a curve that starts from zero, peaks, and then goes negative.
- The V−r graph will start from zero, rise to positive infinity, drop to negative infinity, and return to zero.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.14videos
ELECTROSTATICS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.29videos
ELECTROSTATICS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Example Type 83videos
ELECTROSTATICS
- BOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises50videos
ELECTROSTATIC POTENTIAL AND CAPACITORS
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions120videos
GRAVITATION
Similar Questions
Knowledge Check
- Question 1 - Select One
Two identical charges +Q are kept fixed some distance apart.A small particles P with charge q is placed midway between them . If P is given a small displacement Δ ,it will undergo simple harmonic motion if
(i) q is positive and Δ is along the line joining the charges
(ii) q is positive and Δ is perpendicular to the line joining the charges
(iii) q is negative and Δ is perpendicular to the line joining the charges
(iv) q is positive and Δ is along the line joining the chargesA(i),(ii)B(i),(iii)C(ii),(iii)D(i),(iv) - Question 2 - Select One
Two charge +q and −q are kept apart.then at any point on the right beisector of line joining the two charges
Athe electric fields strenght is zeroBthe elctric potential is zeroCboth electric potential and electric field strength are zeroDboth electric potential and electric field strength are non zero - Question 3 - Select One
Two point charges +9e and +e are kept at a distance 'a' from each other. A third charge is placed at a distance 'x' from +9e on the line joining the above two charges. For the third charge to be in equilibrium 'x' is
A3a/4B3a/2Ca/2Da/4
Two charge +aq and -q are kept apart. Then , at any point on the right bisector of line joining the two charge
View SolutionTwo idential point charges Q are kept at a distance r from each other. A third point charge is placed on the line joing the above two charges such that all the three charges are in equilibrium. The third charge
(a) should be of magnitude q=………..
(b) should be of sign .......
(c ) should be placed ........ .View SolutionTwo point charges+ q and-q are placed at distanced apart. What are the points at which the resultant field is parallel to the line joining the two charges ?
View SolutionTwo point charges +4q and +q are placed 30cm apart. At what point on the line joining them is the electric field zero
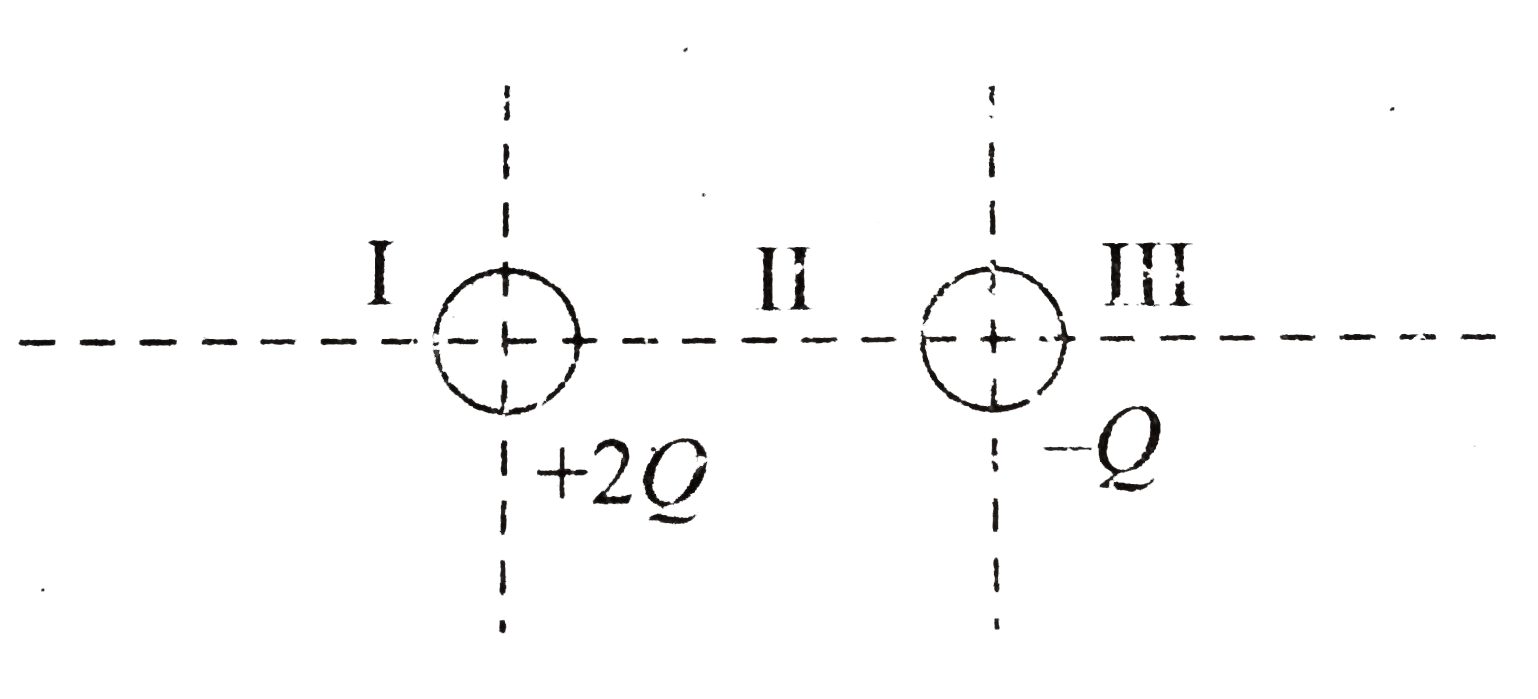
View SolutionThe figure shows two point charges 2Q(gt0) and -Q. The charges divide the line joining them in three parts I, II, and III.
 View Solution
View Solution


