A double slit experiment is performed with light of wavelength 500nm. A thin film of thickness 2μm and refractive index 1.5 is introduced in the path of the upper beam. The location of the central maximum will
Remain unshifted
Shift downward by nearly two fringes
Shift upward by nearly two fringes
Shift downward by 10 fringes.
The correct Answer is:C
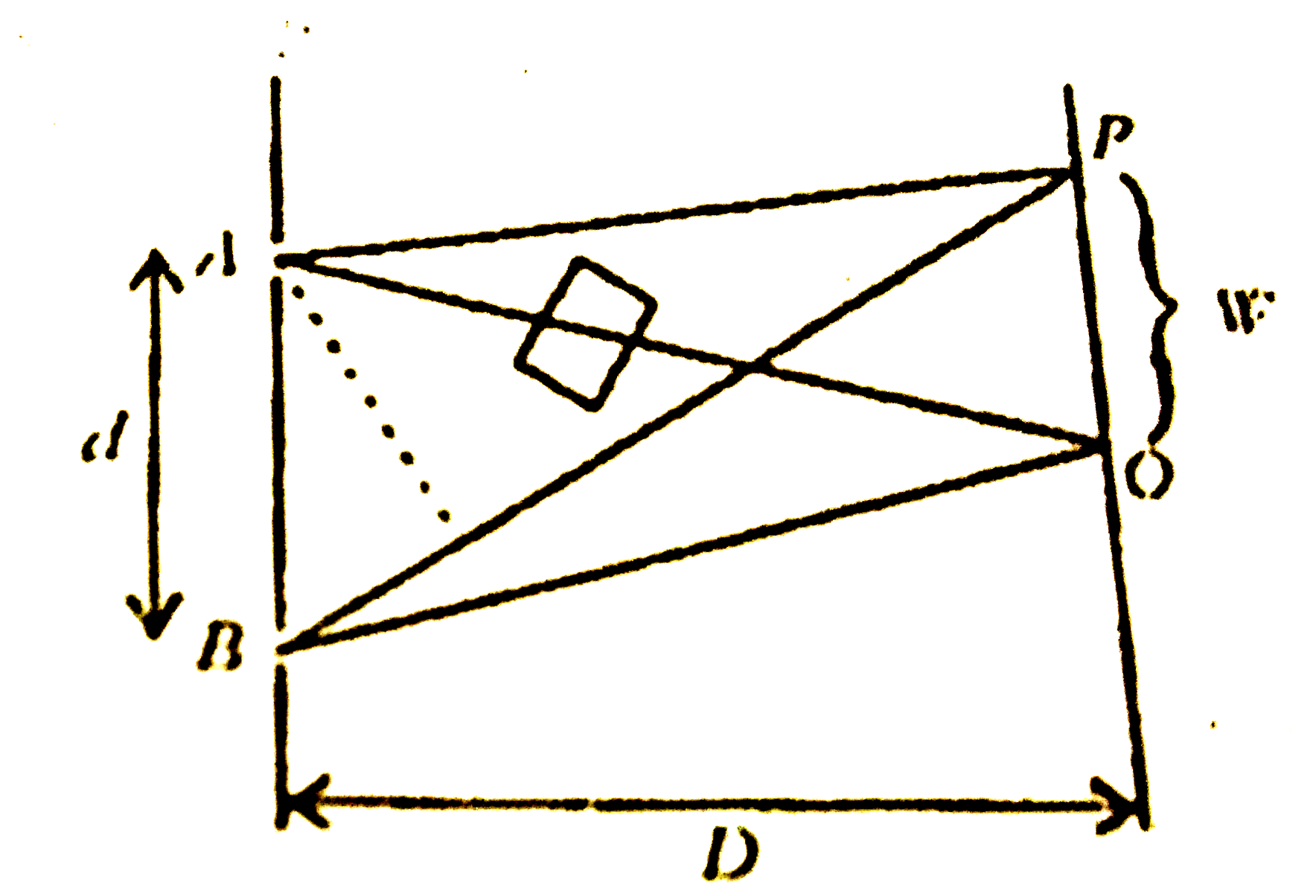
Wavelength λ=500nm=500×10−9m
Thickness of the film, t= 2 μm=2×10−6m
Refractive index μ=1.5
When there is no thin placed in the path of any one of the two beams, the path difference between them is given by dy/D ( considering the two beams meetings at a point P). In case we put a thin film in the path of one of the beams , then the optical path of that beam gets longer. Now for the central maximum, path difference in absence of the film is Δx=0. But when we put the film , the path difference becomes
Δ=μt−t=(μ−1)t
=(1.5−1)×2×10−6=10−6m=1mm
Now, Δx=dvD⇒y=DdΔx=Ddxx1μm
and the fringe width is given by
W=Dλd=Dd×500×10−9m=Ddxx0.5×1μm
∴y=Ddxx1μm=2×Ddxx12×1μm=2W
As the film is placed in the path of the upper beam, the central maximum will shift upward by nearly two fringes.
Topper's Solved these Questions
Similar Questions
In Young's double slit experiment the two slits are illuminated with light of wavelength λ .The thickness of a film of refractive index 1.4 to be introduced in the path of one of the interfering beams so that the central image shifts by one fringe width is
View SolutionIn YDSE, the central bright fringe produced by light of wavelength 5600Å shifts to the position of 5th bright fringe when a thin transparent film of refractive index 1.28 is introduced in the path of light from one of the two slits. Find the thickness of the film.
View Solution
Knowledge Check
- Question 1 - Select One
A double slit experiment is performed with light of wavelength 500 nm A thin film of thickness 2 μm and refractive index 1.5 is introduced in the path of the upper beam the location of the central maximum will
Aremain unshiftedBshift downward by nearly two fringesCshift upward by nearly two fringesDshift downward by its fringes - Question 2 - Select One
A double slit experiment is performed with light of wavelength 500 nm A thin film of thickness 2 μm and refractive index 1.5 is introduced in the path of the upper beam the location of the central maximum will
Aremain unshiftedBshift downward by nearly two fringesCshift upward by nearly two fringesDshift downward by its fringes - Question 3 - Select One
A thin mica sheet of thickness 2×10−6m and refractive index (μ=1.5) is introduced in the path of the first wave. The wavelength of the wave used is 5000Å. The central bright maximum will shift
A2 fringes upwardB2 fringes downwardC10 fringes upwardDNone of these
A thin mica sheet of thickness 4×10−6 m and refractive index (μ=1.5) is introduced in the path of the light from upper slit. The wavelength of the wave used is 5000Å The central bright maximum will shift
View SolutionYoung's double slit experiment is performed with monochromatic light. A thin film is introduced in front of one of the slits
View SolutionIn Young's double-slit experiment, let A and B be the two slit. A thin film of thickness t and refractive index μ is placed in front of A. Let β= fringe width. Then the central maxima will shift
View SolutionIn a young's double slit experiment ,let Aand B be the two slits.A thin film film of thickness t and refractive indexμ is pklaced in front of A.Let β =fringe width.The central maximum will shift:
View SolutionThe central fringe shifts to the position of fifth bright fringe, if a thin film of refractive index 1.5 is introduced in the path of light of wavelength 5000Å. The thickness of the glass plate is
View Solution

