OABC is a tetrahedron D and E are the mid points of the edges −−→OA and −−→BC. Then the vector −−→DE in terms of −−→OA,−−→OBand−−→OC
Step by step video & image solution for OABC is a tetrahedron D and E are the mid points of the edges vec (OA) and vec (BC). Then the vector vec(DE) in terms of vec(OA), vec (OB) and vec(OC) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
.Knowledge Check
- Question 1 - Select One
The resultant of the three vectors −−→OA,−−→OB and −−→OC shown in figure is
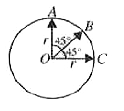ArB2rCr(1+√s)Dr(√2−1) - Question 2 - Select One or More
If OAB is a tetrahedron with edges and ˆp,ˆq,ˆr are unit vectors along bisectors of
−−→OA,−−→OB:−−→OB,−−→OC:−−→OC,−−→OA respectively and ˆa=−−→OA∣∣∣−−→OA∣∣∣,→b=−−→OB∣∣∣−−→OB∣∣∣,→c=−−→OC∣∣∣−−→OC∣∣∣, then :A[ˆaˆbˆc][ˆpˆqˆr]=3√32B[ˆa+ˆb ˆb+ˆc ˆc+ˆa][ˆp+ˆq ˆq+ˆr ˆr+ˆp]=3√34C[ˆa+ˆb ˆb+ˆc ˆc+ˆa][ˆpˆqˆr]=3√32D[ˆaˆbˆc][ˆp+ˆq ˆq+ˆr ˆr+ˆp]=3√34
If P, Q , R are the mid-points of the sides AB, BC and CA of ΔABC and O is point whithin the triangle, then −−→OA+−−→OB+−−→OC=
View SolutionProve that 3−−→OD+−−→DA+−−→DB+−−→DC is equal to −−→OA+−−→OB+−−→OC.
View SolutionThe resultant of vectors −−→OAand−−→OB is peerpendicular to vec(OA). Find the angle AOB.
View SolutionThe resultant of vectors −−→OAand−−→OB is perpendicular to −−→OA. Find the angle AOB.
View SolutionThe resultant of vectors −−→OAand−−→OB is peerpendicular to −−→OA. Find the angle AOB.
View SolutionIf OAB is a tetrahedron with edges and ˆp,ˆq,ˆr are unit vectors along bisectors of
−−→OA,−−→OB:−−→OB,−−→OC:−−→OC,−−→OA respectively and ˆa=−−→OA∣∣∣−−→OA∣∣∣,→b=−−→OB∣∣∣−−→OB∣∣∣,→c=−−→OC∣∣∣−−→OC∣∣∣, then :View Solution