The resultant of vectors vec(OA) and vec(OB) is peerpendicular to vec(

The resultant of two vectors $\to A$ and $\to B$ is perpendicular to the vector $\to A$ and its magnitudes is equal to half of the magnitudes of vector $\to B$ (figure). The angle between $\to A$ and $\to B$ is

A $120^{\circ}$
B $150^{\circ}$
C $135^{\circ}$
DNone of these
The resultant of two vectors $\to A and \to B$ is perpendicular to the vector $\to A$ and its magnitude is equal to half the magnitude of vector $\to B$ . What is the angle between $\to A and \to B$ ?
A $30^{\circ}$
B $45^{\circ}$
C $150^{\circ}$
D $120^{\circ}$
The resultant of the three vectors $- - \to O A, - - \to O B$ and $- - \to O C$ shown in figure is

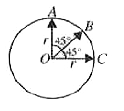Ar
B2r
C $r (1 + \sqrt{s})$
D $r (\sqrt{2} - 1)$

The resultant of vectors $- - \to O A and - - \to O B$ is peerpendicular to vec(OA). Find the angle AOB.