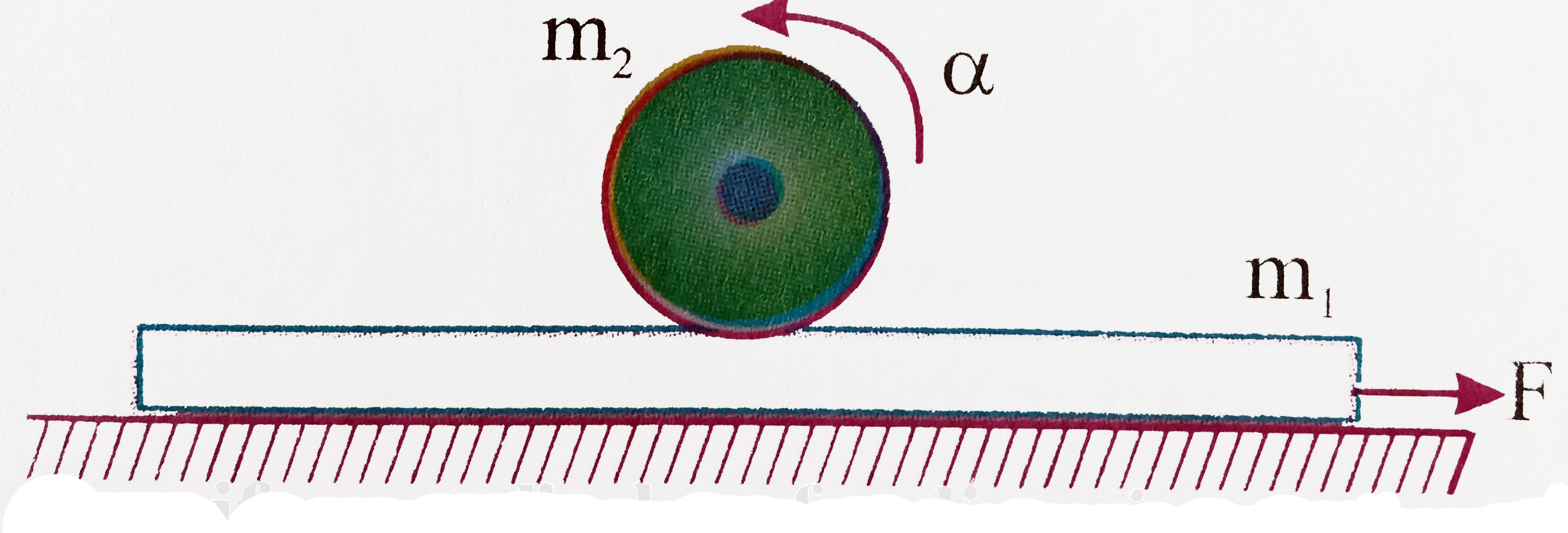
In the following figure, a sphere of radius 3m rolls on a plank. The accelerations of the sphere and the plank. The accelerations of the sphere and the plank are indicated. The value of α is

3rad/s2
6rad/s2
3rad/s2 (opposite to the direction shown in figure)
1rad/s2
The correct Answer is:A
a1=Rα−a2
3=3α−6
9=3α
α=3rad/s2
Topper's Solved these Questions
Similar Questions
Knowledge Check
In Fig a sphere of radius 2m rolls on a plank. The accelerations of the sphere and the plank are indicated. The value of α is
 A2rad/s2B4rad/s2C3rad/s2D1rad/s2
A2rad/s2B4rad/s2C3rad/s2D1rad/s2A solid sphere of mass m rolls without slipping on an inclined plane of inclination θ. The linear acceleration of the sphere is
A75gsinθB27gsinθC37gsinθD57gsinθA sphere rolls down on an inclied plane of inclination θ. What is the acceleration as the sphere reaches bottom ?
A57gsinθB35gsinθC27gsinθD25gsinθ
A sphere rolls down on an inclined plane of inclination q. What is the acceleration as the sphere reaches bottom?
View SolutionA plank of mass m1 with a uniform solid sphere of mass m2 placed on it rests and a force F is applied to the plank. The acceleration of the plank provided there is no sliding between the plank and the sphere is (Fm1+n7m2) then the value of n is
View SolutionA plank of mass M is placed on a rough horizontal force F is applied on it A man of mass m rens on the plank find the acceleration of the man so that the plank does not move on the surface . The coefficent of friction between the plank and the surface is μ Assume that the man does not slip on the plank

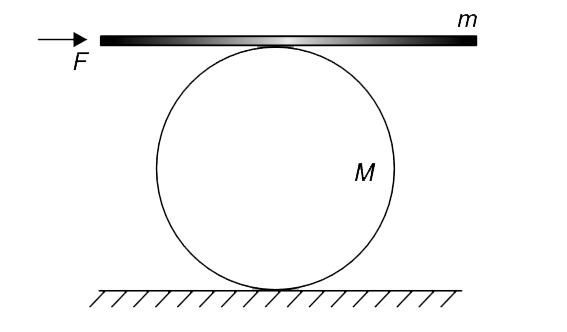
View SolutionA boy pushes a cylinder of mass M with the help of a plank of mass m as shown in figure. There is no slipping at any contact. The horizontal component of the force applied by the boy on the plank is F. Find
(a) The acceleration of the centre of the cylinder
(b) The friction force between the plank and the cylinder
View SolutionA sphere rolls down on an inclied plane of inclination θ. What is the acceleration as the sphere reaches bottom ?
View Solution