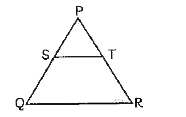
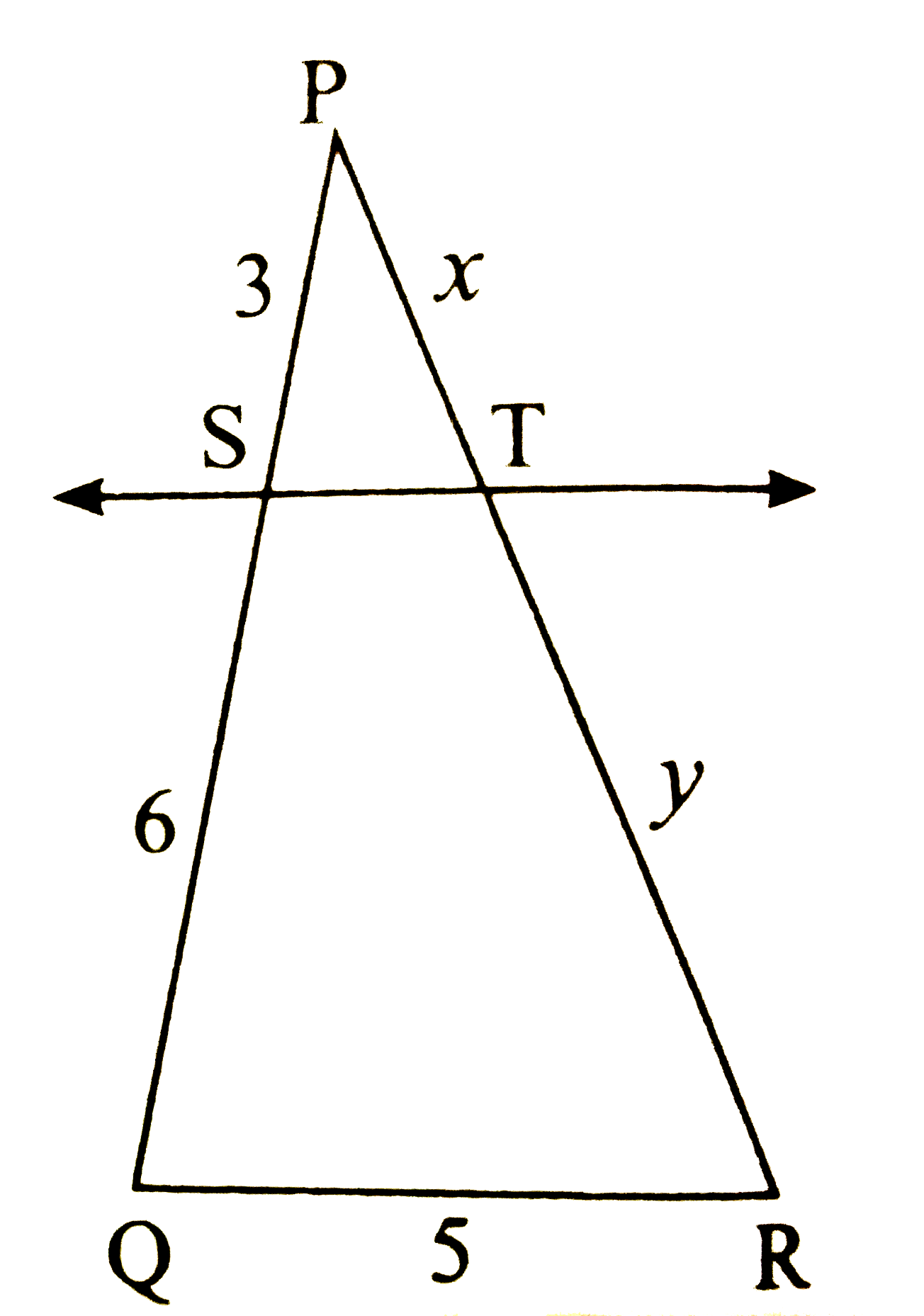
In the figure, PS=3, SQ=6, QR=5, PT=x and TR=y. Give any two pairs of values of x and y such that line ST∣∣ side QR.
Video Solution
Text Solution
In ΔPQR,
line ST∣∣ side QR ………..(Given)
∴ by basic proportionally theorem,
PSSQ=PTTR
∴36=xy
∴12=xy
∴y=2x
Consider x=2, then y=2×2=4
∴ When x=2 , then y=4 is one possibility
Consider x=2.5, then y=2×2.5=5
∴ When x=2.5, then y=5 is the other possibility
Note : while selecting the values of x and y the student needs to be careful as the sum of the lengths of two sides of a triangle is greater than lengths of the third side.
∴x= and y=2 cannot be possible though it satisfies the equation y=2x.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - 2.5 (4 mark each)3videos
SIMILARITY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - EXAMPLE TYPE50videos
SIMILARITY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - 2.3 (2 mark each)10videos
SIMILARITY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUADRATIC EQUATIONS EXERCISE - ASSIGNEMENT 2.48videos
QUADRATIC EQUATIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - STATISTICSEXERCISE - EXAMPLES FOR PRACTICE (MCQs)35videos
STATISTICS
Similar Questions
Knowledge Check
- Question 1 - Select One
S and U are two points on the side PQ of ΔPQR , such that QU=PS . If ST||QR and UV∣∣PR , then
ATV||PQBTV⊥PQCTV and PQ intersect at 60∘DTV and PQ intersect at 45∘ - Question 2 - Select One
In the figure, PQ = QR = RS = SP = SQ = 6 cm and PT = RT = 14 cm. The length of ST (in cm) is
 A4√10B(7√3−2)C10D11
A4√10B(7√3−2)C10D11 - Question 3 - Select One
In the following figure, ST || QR, point S divides PQ in the ratio 4:5. If ST = 1.6 cm, what is the length of QR?
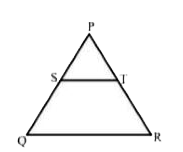 A0.71 cmB2 cmC3.6 cmD(cannot be calculated from the given data.)
A0.71 cmB2 cmC3.6 cmD(cannot be calculated from the given data.)
In the figure, RQ⊥PQ, PQ⊥PT and ST⊥PR. Prove that: ST × QR = PS × PQ
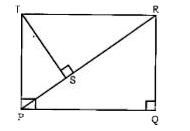 View Solution
View SolutionIn ΔPQR, if ST || QR, then what is the value of x?
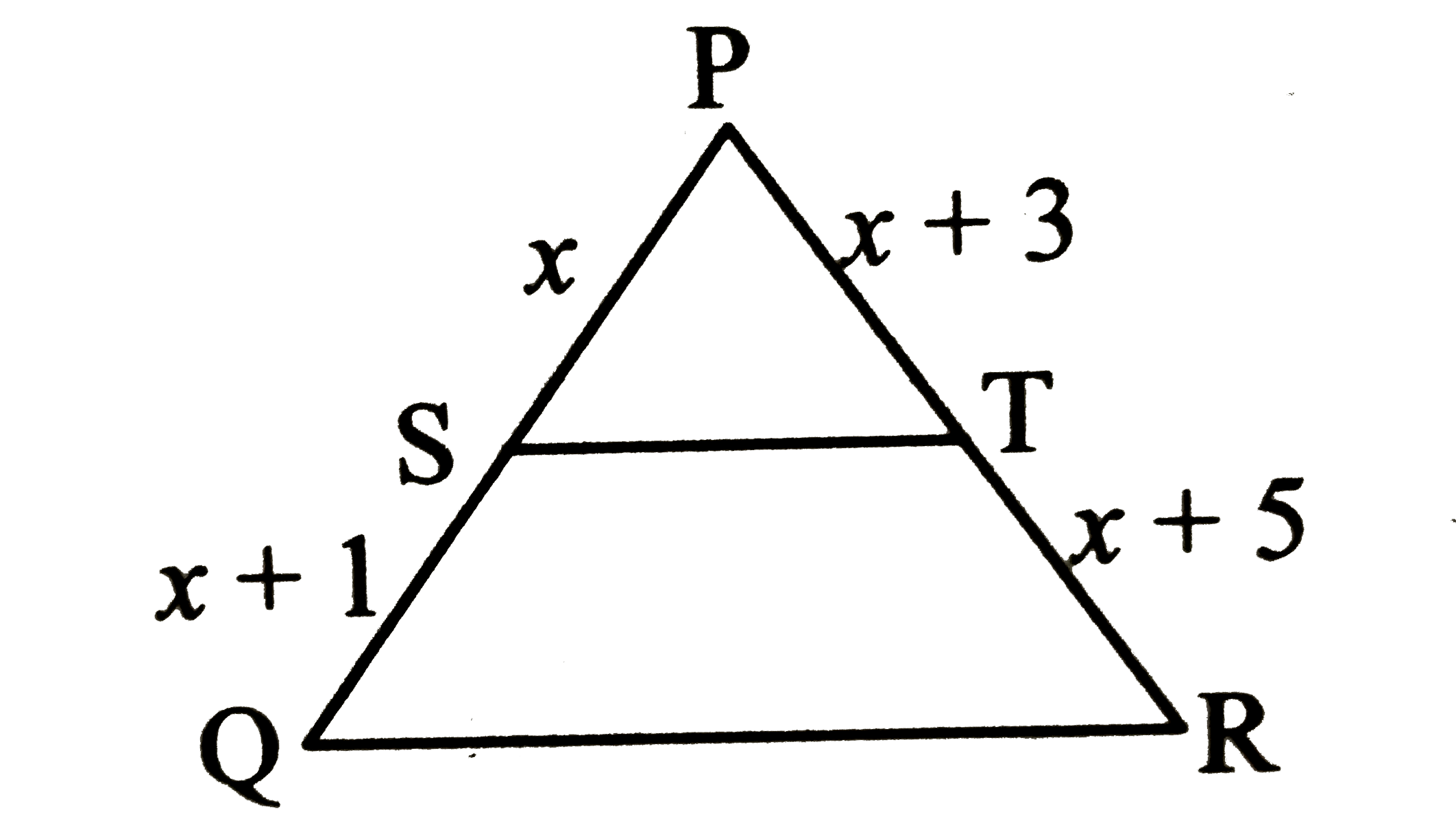 View Solution
View SolutionIn the adjoining figure, x:y:z=5:4:6. If XOY is a straight line, find the values of x, y and z.
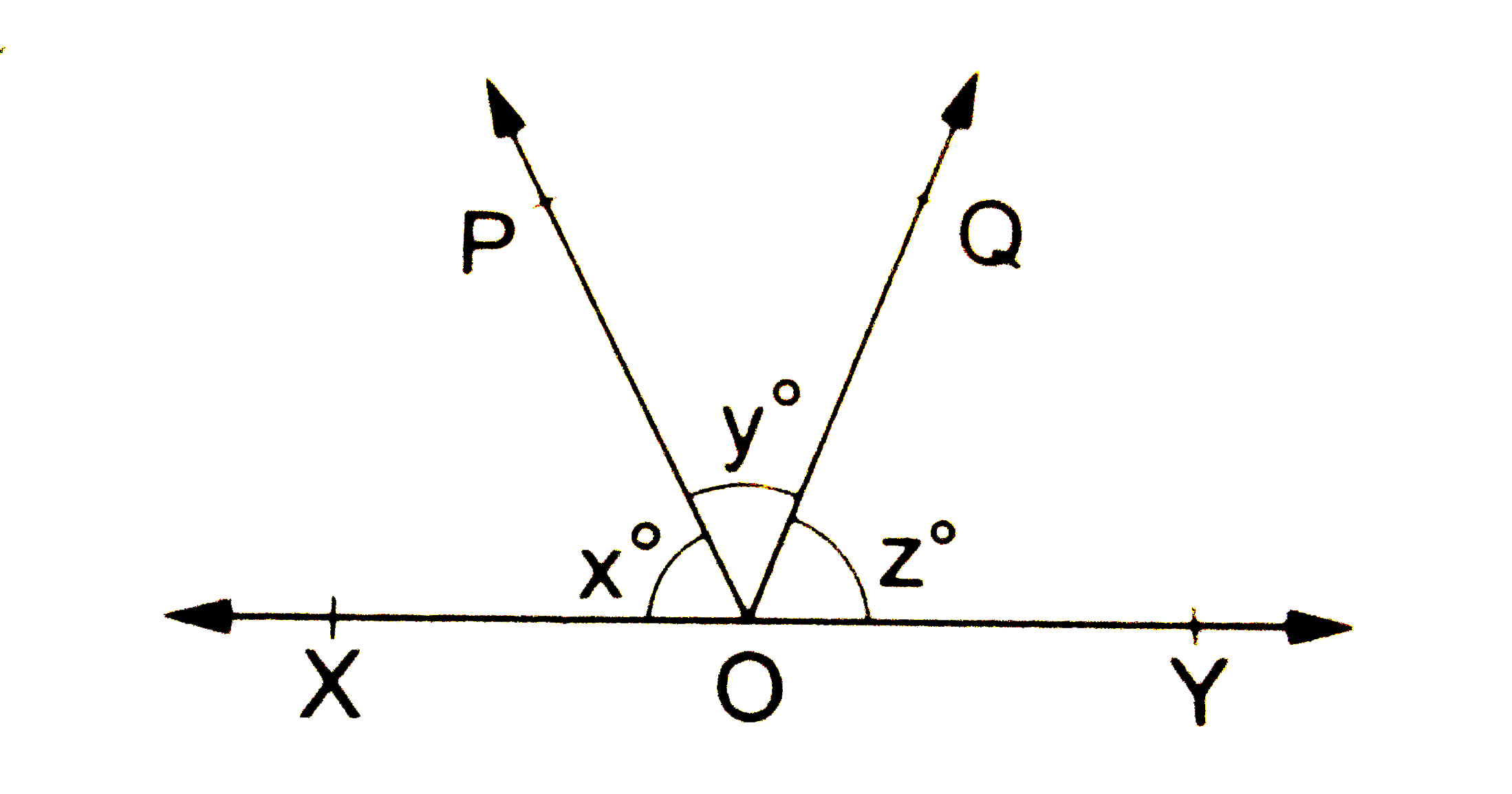 View Solution
View SolutionIn the given figure PR = QR = PS, ∠PSR=x∘,∠TPS=90∘ then x=?
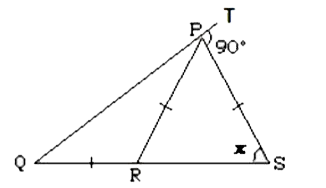 View Solution
View SolutionIn the figure □PQRS is a rectangle.
If Pq = 14 cm, QR = 21 cm , find the areas of the parts x,y and z.
 View Solution
View Solution