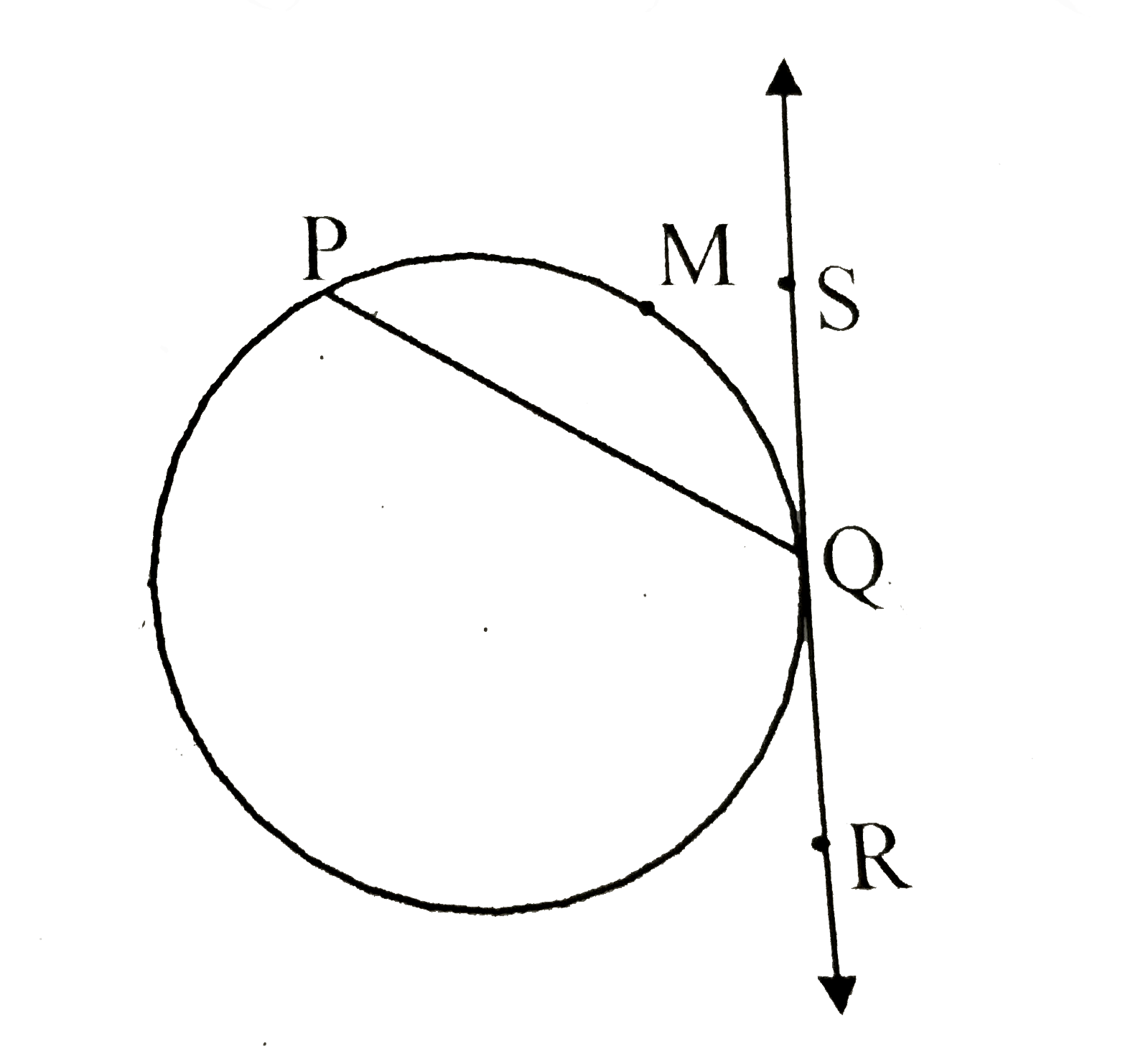
In the figure, m (arc LN ) =110∘, m ( ar PQ ) = 50∘ then complete the following activity to find ∠LMN
∠LMN=12[m(arcLN)=−□]
∴∠LMN=12[□−50∘]
∴_LMN=12×□
∴∠LMN=□
![In the figure, m (arc LN ) =110^(@), m ( ar PQ ) = 50^(@) then complete the following activity to find / LMN / LMN = (1)/(2) [ m ( arc LN ) =- square ] :. / LMN = (1)/(2) [ square - 50^(@) ] :. / LMN = (1)/(2) xx square :. / LMN = square](https://d10lpgp6xz60nq.cloudfront.net/physics_images/NVT_21_MAT_P2_X_C04_E04_009_Q01.png)
The correct Answer is:PQ,110∘,60∘,30∘
Step by step video & image solution for In the figure, m (arc LN ) =110^(@), m ( ar PQ ) = 50^(@) then complete the following activity to find /_ LMN /_ LMN = (1)/(2) [ m ( arc LN ) =- square ] :. /_ LMN = (1)/(2) [ square - 50^(@) ] :. / _ LMN = (1)/(2) xx square :. /_ LMN = square by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.4
12 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.5
8 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - ASSIGNMENT 4.2
6 Videos
- CHALLENGING QUESTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONSEXERCISE - SECTION 3 (MODEL QUESTION PAPER FOR PRACTICE ) Solve any one of the following subquestions :
1 Videos
- COORDINATE GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - COORDINATE GEOMETRY EXERCISE - Assignment 6.5
13 Videos
Similar Questions
Knowledge Check
In two triangles PQR and LMN, PQ = QR, ∠P=∠MandQR=LN, then which of the following is true?
ATriangles are congruent onlyBTriangles are isosceles onlyCTriangles are both congruent and isoscelesDcan't be determined
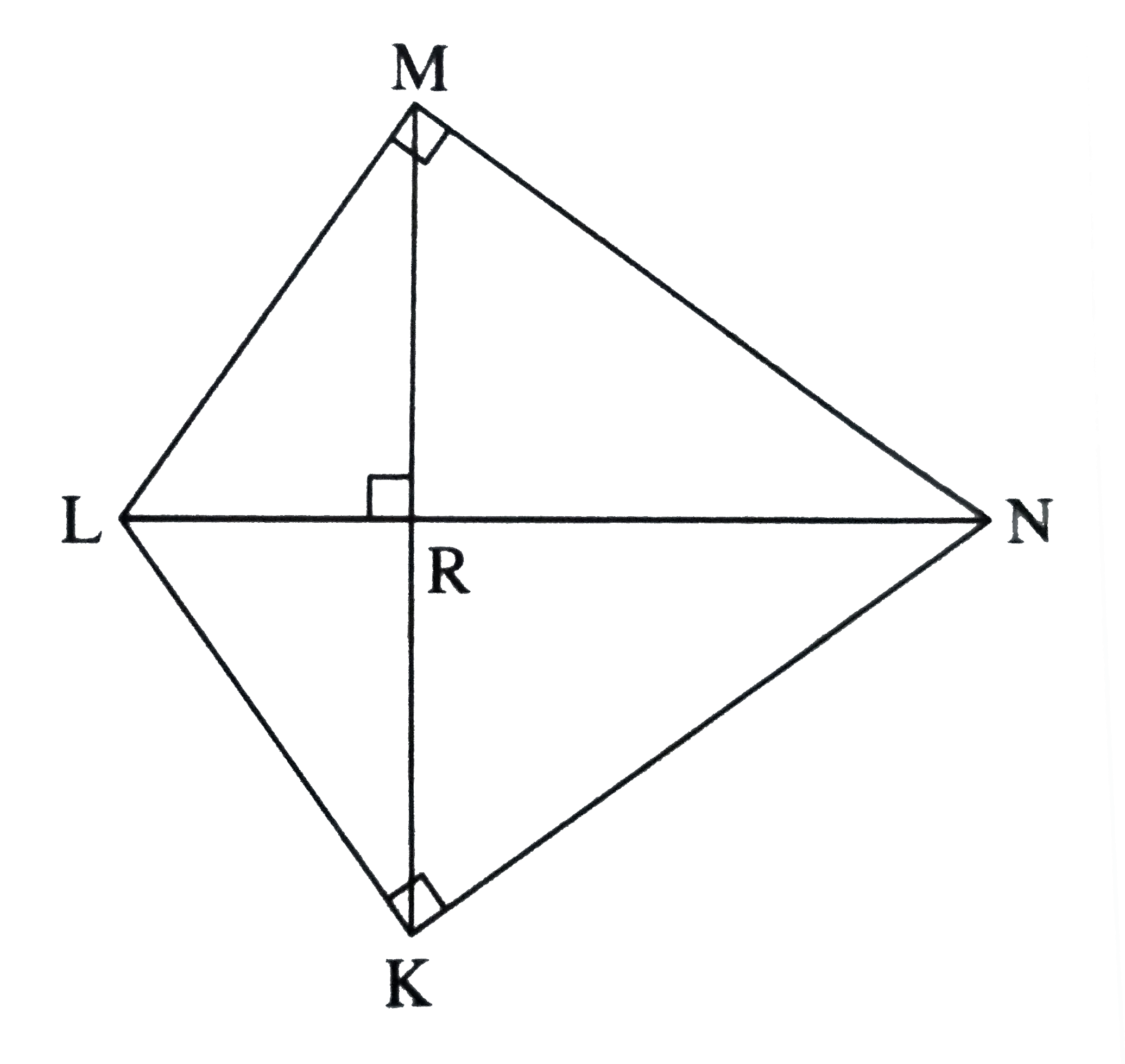
In the figure , ∠LMN=∠LKN=90∘
seg MK ⊥ seg LN .
Complete the following activtiy
to prove R is the midpoint of seg MK .
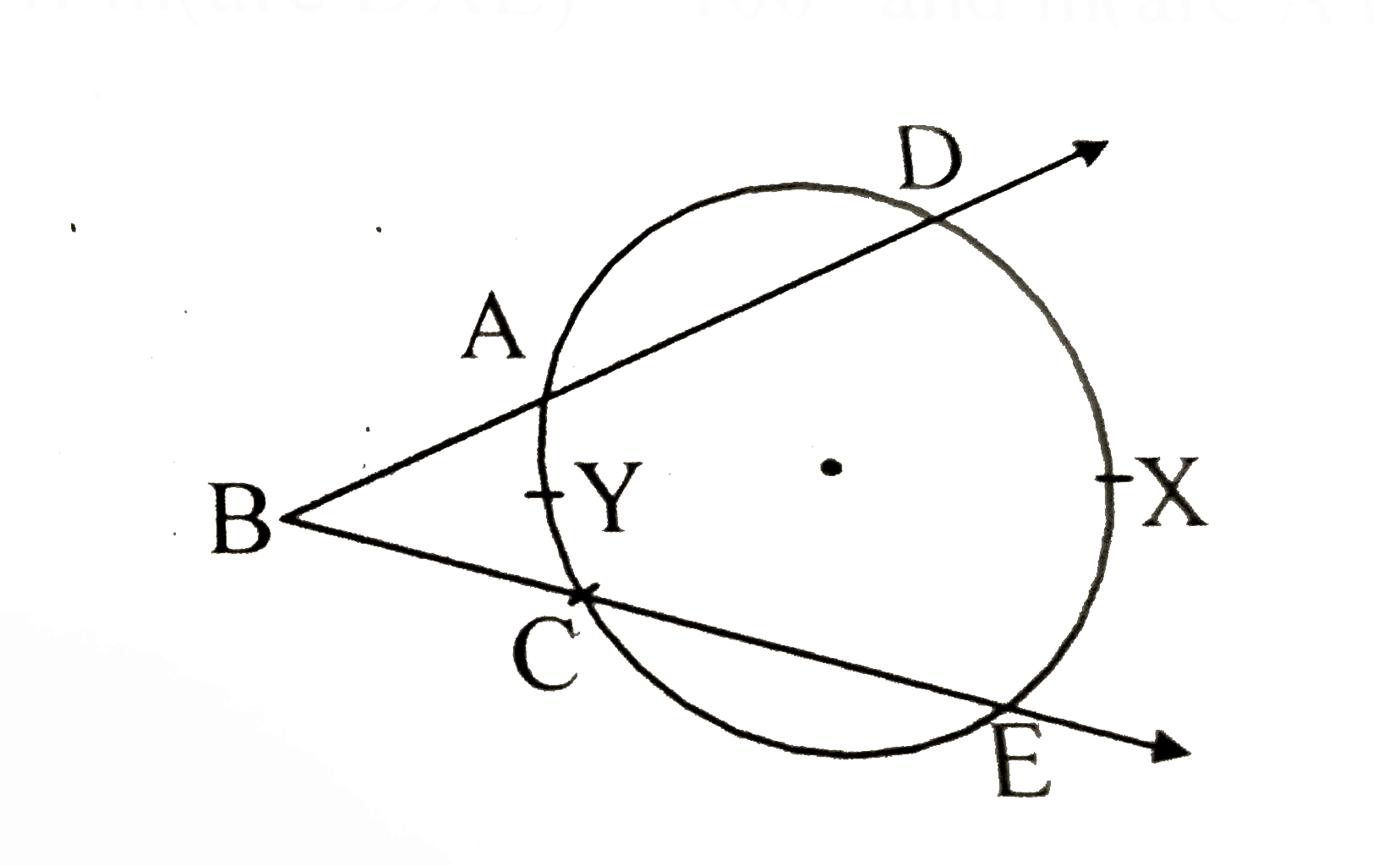
View SolutionIn the following figure, if m(arc DXE) =100∘ and m(arc AYC) =40∘, find ∠DBE.
View SolutionIn the following figure, O is the centre of the circle. ∠ABC is inscribed in arc ABC and ∠ABC=65∘. Complete the following activity to find the measure of ∠AOC
Activity :
∠ABC=12m□ …..(Inscribed angle theorem)
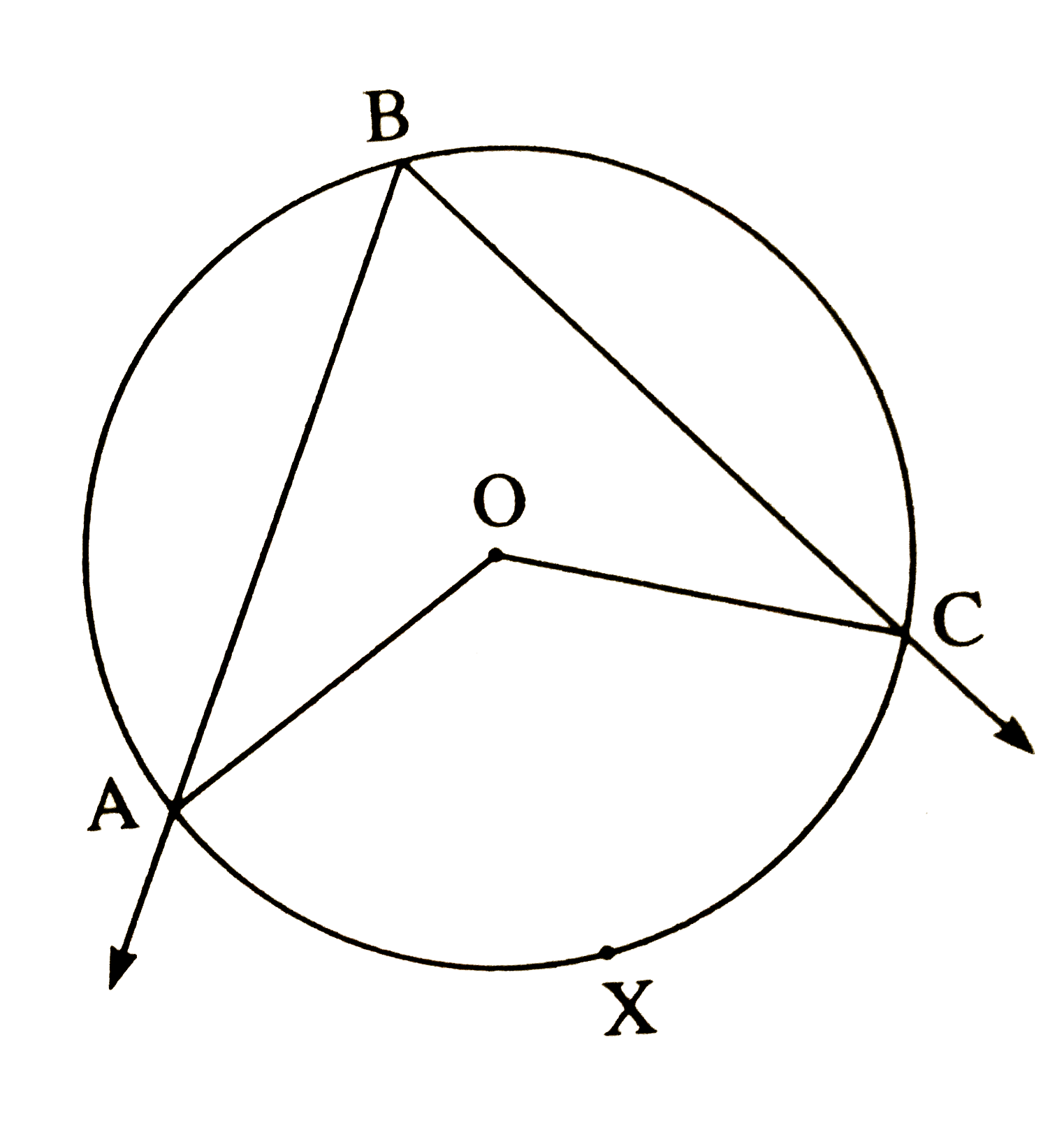
∴□×2m (arc AXC)
∴m (arc AXC = □
∠AOC=m (arc AXC)... (Definition of measure of minor arc)
∴∠AOC=□
View SolutionIn ΔLMN, if LM=10cm and ∠LNM=90∘, ∠LMN=30∘, then NM=?
View SolutionProve that, angles inscribed in the same arc are congruent
∠PQR and ∠PSR are inscribed in the same arc.
Arc PXR is intercepted by the angles.
To prove :∠PQR≅∠PSR

Proof :
m∠PQR=12m(arcPXR) …...□
m∠□=12m(arcPXR) ....(2) □
∴m∠□=m∠PSR ......[ From (1) and (2) ]
∴∠PQR≅∠PSR ...(Angles equal in measure are congrument )
View SolutionIn the figure, ∠LMN=∠LKN=90∘
seg MK⊥ seg LN.
Complete the following activity to prove R is the midpoint of seg MK.
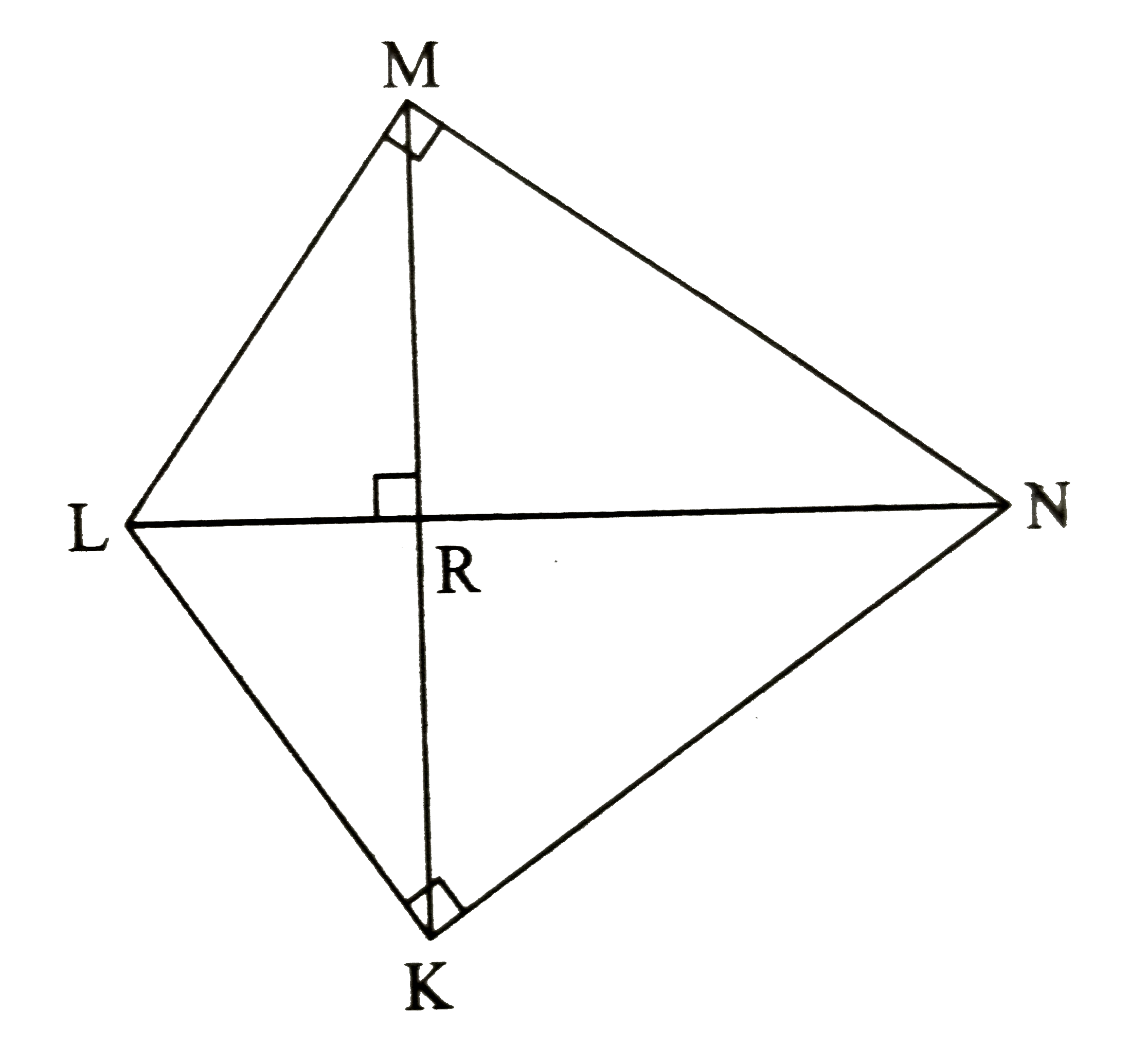
Proof: In ΔLMN, ∠LMN=90∘
seg MR⊥ hypotenuse LN
∴ by property of geometric mean,
MR2=□×RN
In ΔLKN, ∠LKN=90∘
seg KR⊥ hypotenuse LN
∴ by property of geometric mean,
KR2=LR×□
From (1) and (2), we get
MR2=□∴MR=□.
∴R is the midpoint of seg MK.
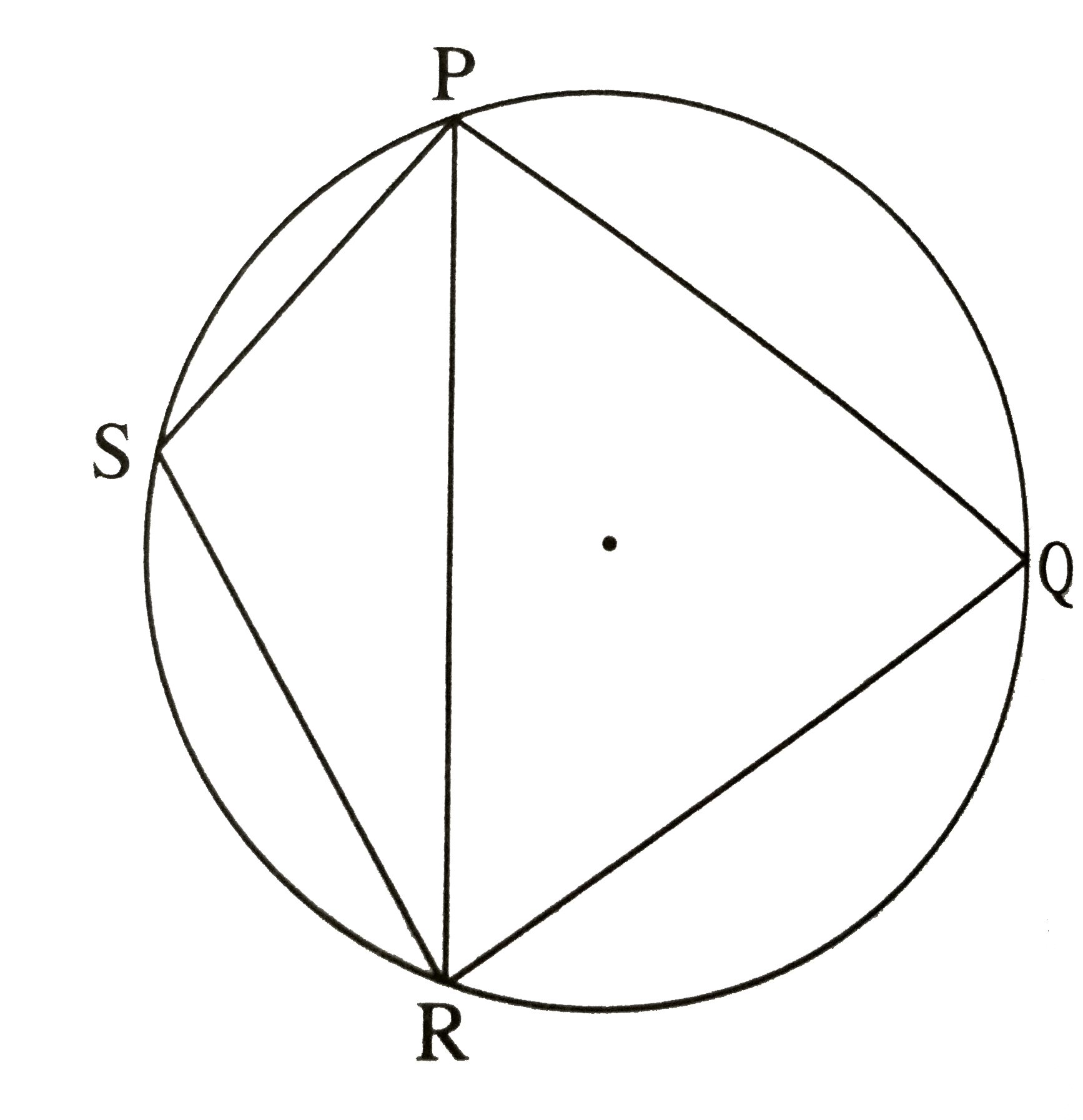
View SolutionIn the figure, □PQRS is cyclic side PQ ≅ RQ, ∠PSR=110∘, Find
m(arc PQR)
View Solution