A very long, strainght, thin wire carries −3.60nCm−1 of fixed negative charge. The wire is to be surrounded by a uniform cylinder of positive charge, radius 1.50 cm, coaxial with the wire. The volume charge density ρ of the cylinder is to be selected is zero. Calculate the required positive charge density ρ (in μCm−3).
6
7
5
3
The correct Answer is:C
We don't really need ot write an interal, we juset need the charge per unit length in the cyliner to be equal to zero. This means that the positive charge in cylinder must be +3.60nCm−1. This positive charge is uniformaly distributned in a circle of radius R=1.50 cm, so
ρ=3.60nCm−1πR2=3.60nCm−1π(0.015m)2=5μCm−3.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTIPSCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - NCERT Exemplar
5 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTIPSCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EXEMPLAR PROBLEMS
1 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT FINGERTIPSCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - HOTS
1 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NCERT FINGERTIPSCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Assertion And Reason
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT FINGERTIPSCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Assertion And Reason
15 Videos
Similar Questions
A copper rod of cross sectional area A carries a uniform current I through it. At temperature T if the volume charge density of the rod is ρ how long will the charges take to travel a distance d?
View SolutionA copper rod of cross sectional area A carries a uniform current I through it. At temperature T if the volume charge density of the rod is ρ how long will the charges take to travel a distance d?
View Solution
Knowledge Check
A very long, straight, thin wire carries −3.60nCm−1 of fixed negative charge. The wire is to be surrounded by a uniform cylinder of positive charge, radius 1.50 cm, coaxial with the wire. The volume charge density ρ of the cylinder is to be selected so that the net electric field outside the cylinder is zero. Calculate the required positive charge density ρ (in μCm−3).
A6B7C5D3
An infinity long cylinder of radius R has an infinitely long cylindrical cavity of radius R2 are shown in the figure. The remaining portion has uniform volume charge density ρ. The magnitude of electrical field at the centre of the cavity is-AρR6ε0BρR4ε0CρR2ε0DρR3ε0

An infinity long cylinder of radius R has an infinitely long cylindrical cavity of radius R2 are shown in the figure. The remaining portion has uniform volume charge density ρ. The magnitude of electrical field at the centre of the cavity is-
View SolutionA sphere of radius R_(0 carries a volume charge density proportional to the distance (x) from the origin, rho=alpha x where alpha is a positive constant.The total charge in the sphere of radius R_(0) is
View SolutionA charged belt 60 cm wide, travels at 20ms−1 between a source of charge and a sphere. The belt carries charges into the sphere at a rate corresponding to 120μA. Calculate the surface charge density of the belt.
View SolutionFigure shows a long wire having uniform charge density λ as shown in figure. Calculate electric field intensity at point P.
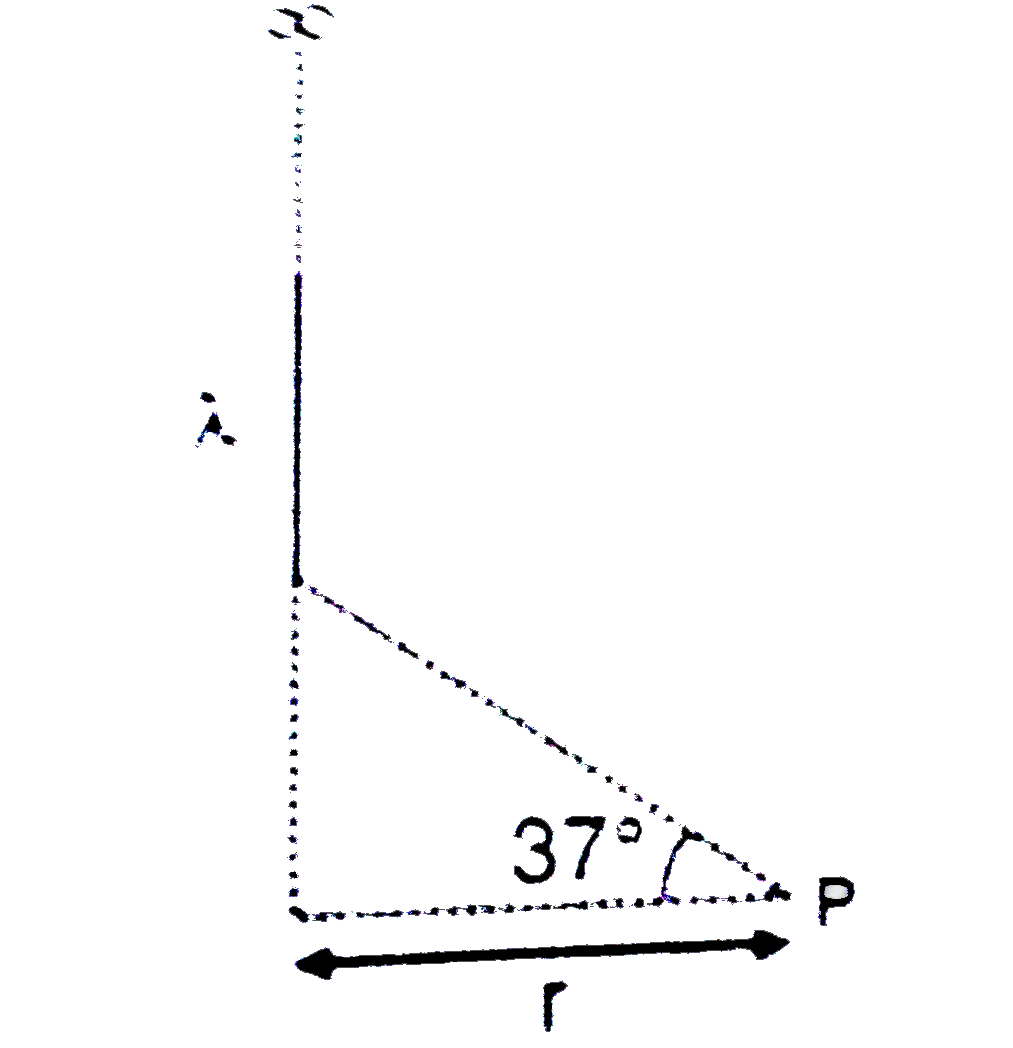
View SolutionFigure shows a long wire having uniform charge density λ as shown in figure. Calculate electric field intensity at point P.
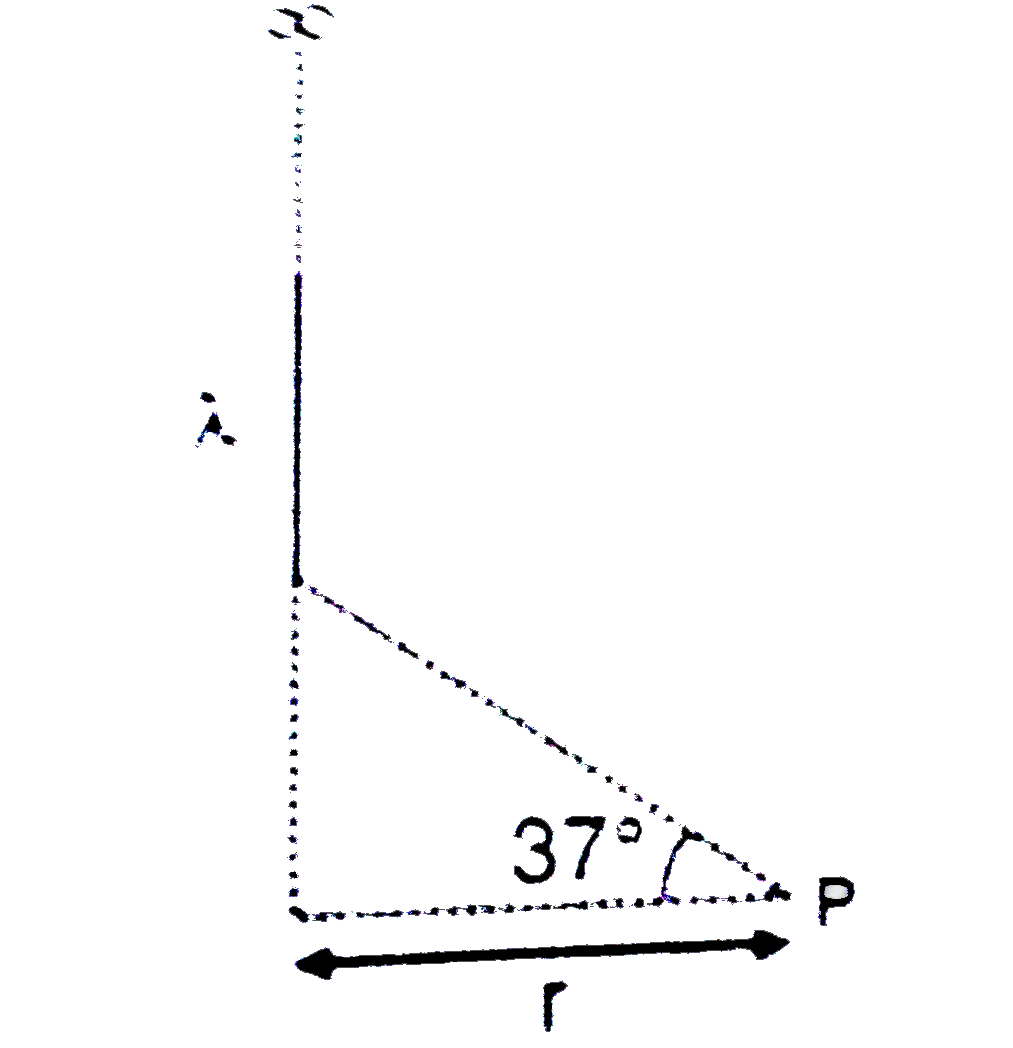
View SolutionA long cylinder of radius a carrying a uniform surface charge rotates about its axis with an angular velocity ω. Find the magnetic field energy per unit length of the cylinder if the linear charge density equals λ and μ=1.
View Solution
