If $α = 2 N_{0} λ$ , calculate the number of nuclei of A after one half-life of A, and also the limiting value of N as

A

$2 N_{0}, \frac{5}{2} N_{0}$

B

$3 N_{0}, 2 N_{0}$

C

$4 N_{0}, 2 N_{0}$

D

$\frac{3}{2} N_{0}, 2 N_{0}$

Text Solution

Verified by Experts

The correct Answer is:d

If $α = 2 λ N_{0}$ , t=half life = $\frac{I n (2)}{λ}$
$∴ N = \frac{1}{λ} [2 λ N_{0} - (2 λ N_{0} - λ N_{0}) e^{- λ t}]$
or $N = \frac{λ N_{0}}{λ} [2 - e^{I n (2)}] [H e r e e^{- I n (2)} = 2^{- 1} = \frac{1}{2}]$
or $N = \frac{λ N_{0}}{λ} [2 - \frac{1}{2}] = \frac{3 N_{0}}{2}$ or $N = \frac{3}{2} N_{0}$
When $t \to \infty$ and $α = 2 λ N_{0}$
$N = \frac{α}{λ} = \frac{2 λ N_{0}}{λ} = 2 N_{0}$ or $N = 2 N_{0}$

|

Class 12 PHYSICS NUCLEI

Topper's Solved these Questions

Similar Questions

Knowledge Check

Nuclei of a radioactive element 'A' are being produced at a constant rate, $α$ . The element has a decay constant, $λ$ . At time, t = 0, there are $N_{0}$ nuclei of the element.
If $α = 2 N_{0} λ$ the number of nuclei of A after one half life of A becomes
Azero
B $2 N_{0}$
C $1.5 N_{0}$
D $0.5 N_{0}$
Nuclei of a radioactive element 'A' are being produced at a constant rate $α$ the element has a decay constant $λ$ At time $t = 0$ there are $N_{0}$ nuclei of the element
If $α = 2 N_{0} λ$ the number of nuclei of A after undergone radioactivity decay till time $t = \frac{l n 2}{λ}$ :
A $0.5 N_{0}$
B $1.5 N_{0}$
C $\frac{(4 l n 2 - 1) N_{0}}{2}$
D $\frac{(4 l n 2 - 3) N_{0}}{2}$
Consider radioactive decay of $A$ to $B$ with which further decays either to $X$ or $Y$ , $λ_{1}, λ_{2}$ and $λ_{3}$ are decay constant for $A$ to $B$ decay, $B$ to $X$ decay and Bto $Y$ decay respectively. At $t = 0$ , the number of nuclei of $A, B, X$ and $Y$ are $N_{0}, N_{0}$ zero and zero respectively.
$N_{1}, N_{2}, N_{3}$ and $N_{4}$ are the number of nuclei of $A, B, X$ and $Y$ at any instant $t$ .

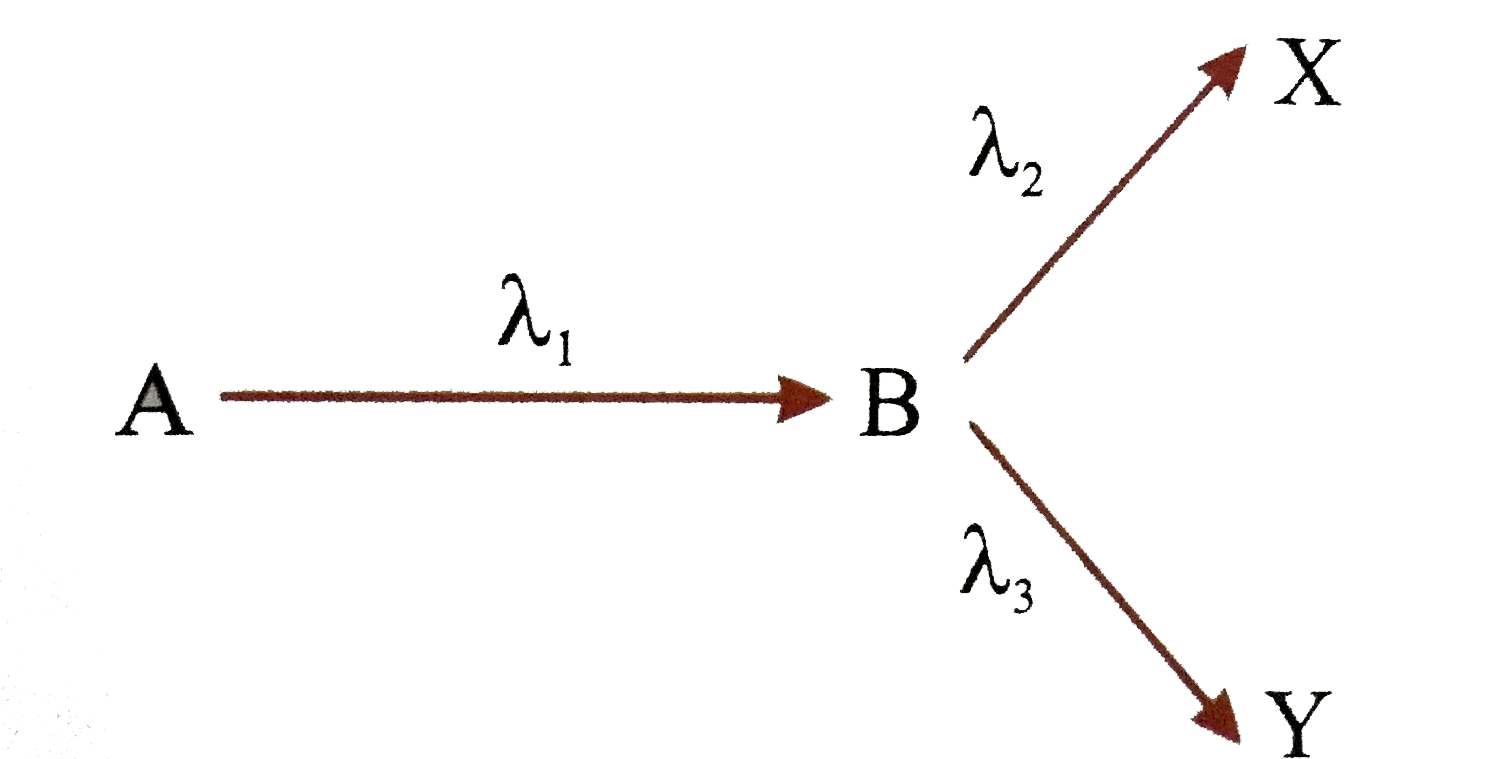
The number of nuclei of $B$ will first increase and then after a maximum value, it decreases for
A $λ_{1} > λ_{2} + λ_{3}$
B $λ_{1} = λ_{2} = λ_{3}$
C $λ_{1} = λ_{2} + λ_{3}$
DFor any values of $λ_{1}, λ_{2}$ and $λ_{3}$

NCERT FINGERTIPS-NUCLEI-Higher Order Thinking Skills

Home
Ask Doubt
Profile