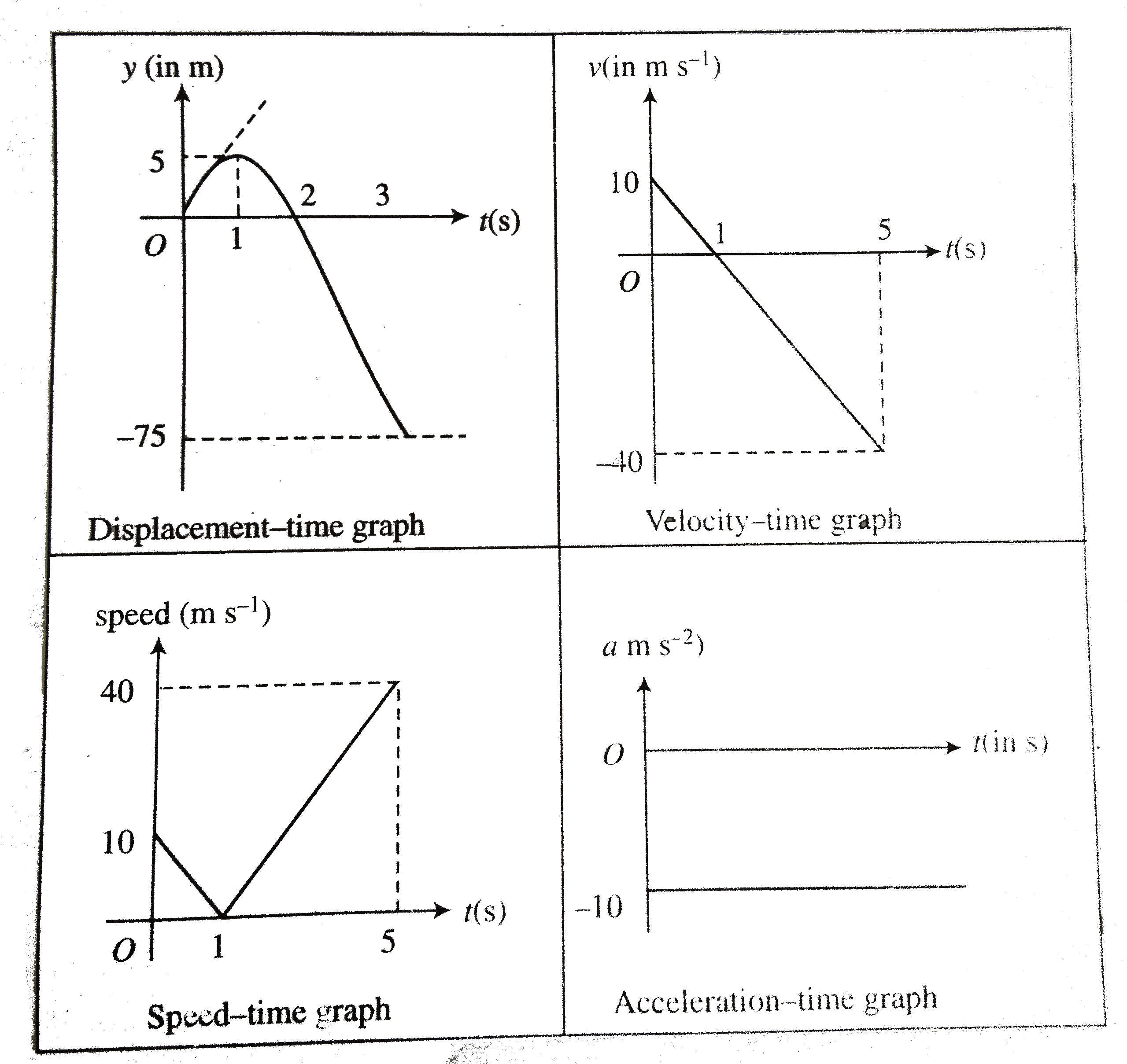
Referring to a−x graph, find the velocity when thedisplacement of the particle is 100m. Assume intial velocity as zero.
 .
.
We can find relation between a and x as:
Using (y−y1=(y2−y1x2−x(1)(x−x1
(a−3)=6−3250−50(x−50)⇒a=3100x+32
 .
.
But v=√v20+2(A)
As v0=0⇒v2=2∫adx
⇒a=3100x32
⇒v2=2 [Area of (a-x) graph upto x=100m]
=2[12(32+92)100]=600⇒v=10√6ns−1.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Solved Examples9videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.117videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE1videos
GRAVITATION
- BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer9videos
KINEMATICS-2
Similar Questions
A particle moves along the x-axis obeying the equation x=t(t−1)(t−2), where x is in meter and t is in second
a. Find the initial velocity of the particle.
b. Find the initial acceleration of the particle.
c. Find the time when the displacement of the particle is zero.
d. Find the displacement when the velocity of the particle is zero.
e. Find the acceleration of the particle when its velocity is zero.View SolutionReferring a−s diagram in , find the velocity after particle travel 120m from starting. Assume v0=0.
 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
Graph between velocity and displacement of a particle, executing S.H.M. is
AA straight lineBA parabolaCA hyperbolaDAn ellipse - Question 2 - Select One
In a linear S.H.M., the acceleration of the particle is zero, when its velocity is
AZeroBMaximumCHalf of its maximum valueDMinimum - Question 3 - Select One
Find the time when the displacement of the particle becomes zero. Assume velocity and position at t=0 to be and −10m respectively.
 A1sB√53sC5sD2s
A1sB√53sC5sD2s
Referring to a−s diagram as shown in , findthe velocity of the particle when the particle when the spaarticle justcovers 20m, (v0=√50ms−1.
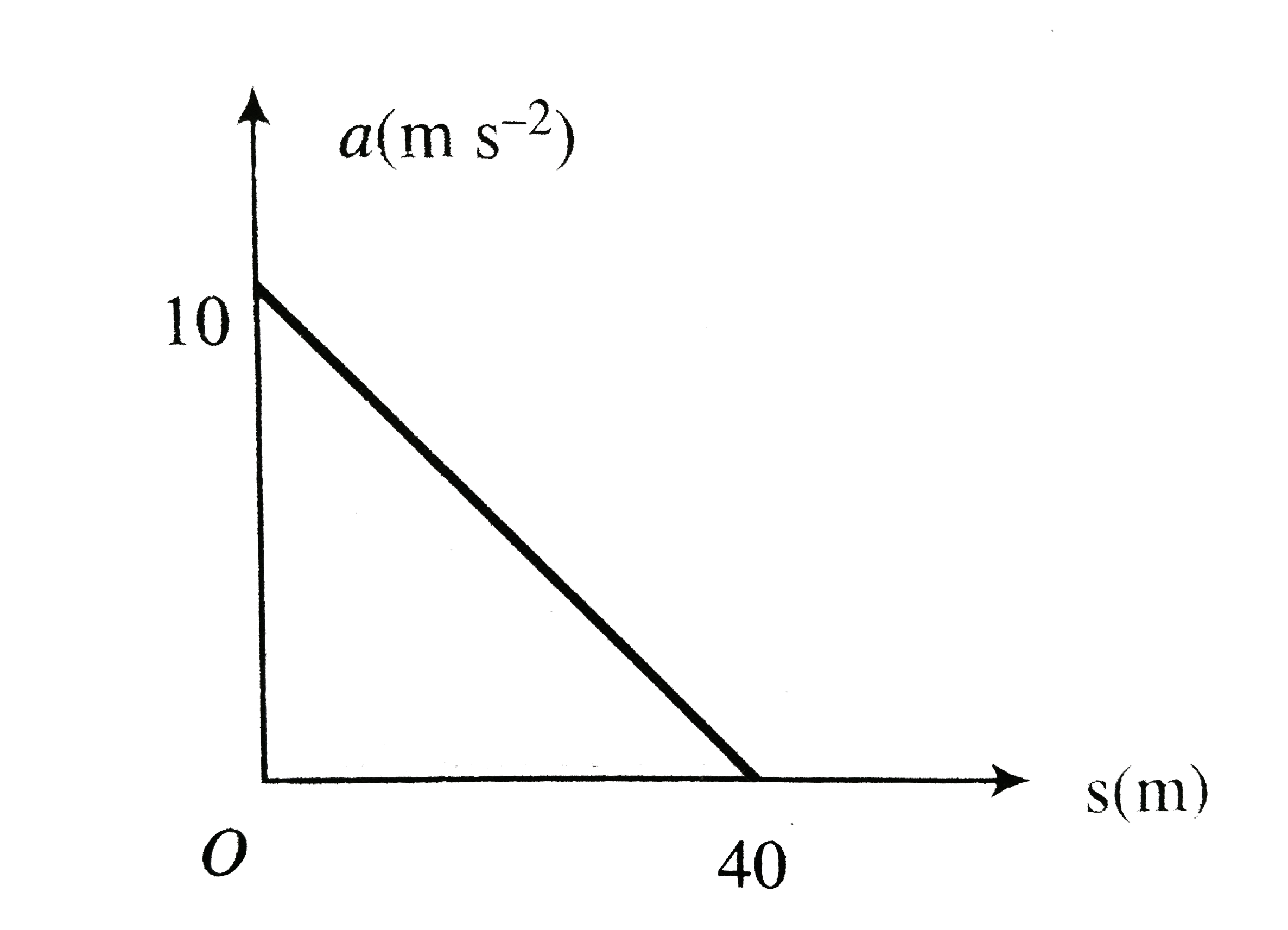 .View Solution
.View SolutionRefering to v−s diagram, find:
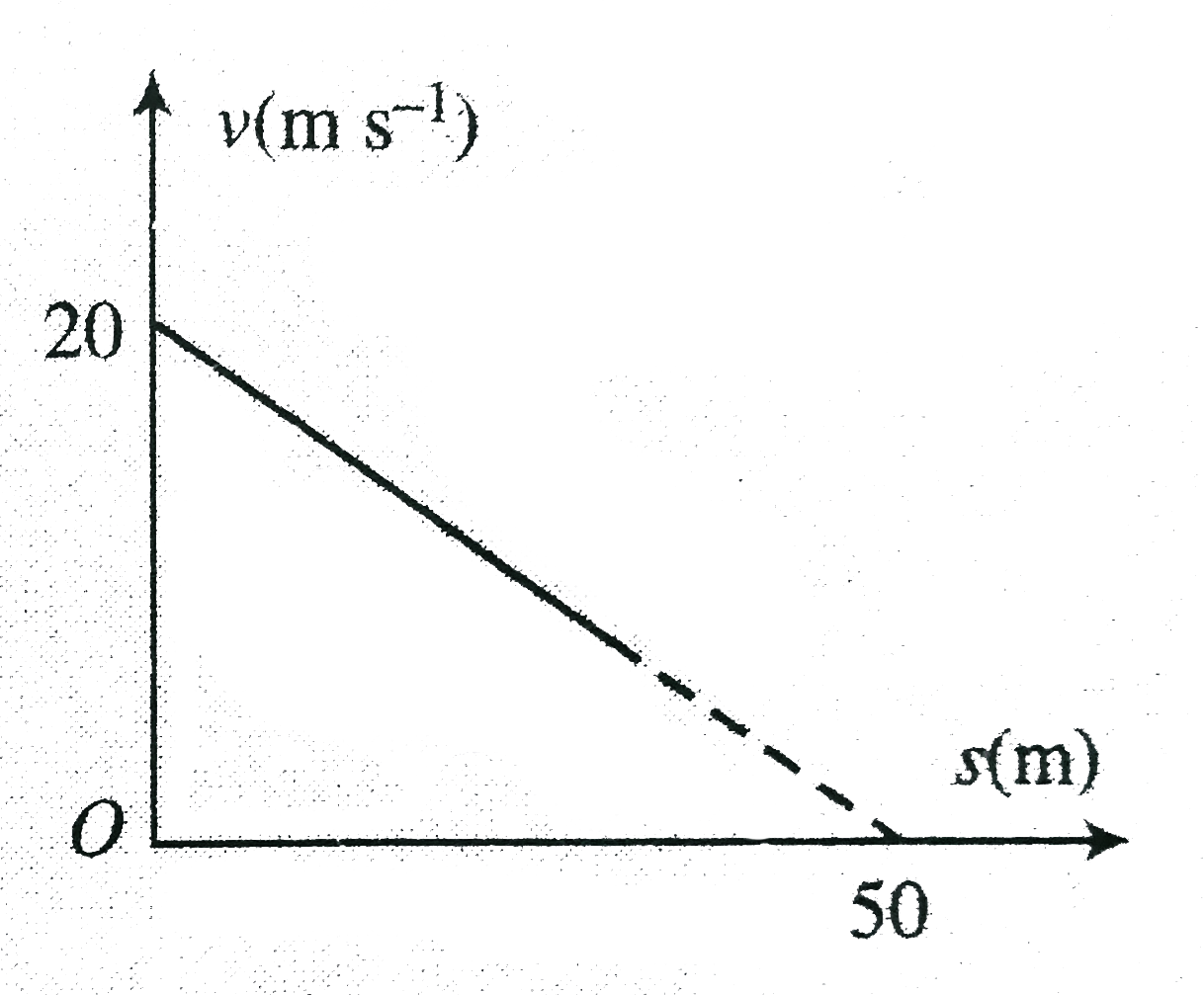 .
.
a. Acceleration of the particle when its velocity becomes half of the initial velocity.
b. Total distance covered by the particle.View Solutionvelocity time graph of a particle is shown in figure. Find displacement of the particle.
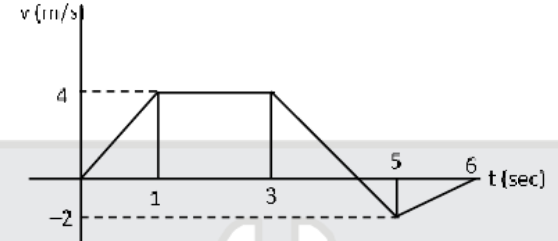 View Solution
View SolutionThe displacement x of a particle moving in one dismension under the action of a constant force is frlated to time t by the equation t√x+3, where x is in merers and t is in seconds, Find the displacemect of the particle when its velocity is zero.
View SolutionThe velocity − time graph of a particle is as shown in figure
 View Solution
View Solution

