A balloon in ascending vertically with an acceleration of $1 m s^{- 2}$ . Two stones are dropped from it at an interval of $2 s$ . Find the distance berween them $1.5 s$ after the second stone is released.

Video Solution

Text Solution

Verified by Experts

Let at any time $t = 0$ , the balloon be position $A$ , where its velocity is $u$ . At $t = 2 s$ , it reaches $B$ , where its velocity becomes $v$ , then
.
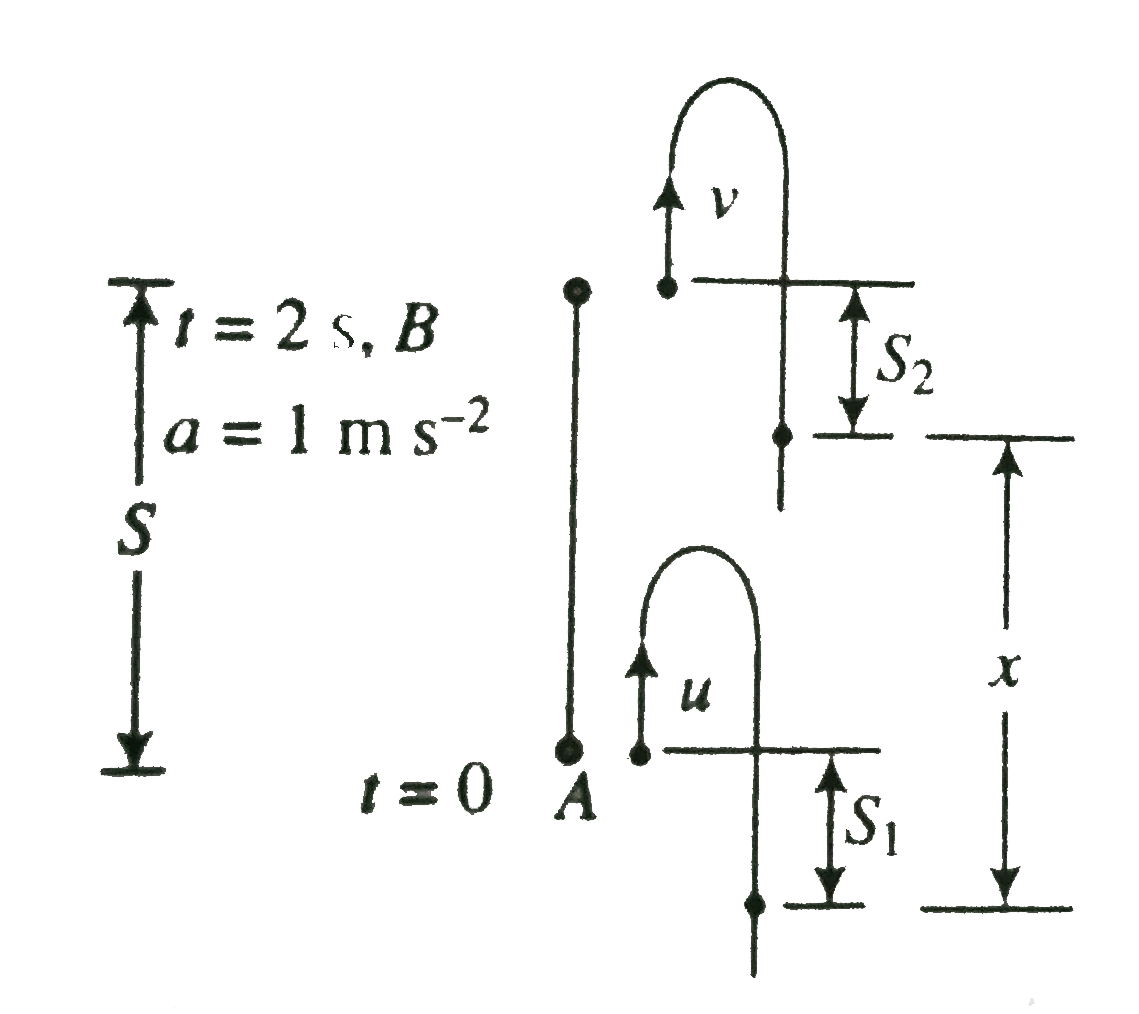$A B = S = u \times 2 + \frac{1}{2} a {(2)}^{2} = 2 u + 2$
Also $v = u + a \times 2 = u + 2$ ,
First stone: $- S_{1} = u \times 3.5 + \frac{1}{2} (- g) {(3.5)}^{2}$
Second stone: $S^{2} = v \times 1.5 + \frac{1}{2} (- g) {(1.5)}^{2}$
Required distance between the stones
$x = S_{1} + S - S_{2}$
Solve to get $x = 55 m$ ,
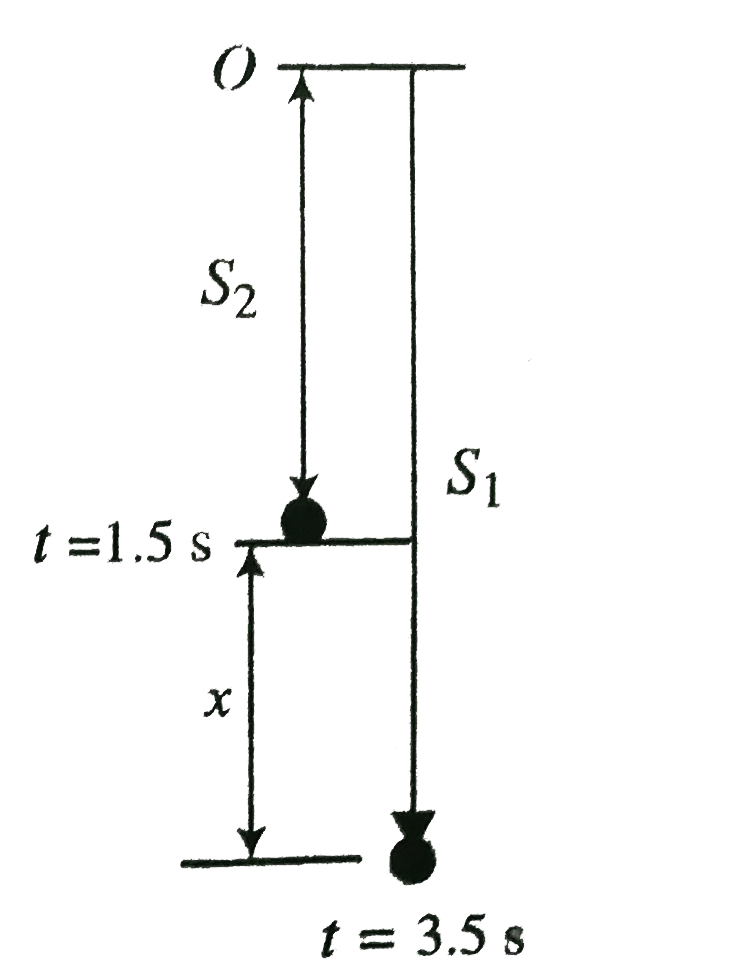
Alterntively: This method can be understood in proper way after studying relative velocity
If we work from the frame of balloon, then the acceleration of each stone w,r.t balloon will be $g + a$ after releasing from it. The initial velocity of each stone will be zero w.r.t. balloon.
.
$S_{1} \frac{1}{2} (g + a) {(3.5)}^{2}, S_{2} = \frac{1}{2} (g + a) {(1.5)}^{2}, x = S_{1} - S_{2} = 55 m$ .

Updated on:21/07/2023

A balloon is ascending vertically with an acceelration of $0.2 m s^{- 2}$ .Two stones are dropped from it at an interval fo $2 s$ , the distance between then when the second stone dropped is (tanke g=9.i8 ma^(-2).
A(a) $0.4 m$
B(b) $4.9 m$
C© 19 .6 m
D( 20 .0 m.
A balloon is rising vertically upwards with uniform acceleration 15.7m/ $s^{2}$ .A stone is dropped from it. After $4 s$ another stone is dropped from it.Find the distance between the two stones 6 second after the second stone is dropped.
A616 m
B716 m.
C816 m
DNone of these
From a balloon rising vertically with uniform acceleration f a ball is dropped, 4 seconds after this another ball is dropped from the balloon. The distance. between the two balls 2 seconds after the second ball is dropped is
A16 (g+f)
B10 (g+f)
C8 (g+f)
DNone