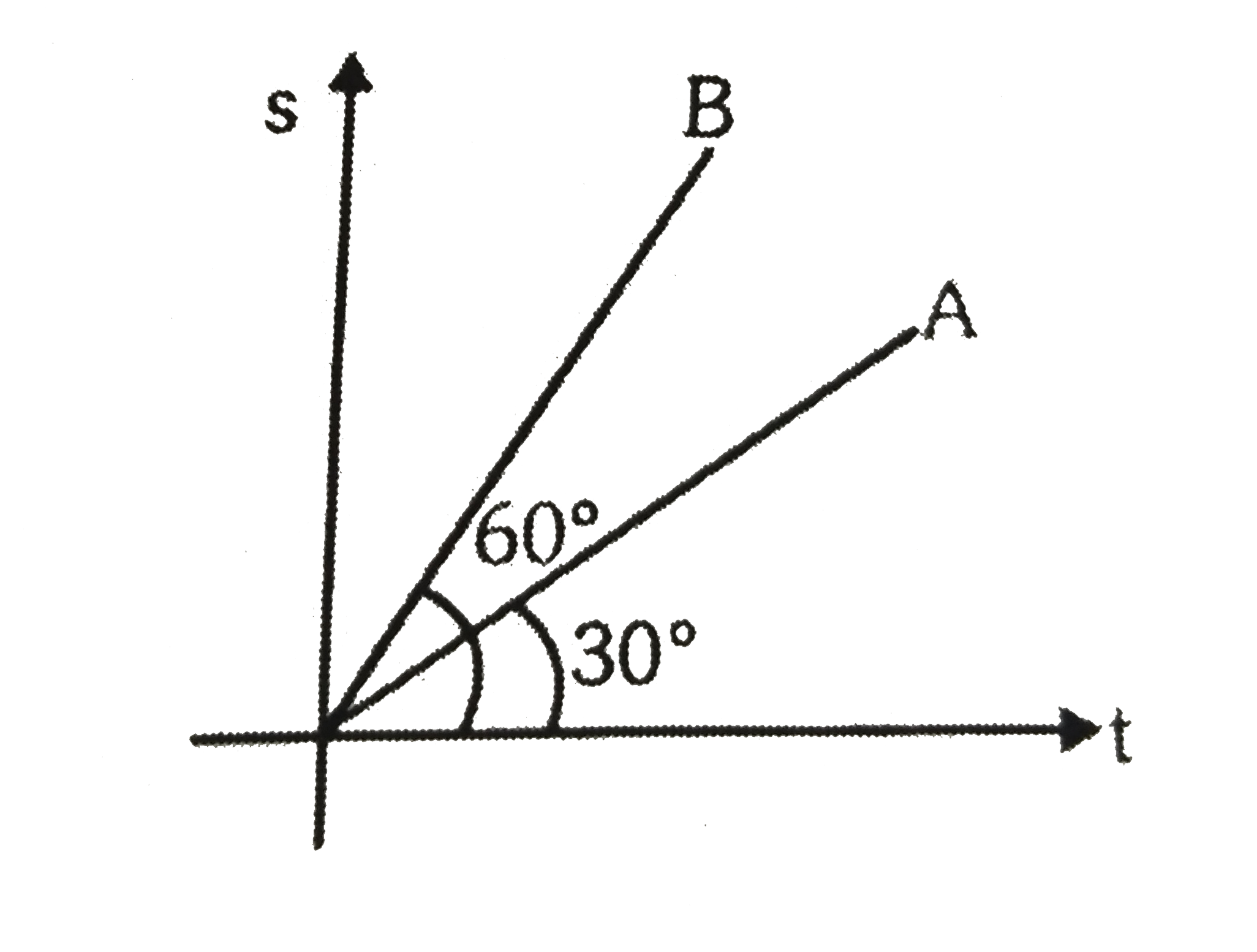
Two particles are moving with uniform velocity as shown in (Fig. 5.130) given below at .
(a) Will the two particles collide ?
(b) Find out the shortest distance between two particles.
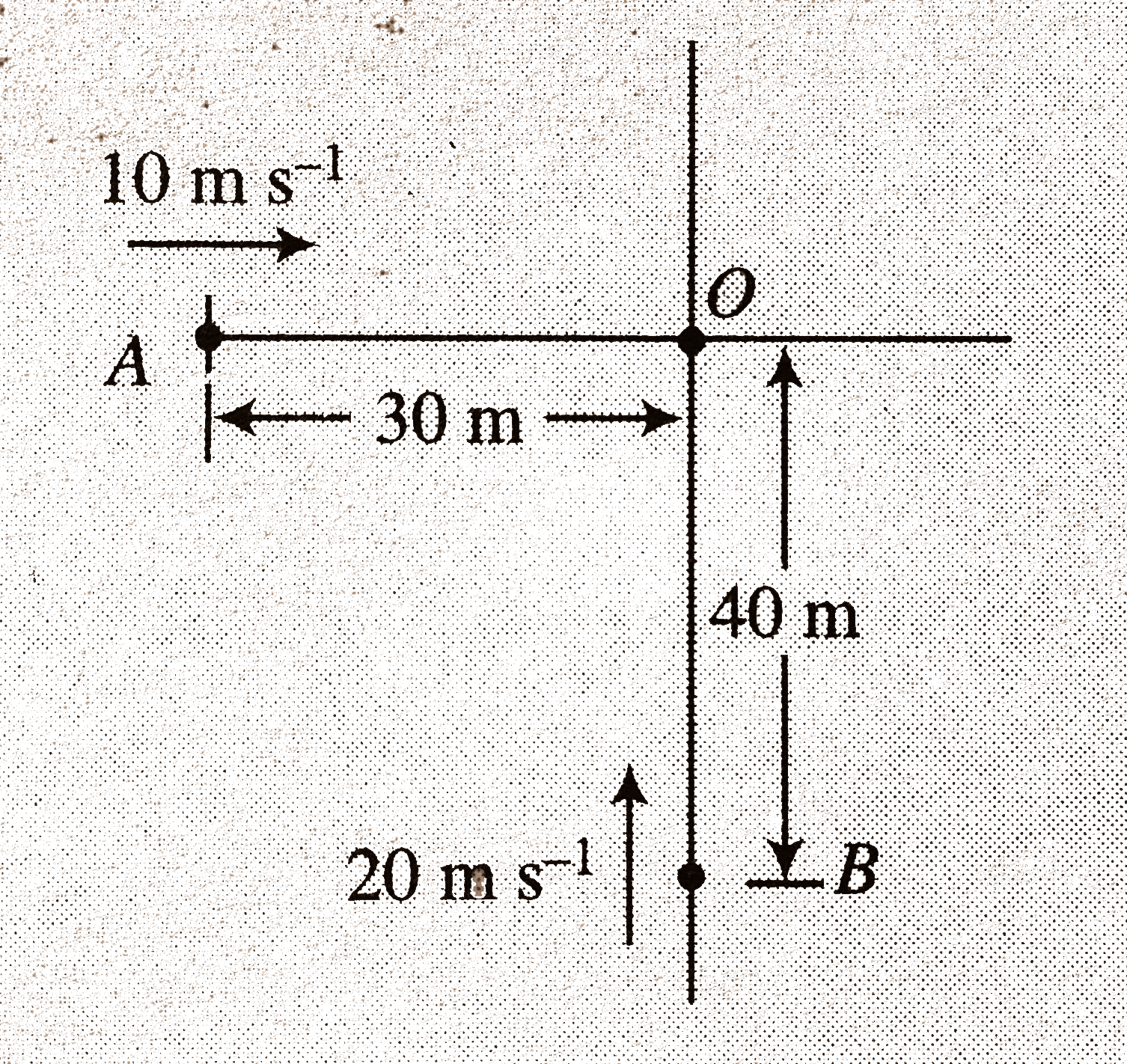 .
.
Observing from the frame of
Let us draw ,
Again
Hence, the shortest distance between the particles
Since the closest distance is non - zero, therefore, the particles will not collide.
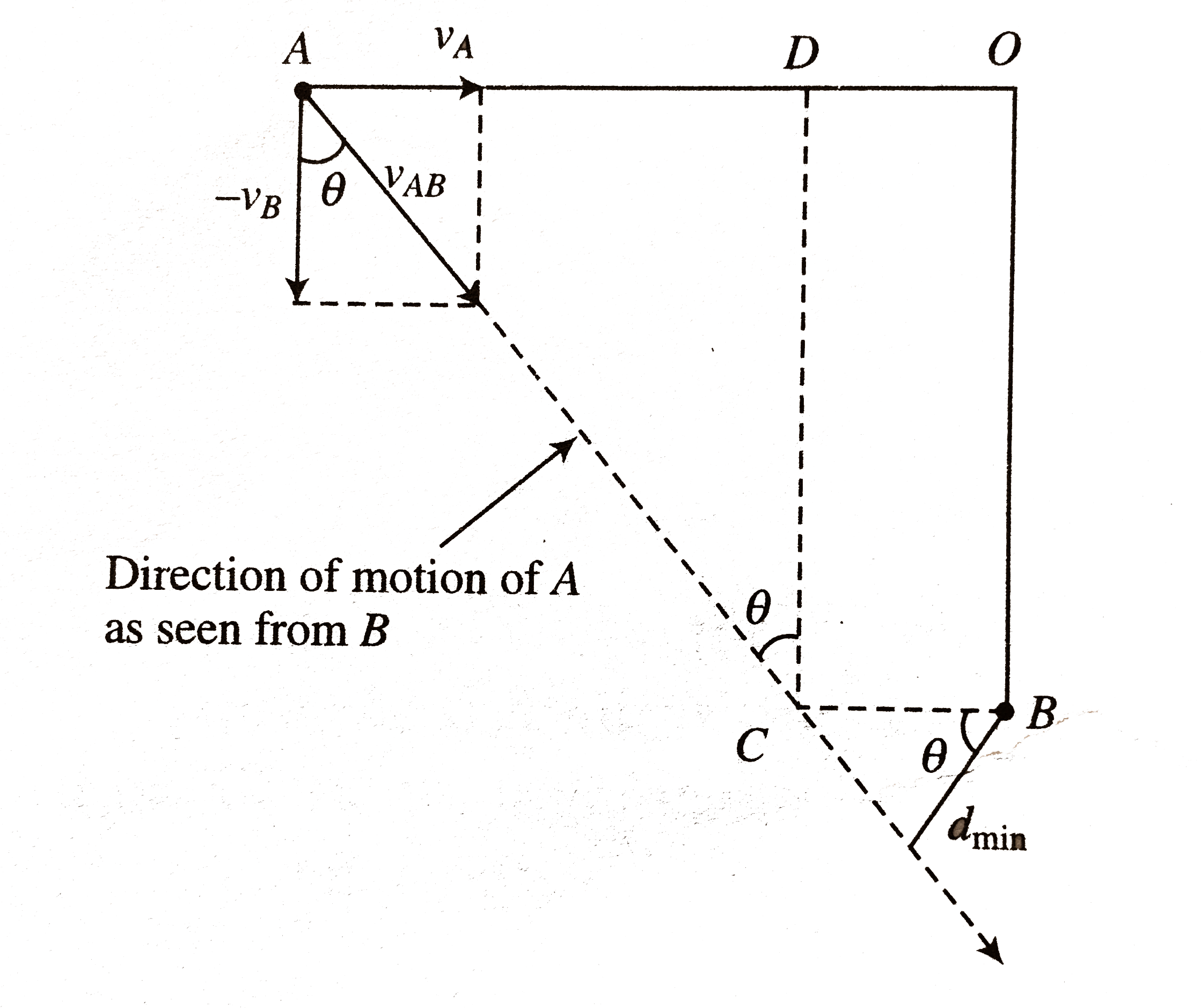 .
.
Topper's Solved these Questions
- KINEMATICS-2Book:CENGAGE PHYSICSChapter:KINEMATICS-2Exercise:Solved Examples
Explore 7 Videos
- KINEMATICS-2Book:CENGAGE PHYSICSChapter:KINEMATICS-2Exercise:Exercise 5.1
Explore 15 Videos
- KINEMATICS-1Book:CENGAGE PHYSICSChapter:KINEMATICS-1Exercise:Integer
Explore 9 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICSChapter:KINETIC THEORY OF GASESExercise:Compression
Explore 2 Videos
Similar Questions
Knowledge Check
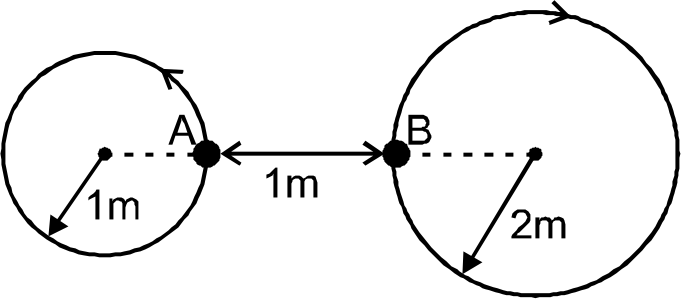
Two particles are moving in different circles in same plane with different angular velocities as shown in figure. At t = 0, initial positions of particles A and B are shown by dots on the respective circles. Initial distance between particles is 1m. Particle A move anticlockwise in the first circle whereas B moves clockwise in the second circle. Angle described (rotated) by A and B in time 't' are θA=(π2t) and θB=(πt) respectively. here θ is in radian and t is in second. Radius of each circle is shown in diagram.
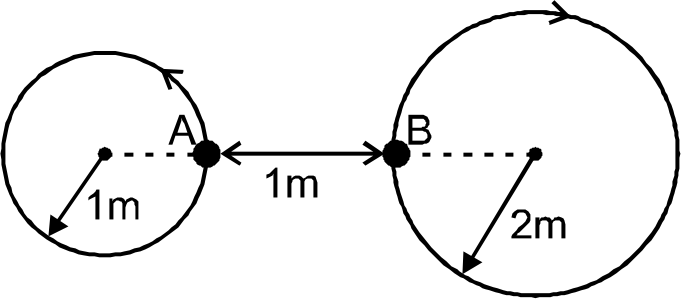
At time t=2 second, the angular velocity of the particle A with respect to the particle B isA5π rad/secB3π2 rad/secC2π3 rad/secD5π6 rad/secTwo particles are moving in different circles in same plane with different angular velocities as shown in figure. At t = 0, initial positions of particles A and B are shown by dots on the respective circles. Initial distance between particles is 1m. Particle A move anticlockwise in the first circle whereas B moves clockwise in the second circle. Angle described (rotated) by A and B in time 't' are θA=(π2t) and θB=(πt) respectively. here θ is in radian and t is in second. Radius of each circle is shown in diagram.
Find the magnitude of acceleration of A at t=1 secAπ23m/sBπ27Cπ24DNone of theseTwo particles are moving in different circles in same plane with different angular velocities as shown in figure. At t = 0, initial positions of particles A and B are shown by dots on the respective circles. Initial distance between particles is 1m. Particle A move anticlockwise in the first circle whereas B moves clockwise in the second circle. Angle described (rotated) by A and B in time 't' are θA=(π2t) and θB=(πt) respectively. here θ is in radian and t is in second. Radius of each circle is shown in diagram.
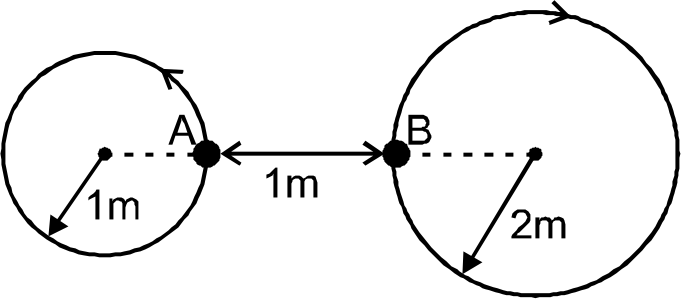
At time t=1 sec. the magnitude of acceleration of A with respect to B isAπ24√65ms2Bπ22√7ms2Cπ23√15ms2Dπ24√7ms2
Two particles start moving simultaneously with constant velocities u1andu2 as shown in (Fig. 5.194). First particle starts from A along AO and second starts from O along OM. Find the shortest distance between them during their motion.
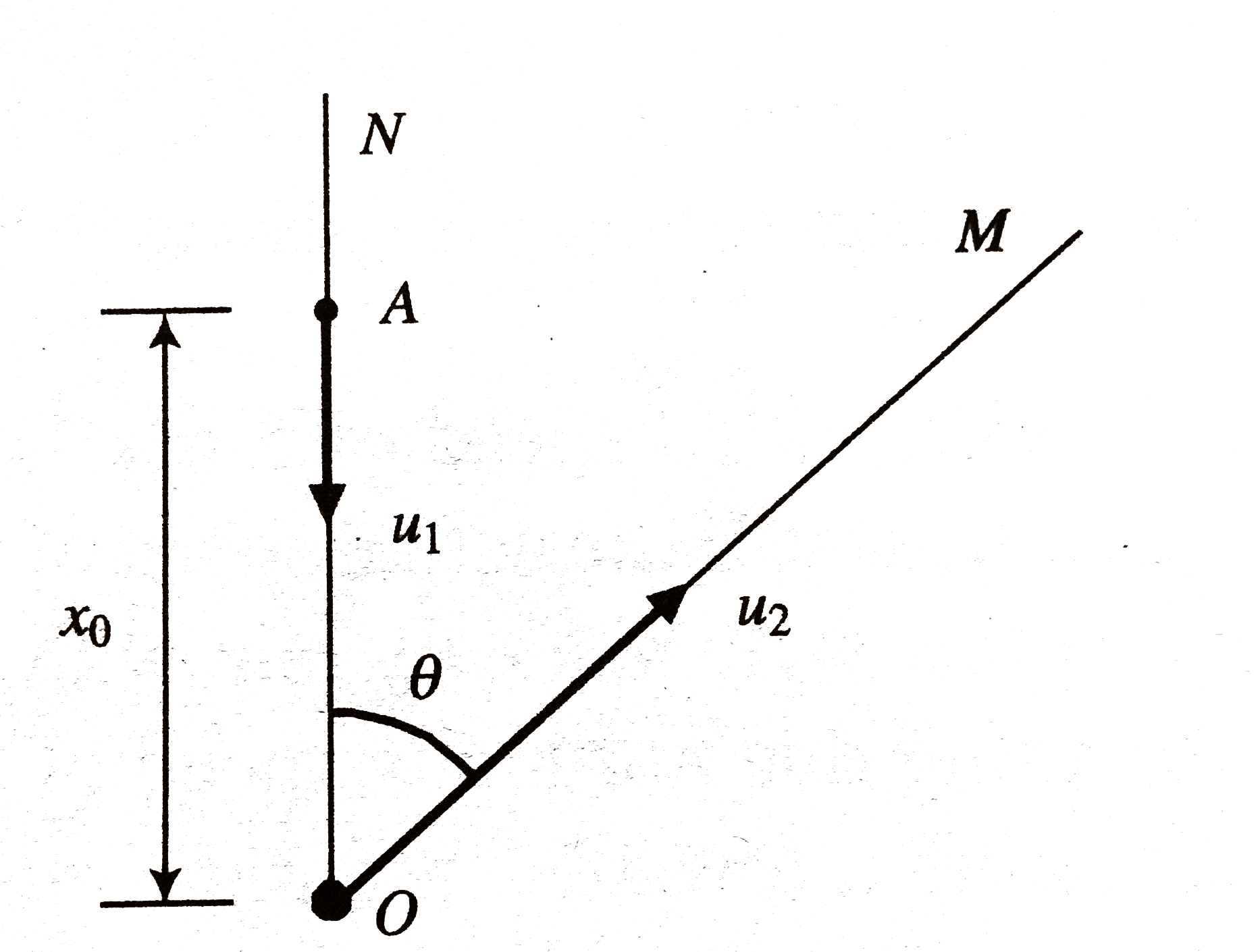 .
.
View SolutionTwo Particle A and B are moving with uniform velocities of 20m/sec and 10m/sec as shown in the shown in the figure. The Magnitude of angular velocity of A with respect to B.when their separation (AB) is 10 meter is
View SolutionTwo objects AandB are moving along the directions as shown in (Fig. 5.77). Find the magnitude and direction of the relative velocity of Bw.r.t.A.
 ,
,
View SolutionParticles AandB move with constant and equal speeds in a circle as shown in (Fig. 5.164). Find the angular velocity of the particle A with respect to B, if the angular velocity of particle Aw.r.t.O is ω.
 .
.
View SolutionTwo particles A and B are initially 40mapart, A is behind B. Particle A is moving with uniform velocity of 10ms−1 towared B. Particle B starts moving away from A with constant acceleration of 2ms−1.
The minimum distance between the two is .
View Solution