A student tries to raise a chain consisting of three identical links. Each link has a mass of 300g. The three-piece chain is connected to a string and then suspended vertically, with the student holding the upper end of the string and pulling upward. Because of the student's pull, an upward force of 12 N is applied to the chain by the string.
(a) Draw a free-body diagram for each of the links in the chain and also for the entire-chain considered a single body.
(b) Use the results of part(a) Newton's laws to find (i) the acceleration of the chain and (ii) the force exerted by the top link on the middle link.
The correct Answer is:103ms−2:8N
(a)T1 is the tension between link 1 and link 2. T2 is between link 2 and link 3.
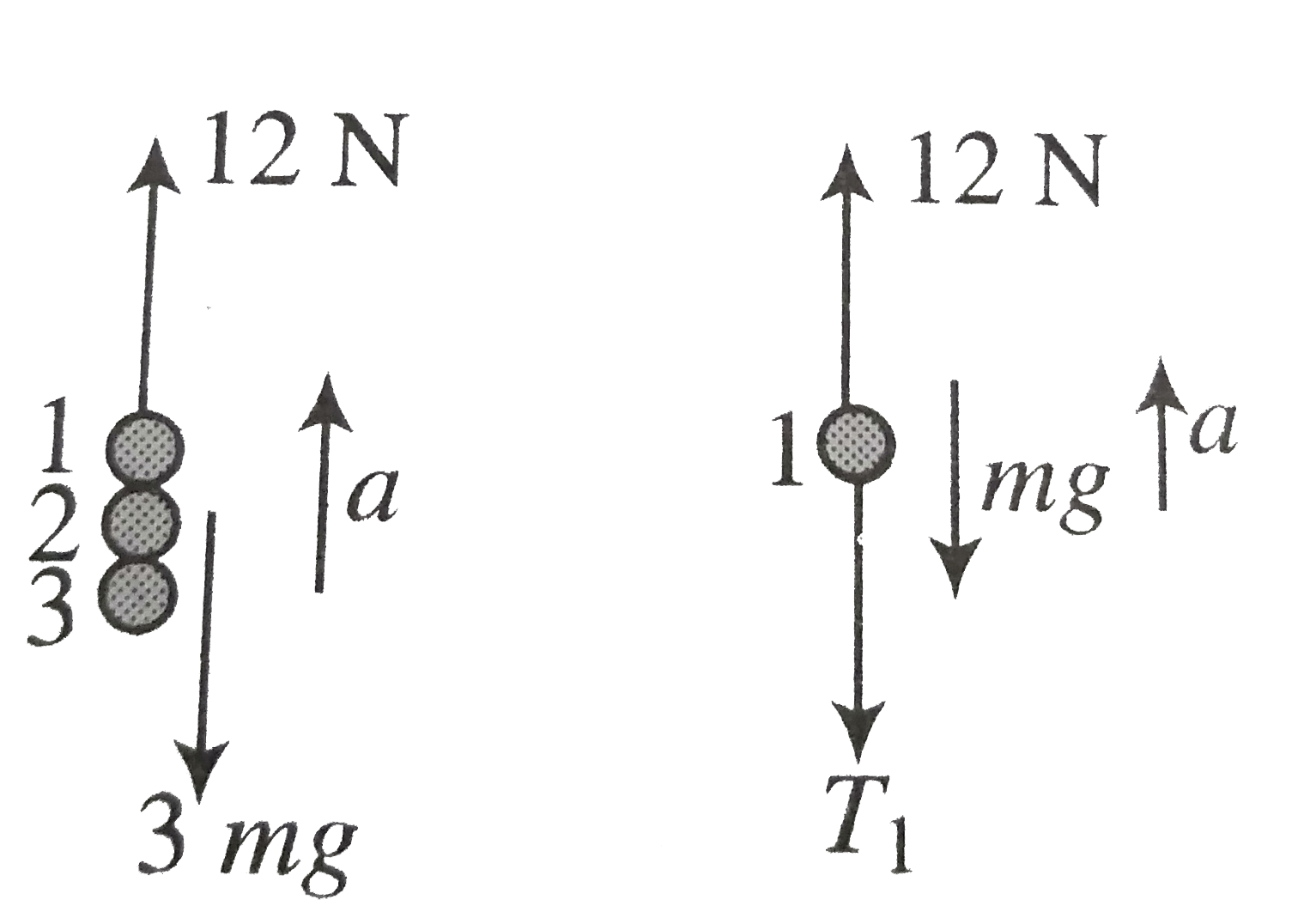
(b) a. a=Net forceMass=12−3(0.3g)3×0.3=103ms−2
b. We have to find T1 :
12−T1−mg=ma
⇒12−T1−3=0.3×103
⇒T1=8N.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Single Correct76videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Multiple Correct11videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.413videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE10videos
MISCELLANEOUS VOLUME 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type1videos
NEWTON'S LAWS OF MOTION 2
Similar Questions
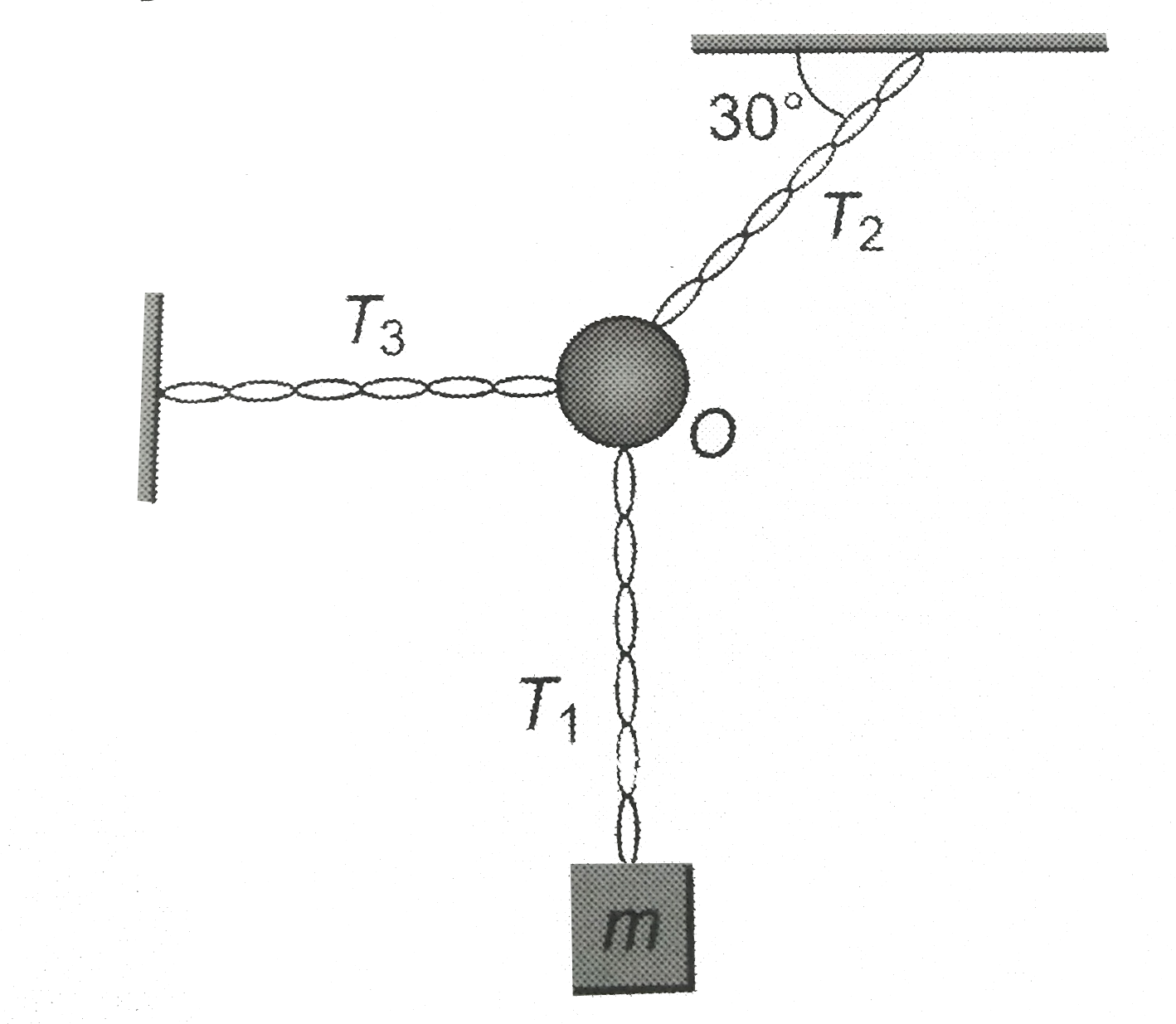
A block of mass m is attached to a chain as shown in the diagram. Find the tension in each of the three chains. The ring and chains are light.
 View Solution
View SolutionA very flexible chain of mass M and length l is suspended vertically in a lift so that its lower end is just touching the surface of the floor. When the upper end of the chain is released, it falls with each link coming to rest intantaneously find the force exerted by the floor of the lift on the chain at the moment when one fourth of the chain has already landed on the floor Assume the lift is moving with acceleration g/2
View Solution
Knowledge Check
- Question 1 - Select One
A student tries to raise a chain consisting of three identical links. Each link has a mass of 300gm. The three piece chain is connected to a string and suspended vertically, while the student holding the upper end of he string, pulling it upward. Beacause of the student's pull an upwards force of 12N is applied to the chain by the string. (g=10m/s2)
Acceleration of chain isA10m/s−2B13.33ms−2C3.3ms−2D40ms−2 - Question 2 - Select One
A student tries to raise a chain consisting of three identical links. Each link has a mass of 300gm. The three piece chain is connected to a string and suspended vertically, while the student holding the upper end of he string, pulling it upward. Beacause of the student's pull an upwards force of 12N is applied to the chain by the string. (g=10m/s2)
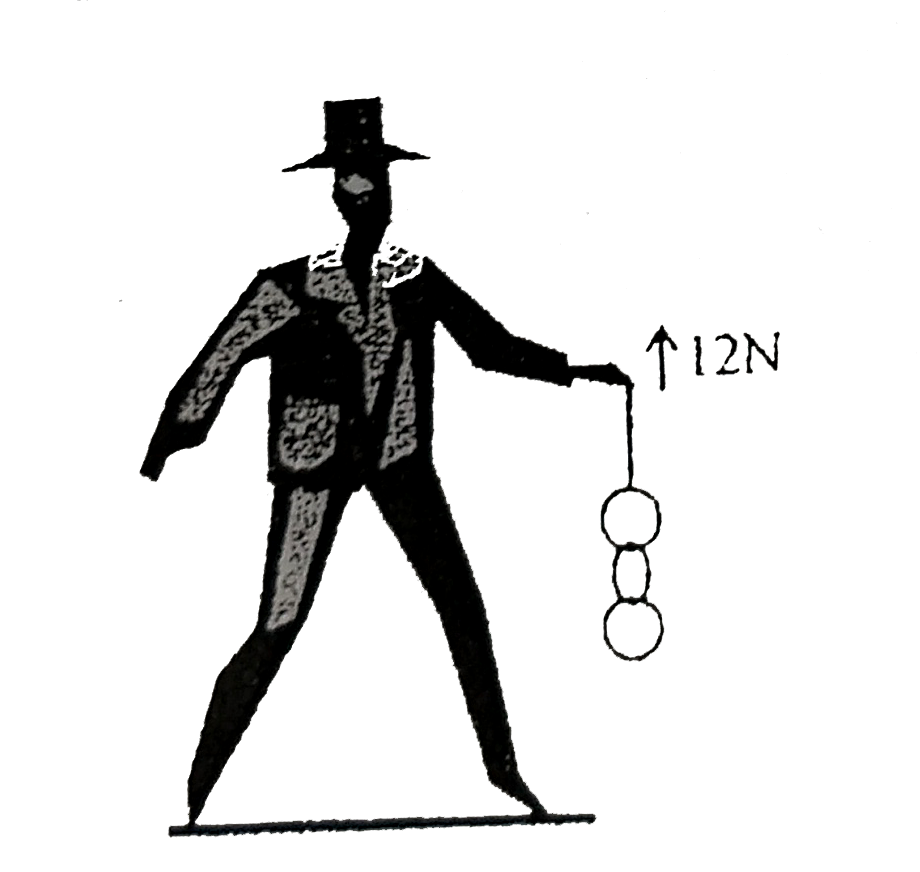
The force exerted by the top link on the middle link.A4NB8NC12 ND3 N - Question 3 - Select One
A body of mass M is being pulled by a string of mass m with a force P applied at one end. The force exerted by the string on the body is
APm(M+m)BPM(M+m)CPm(M+m)DP(M−m)
The blocks are attached by an inextensible light string and pulled vertically upward by force 100N as shows. Find the common acceleration and tension in the string
 View Solution
View Solution
Figure shows three identical yo-yos initially at rest on a horizontal surface. For each yo-yo the string is pulled In the direction shown. In each case there is sufficient friction for the yo-yo to roll without slipping. Draw the free-body diagram for each yo-yo in what direction will each yo-yo rotate?View SolutionA block of mass M is pulled on a smooth horizontal table by a string making an angle θ with the horizontal as shown in figure. If the acceleration of the block is a find the force applied by the string and by the table N on the block.
 View Solution
View SolutionIn insulin chain A and chain B are linked together by ........... bridges.
View SolutionThe acceleration of the 2 kg block if the free end of string is pulled with a force of 20 N as shown is
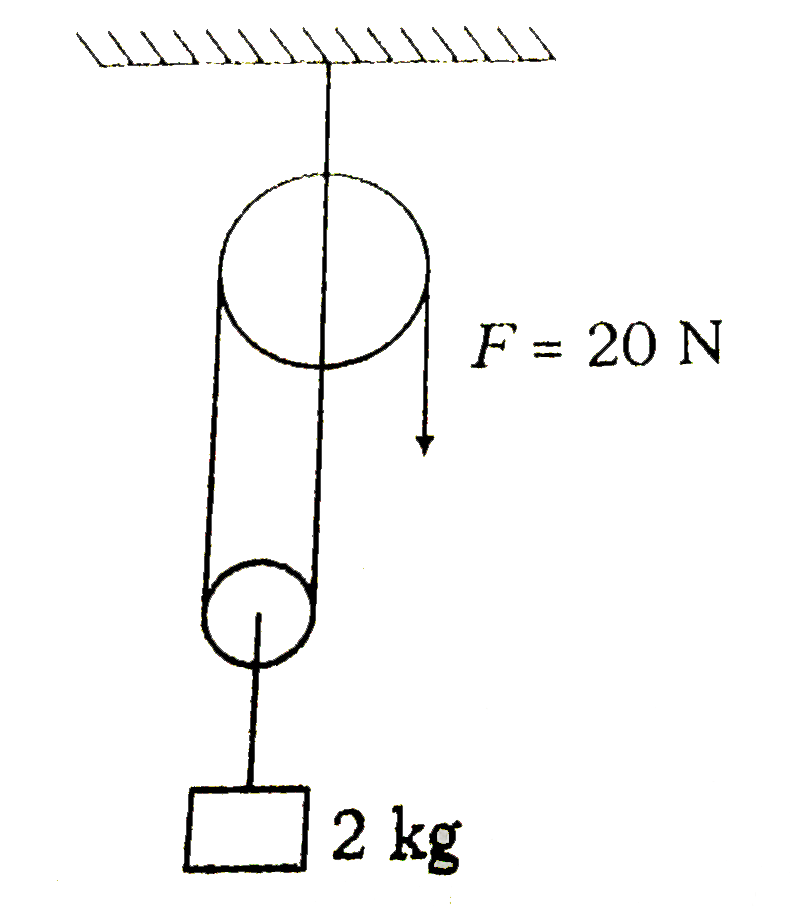 View Solution
View Solution

