Two small spheres of masses m1 and m2 are connected by a light right rod.The system is placed between a rought floor and smooth verticle wall as shown in Fig. The co-efficent of friction between the floor and the sphere of mass m2 isμ .Find the minimum value of θ so that the system of masses does not slip

For the equation of m1
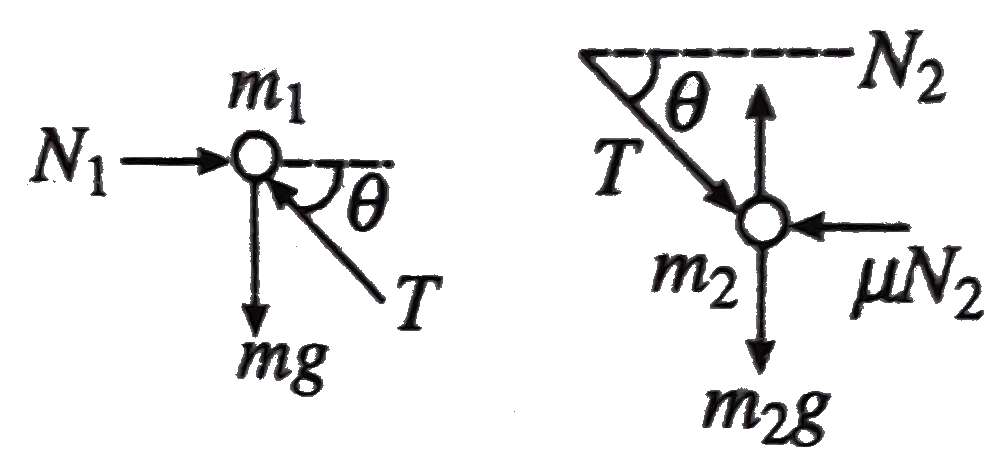
∑Fx=0⇒N1−Tcosθ=0
⇒N1=Tcosθ
∑Fx=0⇒Tsinθ−m1g=0 …(i)
Tsinθ=m2g …(ii)
For the equilibrium of m2
∑Fx=0⇒Tcosθ−μN2=0
⇒Tcosθ=μN2 ....(iii)
∑Fx=0⇒N2−Tsinθ−m2g=0
⇒N2=Tsinθ+m2g ...(iv)
(ii) + (iv) ⇒N2=(m1+m2)g
substituting N2 from (v) in (iii) we obtain
Tcosθ=μ(m1+m2g ....(vi)
(ii) + (vi) tanθ=m1μ(m1+m2)⇒θ=cot−1[μ(1+m1m2)]
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.216videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.327videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Solved Examples12videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer5videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
Similar Questions
Two blocks of mass 'm' and 'M, are connect to the ends of a string passing over a pulley. 'M' lies on the plane inclined at an angle θ with the horizontal and 'm' is hanging vertically as shown in Fig. The coefficient of static friction between 'M' and the incline is μs. Find the minimum and maximum value of 'm' so that the system is at rest.
 View Solution
View SolutionTwo masses m1 and m2 are attached with light strings as shown. If the system is in equilibrium, find the value of θ
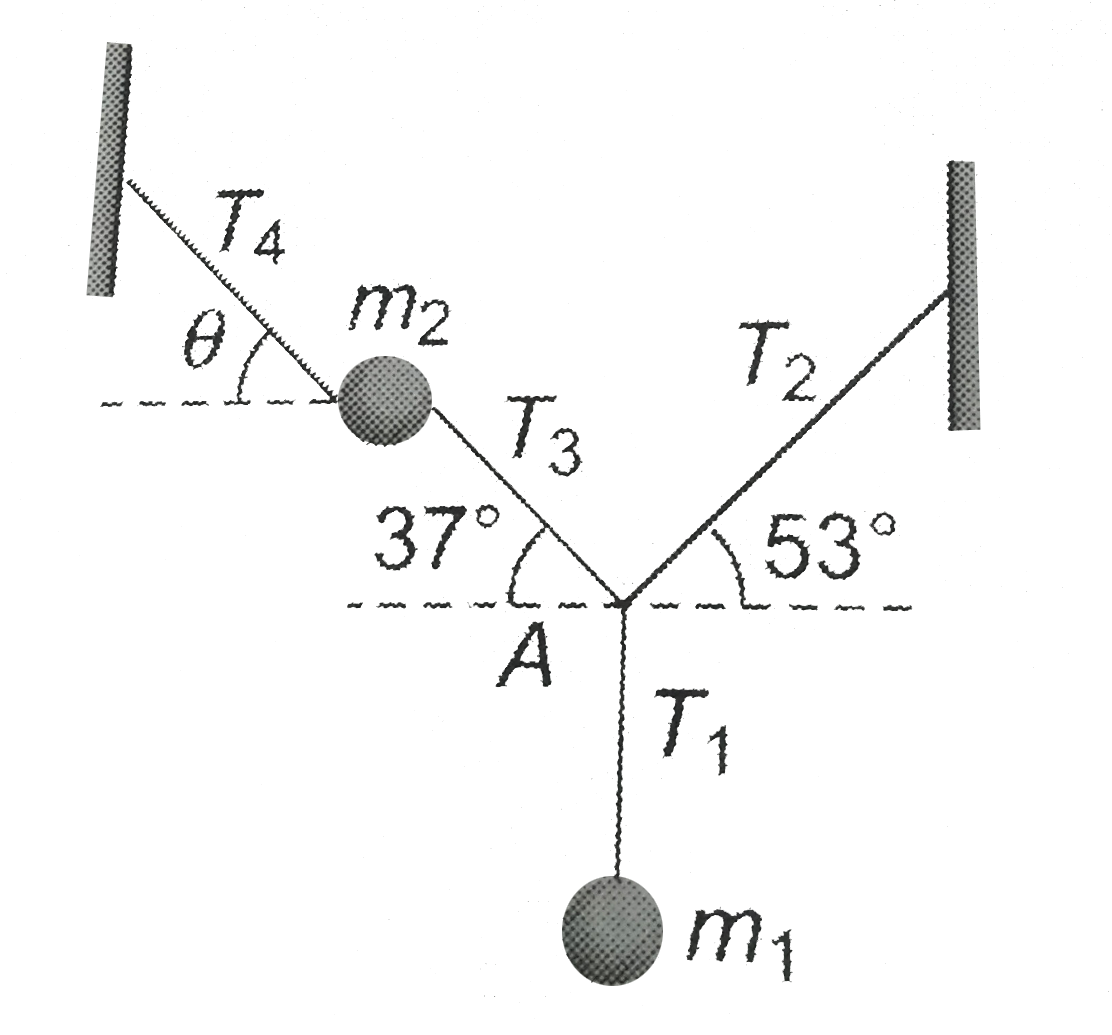 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
In Fig. 7.150 , coefficient of friction between m2 and the horizontal surface is μ , minimum value of m so that the system has zero acceleration , is :
 Aμ(m1+m2)Bμ(m1+m2)Cm2−m1μDm1μ−m2
Aμ(m1+m2)Bμ(m1+m2)Cm2−m1μDm1μ−m2 - Question 2 - Select One
A cart of mass M has a block of mass m in contact with it as shown in the figure-2.133. The coefficient of friction between the block and the cart is μ. What is the minimum acceleration of the cart so that the block m does not fall ?
 AμgBg/μCμ/gDMμg/m
AμgBg/μCμ/gDMμg/m - Question 3 - Select One
A solid sphere of mass m is lying at rest on a rough horizontal surface. The coefficient of friction between the ground and sphere is μ. The maximum value of F, so that the sphere will not slip, is equal to
 A75μmgB47μmgC57μmgD72μmg
A75μmgB47μmgC57μmgD72μmg
If the coefficient of friction between M and the inclined surfaces is μ=1/√3 find the minimum mass m of the rod so that the of mass M=10kg remain stationary on the inclined plane
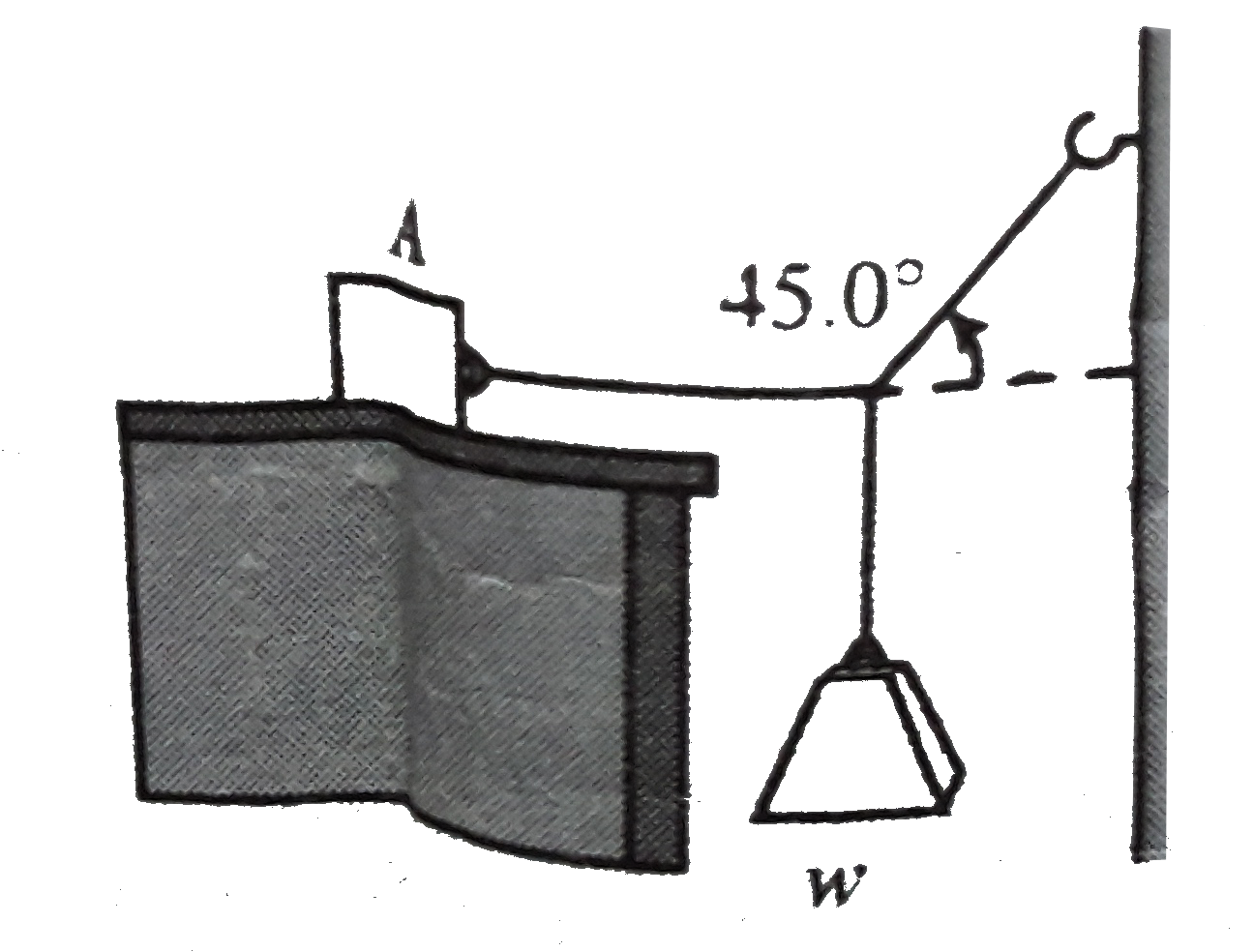 View Solution
View SolutionTwo masses M1andM2 are connected by a light rod and the system is slipping down a rough incline of angle θ with the horizontal. The friction coefficient at both the contacts is μ. Find the acceleration of the system, and the force by the rod on one of the blocks.
View SolutionTwo block of masses M1 and, M2 are connected with a string which passed over a smooth pulley . The mass M1 is placed on a tough inclined plane as shown in figure .The coefficient of friction between and block and the inclined plane is μ what should be the minimum mass M2 so that the block M1 slides upwards?
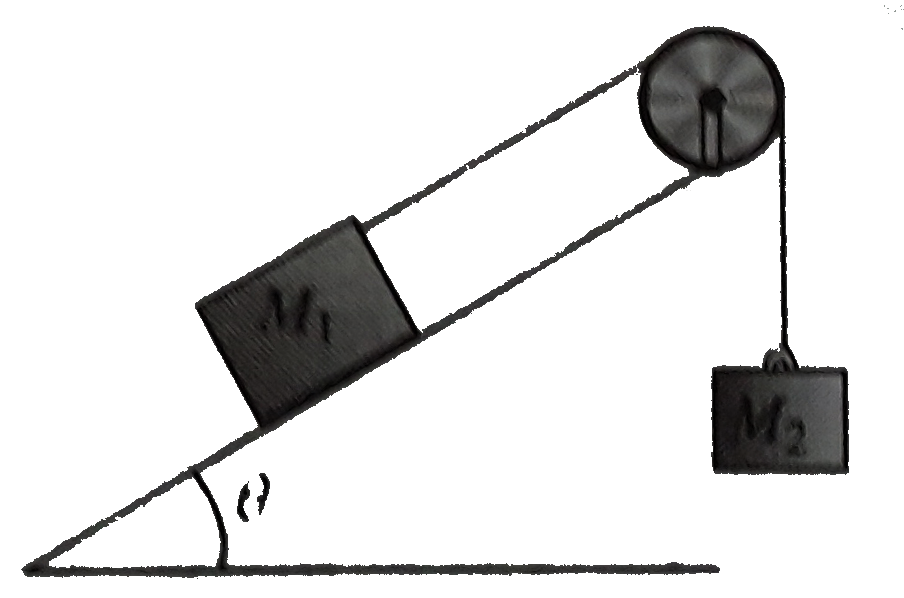 View Solution
View SolutionA solid sphere of mass m is lying at rest on a rough horizontal surface. The coefficient of friction between the ground and sphere is μ. The maximum value of F, so that the sphere will not slip, is equal to
 View Solution
View SolutionA block of mass m is placed on a rough floor of a lift . The coefficient of friction between the block and the floor is μ. When the lift falls freely, the block is pulled horizontally on the floor. What is the force of friction -
View Solution

