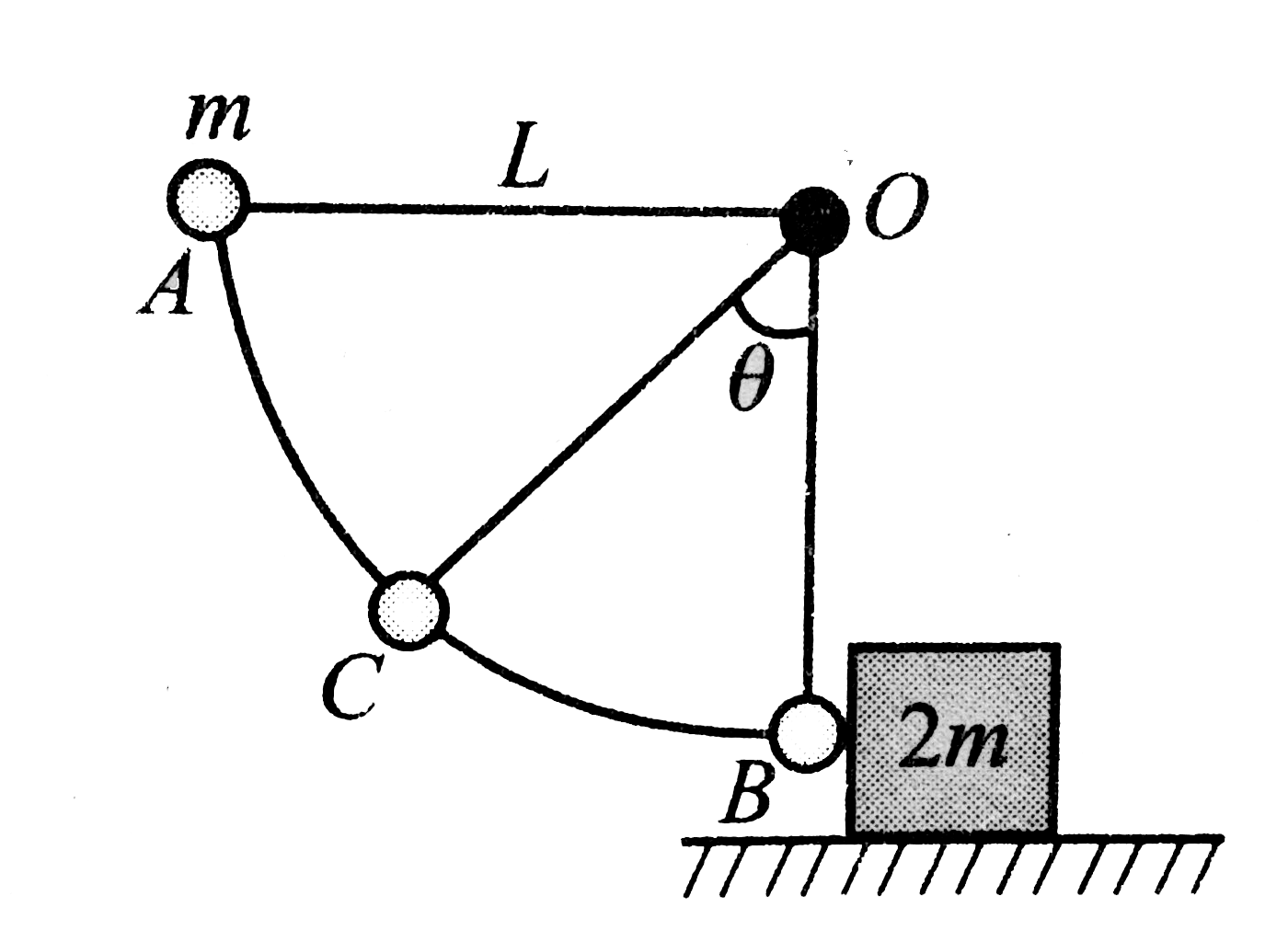
A ball of mass m is attached to a cord of length L, pivoted at point O, as shown in Fig. The ball is released from rest at point A, swings down and makes an inelastic collision with a block of mass 2m kept on a rough horizontal floor. The coefficient of restitution of collision is e=2/3 and coefficient of friction between block and surface is After collision, the ball comes momentarily to rest at C when cord makes an angle of θ with the vertical and block moves a distance of 3L/2 on rough horizontal floor before stopping. The values of μ and θ are, respectively,
50243,cos−1(8081)
5081,cos−1(8081)
281,cos−1(80243)
2243,cos−1(80243)
The correct Answer is:A
Velocity of the ball just before collision v0=√2gL
Applying momentum conservation along horizontal direction (because momentum is conserved in collision along the line of impact), we get
mv0=−mv1+2mv2
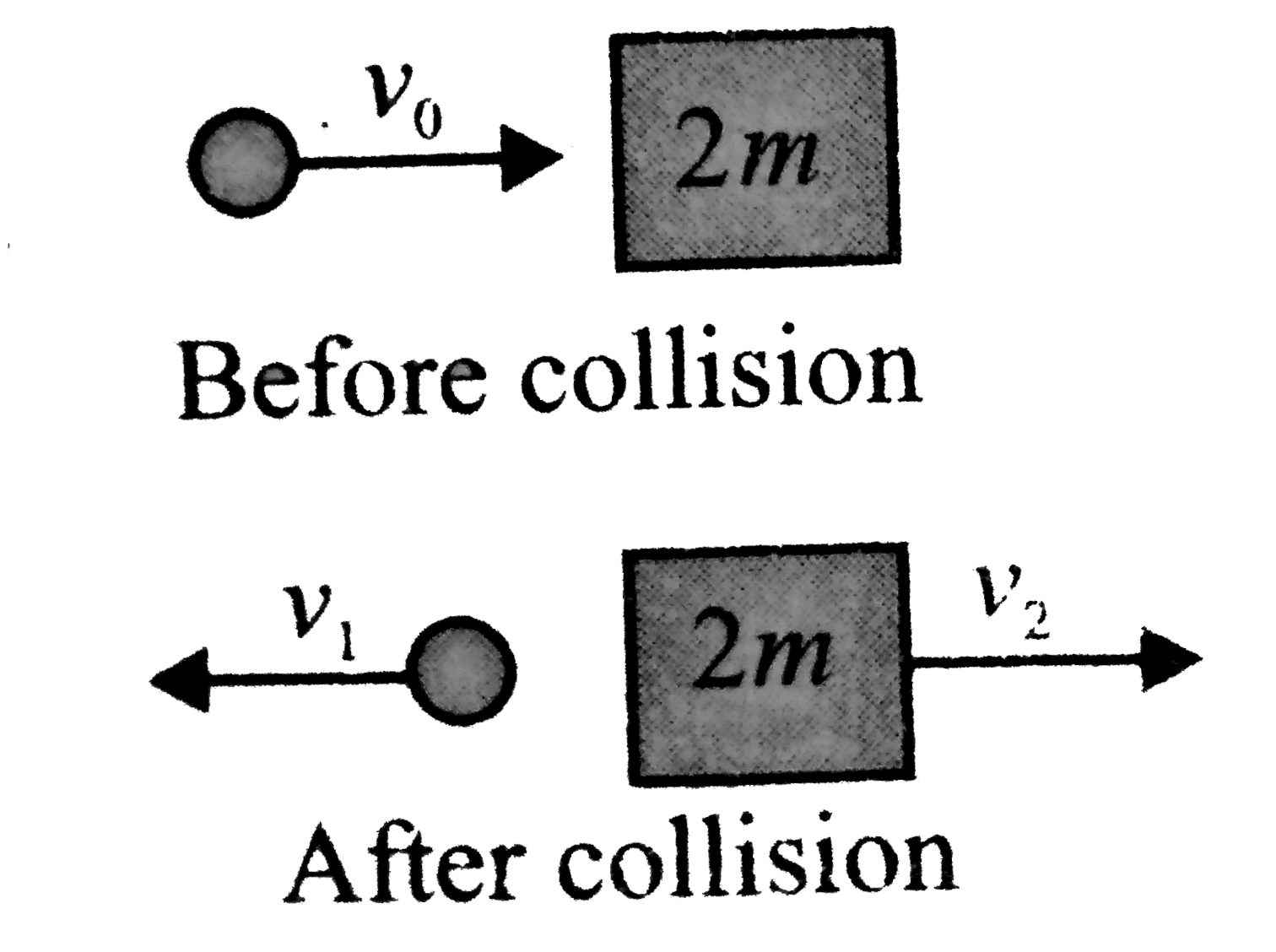
Applying coefficient of restitution equation, we get
e=23=v1+v2v0
Solving the above two equations we get
v1=v09 and v2=5v09
As the block moves a distance of 3L2 before coming to rest, so from work energy theorem,
0−12(2m)v22=−μ×2mg(3L)2⇒v22=3μgL
2581×2gL=3μgLimpliesμ=50243
For ball KE is converted to gravitational potential energy after collision, so
0−mv212=−mg×L(1−cosθ)
cosθ=8081
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Multiple Correct25videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning2videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Subjective23videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example13videos
CALORIMETRY
- BOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer2videos
DIMENSIONS & MEASUREMENT
Similar Questions
A ball strikes a smooth horizontal floor obliquely and rebounds inelastically.
06:02View SolutionStatement I: In a two-body collision, the momenta of the particles are equal and opposite to one another, before as well as after the collision when measured in the centre of mass frame.
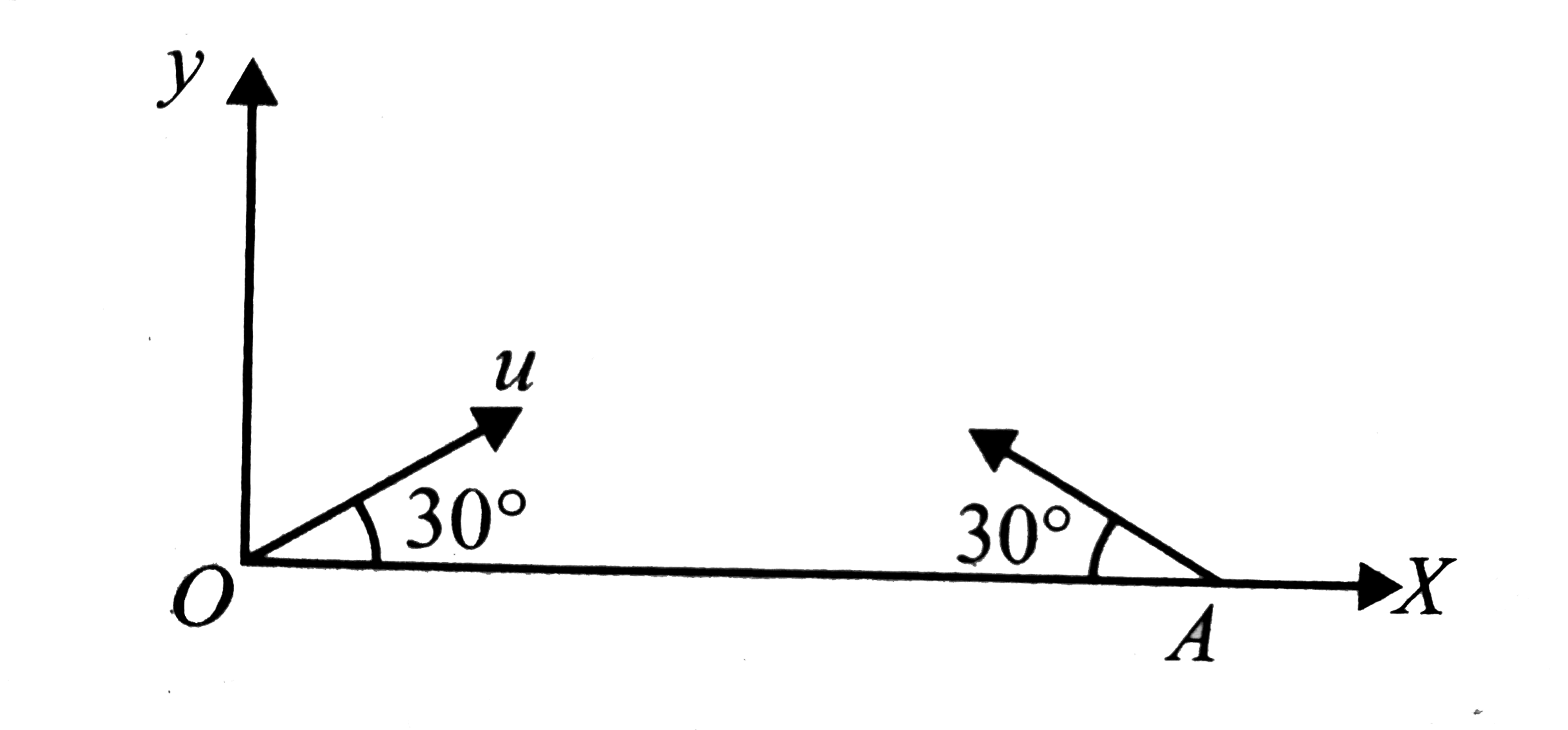
Statement. II: The momentum of the system is zero from the centre of mass frame.02:57View SolutionTwo identical smooth balls are projected from points O and A on the horizontal ground with same speed of projection. The angle of projection in each case is 30∘.The distance between O and A is 100m. The balls collide in mid-air and return to their respective points of projection. If the coefficient of restitution is 0.7, find the speed of projection of either ball (in m/s) correct to nearest integer. (Take g=10ms−2 and √3=1.7)
 04:50View Solution
04:50View SolutionA colorimeter contains 400g of water at a temperature of 5∘C. Then, 200g of water at a temperature of +10∘C and 400g of ice at a temperature of −60∘C are added. What is the final temperature of the contents of calorimeter?
Specific heat capacity of water −1000 cal/kg/K
Specific latent heat of fusion of ice =80×1000 cal/kg
Relative specific heat of ice =0.5View SolutionTo find the distance d over which a signal can be seen clearly in foggy conditions, a railways-engineer uses dimensions and assumes that the distance depends on the mass density ρ of the fog, intensity (power/area) S of the light from the signal and its frequency f. the engineer finds that d is proportional to S1/n. the value of n is
05:16View SolutionA ball of mass m moving at a speed v makes a head on inelastic collision with an identical ball at rest. The kinetic energy of the balls after the collision is 3/4th of the original. Find the coefficient of restitution.
10:06View SolutionA ball of mass m moving at speed v makes a head on collision with an identical ball at rest. The kinetic energy of the balls after the collision is 3//4th of the original. Find the coefficient of restitution.
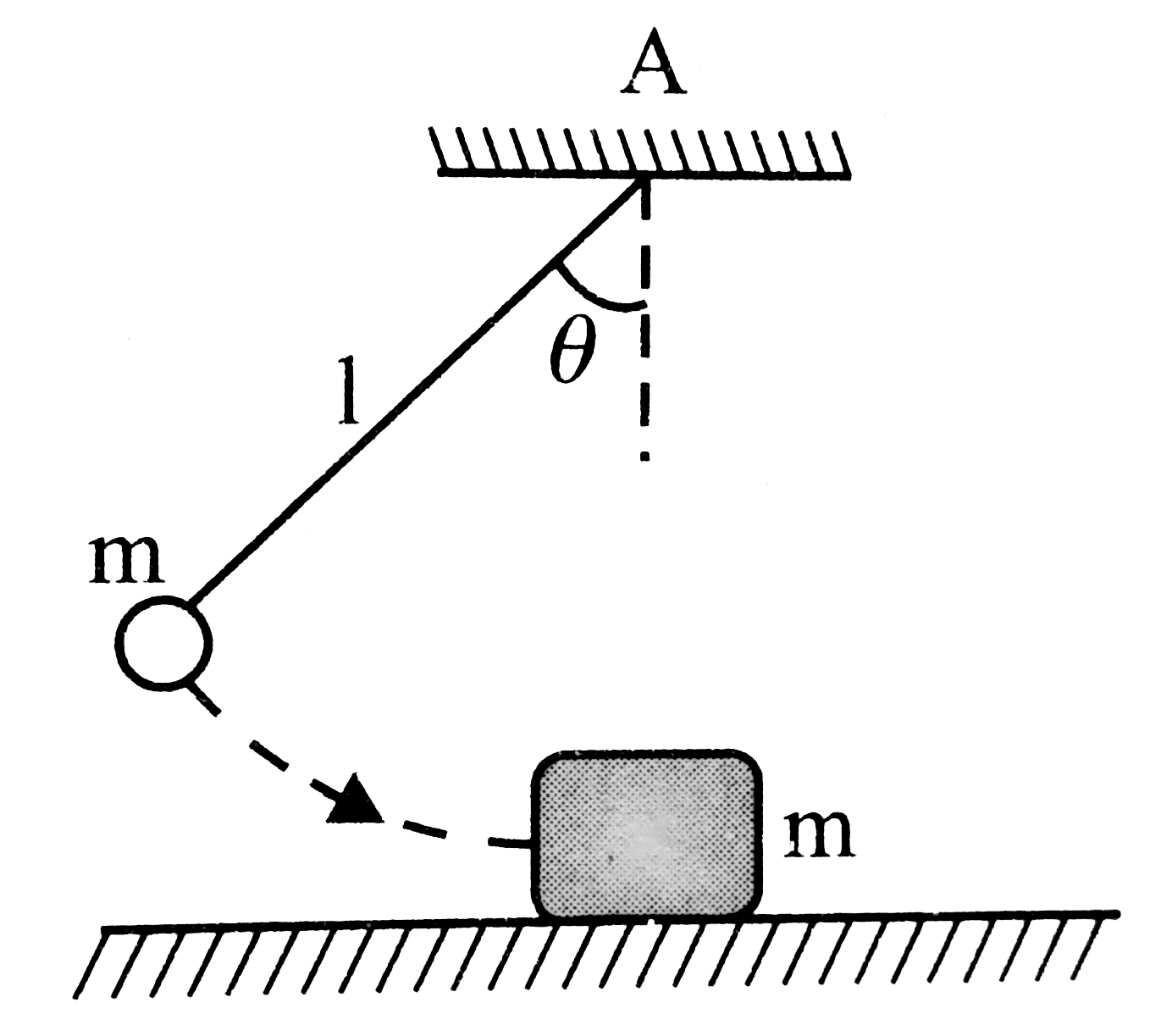
02:33View SolutionIn the arrangement shown in Fig. the ball and the block have the same mass m=1kg each, θ=60∘ and length l=2.50m. Coefficient of friction between the block and the floor is 0.5. When the ball is released from the position shown in Fig. it collides with the block and the block stops after moving a distance 2.50m.
The coefficient of restitution for collision between the ball and the block is02:33View SolutionA ball of mass m falls vertically from a height h and collides with a block of equal mass m moving horizontally with a velecity υ on a surface. The coefficient of kinetic friction between the block and the surface is 0.2, while the coefficient of restitution e between the ball and the block is 0.5. There is no friction acting between the ball and the block. The velocity of the blockdecreases by :
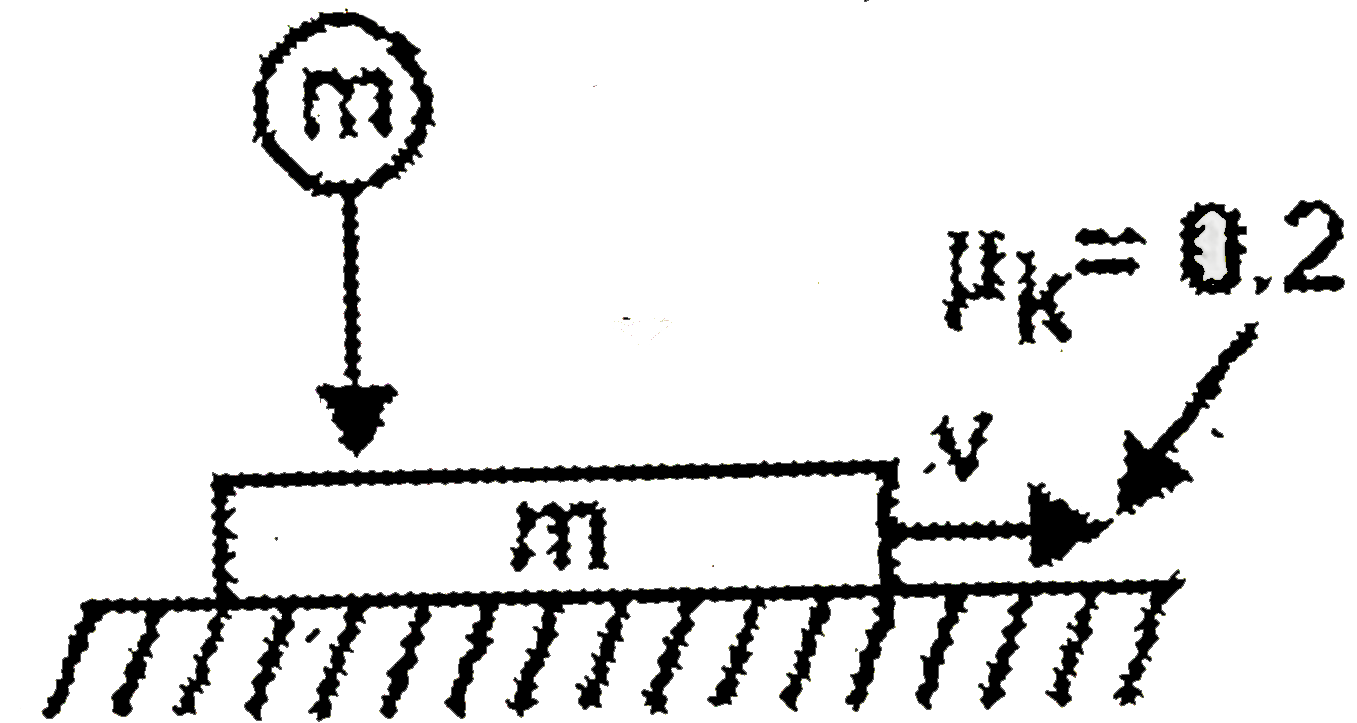 View Solution
View SolutionOn a frictionless surface, a ball of mass m moving at a speed v makes a head on collision with an identical ball at rest. The kinetic energy of the balls after the collision is 3/4th of the original. Find the coefficient of restitution.
View SolutionA ball is dropped from a height h on a floor. The coefficient of restitution for the collision between the ball and the floor is e. The total distance covered by the ball before it comes to the rest.
View SolutionA block of mass m moving with speed v collides with another block of mass 2m at rest. The lighter block comes to rest after the collision. The coefficient of restitution is
01:22View SolutionA block of mass m moving with speed v collides with another block of mass 2m at rest. The lighter block comes to rest after the collision. The coefficient of restitution is
04:01View SolutionA ball of mass 'm' moving at a speed v makes a head on collision with an identical ball at rest. The kinetic energy of balls after the collision is 3/4th of the origial. If e is the coefficient of restitution, then :
09:45View SolutionA block of mass m moving with speed v collides with another block of mass 2m at rest. The lighter block comes to rest after the collision. The coefficient of restitution is
05:23View Solution

