Two identical blocks A and B each of mass 2kg are hanging stationary by a light inextensible flexible string, passing over a light and frictionless pulley, as shown in Fig. A shell C of mass 1kg moving vertically upwards with velocity 9m/s collides with block B and gets stuck to it.
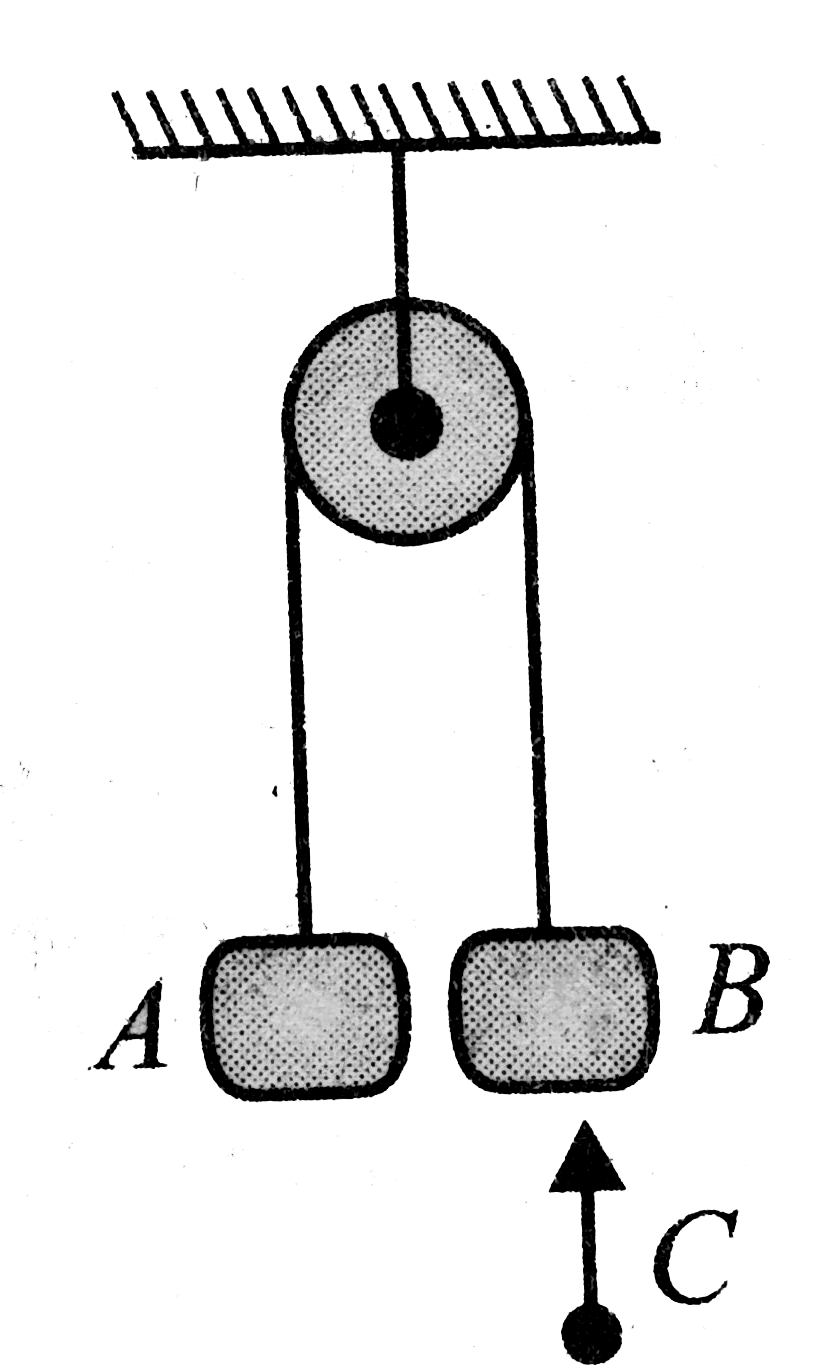
Calculate the time after which block B starts moving downwards.
0.90s
1s
0.60s
0.30s
The correct Answer is:A
When C strikes with B, the combined body starts to rise vertically upward. According to law of conservation of momentum, velocity v0 of combined body (just after collision) is given by
(2+1)v0=1×9 or v0=3m/s
But A is at rest. Therefore, string becomes slack and bodies move under gravity, combined body upwards and A downwards till string again becomes taut. This happens when downward displacement of A becomes equal to upward displacement of combined body. Let it happen at instant t1. Then,
12gt21=3t1−12gt21
or t1=0.3s Displacement of each block at that instant,
h1=12gt210.45m
And velocity of combined body is 3−gt1=0 and velocity of A is gt1=3m/s (downward).
Since at this instant, velocities are different, therefore an impulse is developed in the string and magnitude of velocities of bodies becomes equal. Let that velocity magnitude be v and impulse developed be J. This impulse acts upwards on both the bodies.
Hence for A
2×3−J=v’………i
and for combined body,
J=3v’…………..ii
From above equations
v’=1.2m/s
Now combined body starts to move upwards with velocity v’=1.2m/s and retardation a, where
a=3−23+2=2m/s2
Combined body comes to an instantaneous rest after time
t2=v′a=0.6s
After this instant, combined body starts moving down. Time interval between this instant and instant of collision is given by
t1+t2=0.3+0.6=0.9s
Further height ascended by combined body during time t2 is
h2=v’v2−12at22=0.36m
Maximum height raised by combined body is
h=h1+h2=0.81m
Loss of energy during collision of B and C is
E1=12×1×92−123v20=27J
Loss of energy when impulse is developed in string is
E2=12×2x32−12(2+3)3v20(2+3)(v’)2=5.4J
Loss of mechanical energy till B reaches highest point is
E=E1+E2=27+5.4=32.4J
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Integer
16 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Fill In The Blanks
2 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning
2 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICSCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICSCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
A bullet is fired on a fixed target. It penetrates inside the target through distance d=3.75cm and then stops. mass of the bullet is m=1kg and of the target is M=4kg. Now an identical bullet moving with the same velocity is fired on the identical target which is placed at rest on a frictionless horizontal surface. Then find the distance (in cm) to which the bullet will penetrate inside the target?
View SolutionA particle of mass 4 m which is at rest explodes into three fragments. Two of the fragments each of mass m are found to move with a speed v each in mutually perpendicular directions. The total energy released in the process of explosion is ............
View SolutionStatement I: In a two-body collision, the momenta of the particles are equal and opposite to one another, before as well as after the collision when measured in the centre of mass frame.
Statement. II: The momentum of the system is zero from the centre of mass frame.
View SolutionA colorimeter contains 400g of water at a temperature of 5∘C. Then, 200g of water at a temperature of +10∘C and 400g of ice at a temperature of −60∘C are added. What is the final temperature of the contents of calorimeter?
Specific heat capacity of water −1000 cal/kg/K
Specific latent heat of fusion of ice =80×1000 cal/kg
Relative specific heat of ice =0.5
View SolutionTo find the distance d over which a signal can be seen clearly in foggy conditions, a railways-engineer uses dimensions and assumes that the distance depends on the mass density ρ of the fog, intensity (power/area) S of the light from the signal and its frequency f. the engineer finds that d is proportional to S1/n. the value of n is
View SolutionFor the system shown in Fig. the string is light and pulley is frictionless. The 4kg block is given an upward velocity of 1m/s. The centre of mass of the two blocks will [neglect the impulse duration]
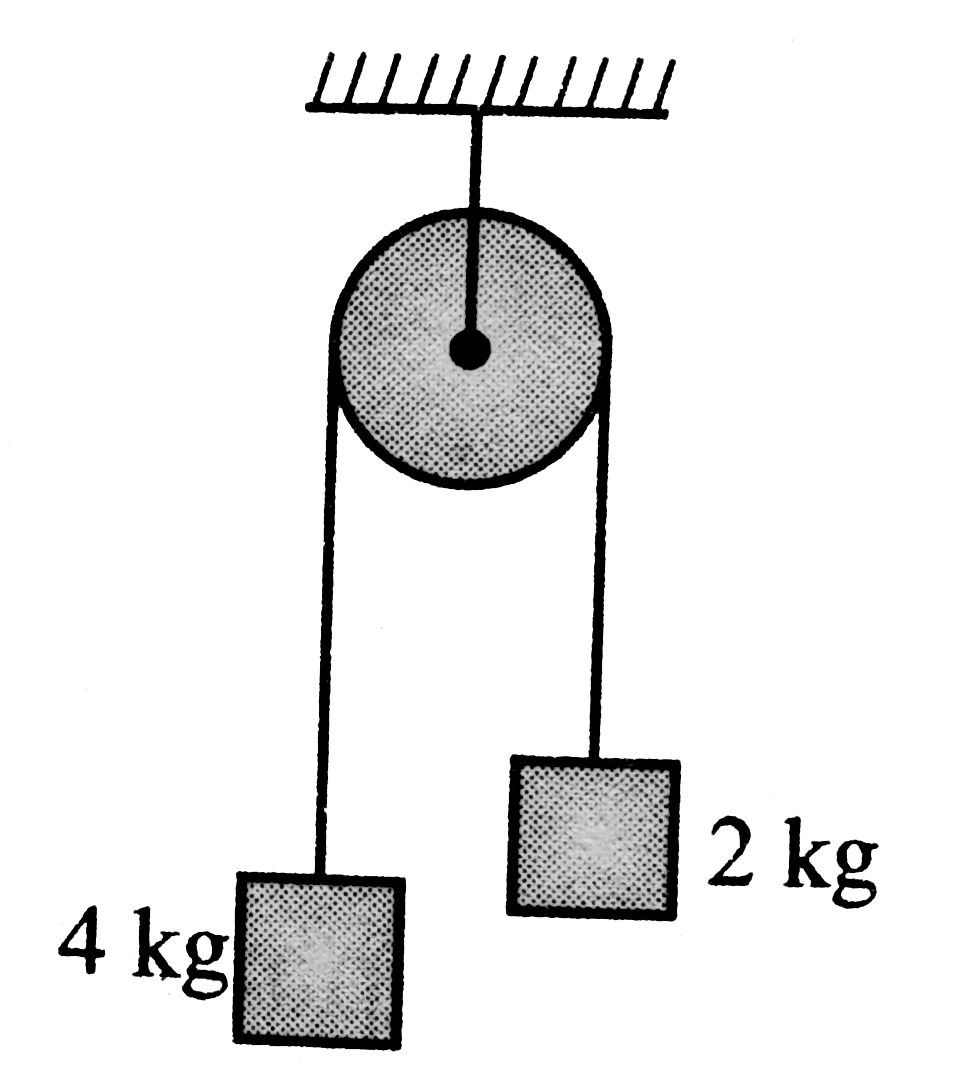
View SolutionTwo identical blocks A and B each of mass 2kg are hanging stationary by a light inextensible flexible string, passing over a light and frictionless pulley, as shown in Fig. A shell C of mass 1kg moving vertically upwards with velocity 9m/s collides with block B and gets stuck to it.
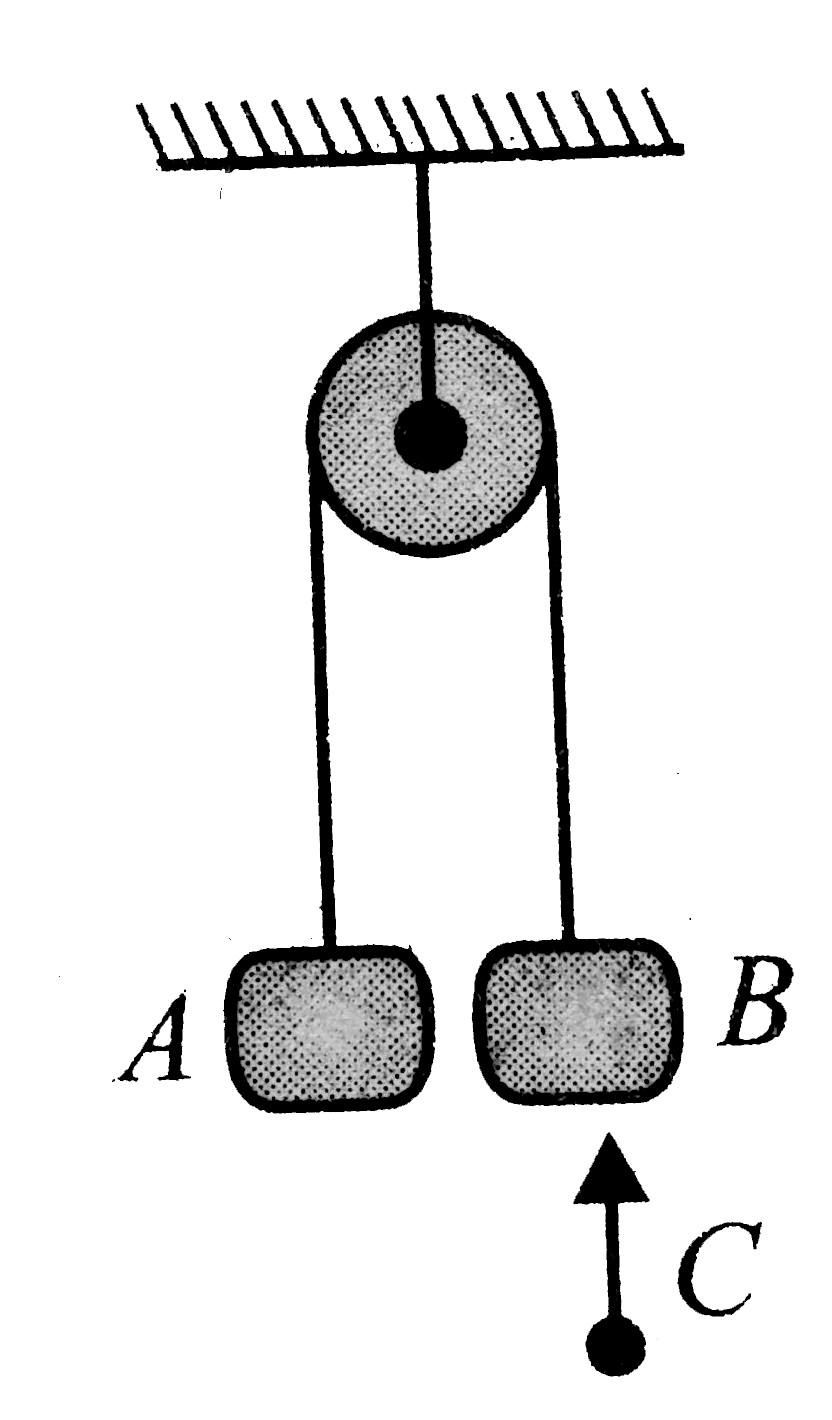
The maximum height reached by B
View SolutionTwo identical blocks A and B each of mass 2 kg are hanging stationary by a light inextensible flexible string, passing over a light and frictionless pulley, as shown in Fig. A shell C of mass 1 kg moving vertically upwards with velocity 9 m//s collides with block B and gets stuck to it. The loss of mechanical energy up to that instant is
View SolutionTwo blocks A and B , each of mass 10 kg , are connected by a light string passing over a smooth pulley as shown in the figure. Block A will remain at rest if the friction on it is
View SolutionA light in extensible thread passes over a small frictionless pully. Two blocks of masses m=kg and M=3kg respectively are attached with the thread as shown in the fig. The heavier block rests on a horizontal surface. A shell of mass 1kg moving upward with a velocity 10m/s collides and sticks with the block of mass 'm' as shown in the fig at t=0. If the long inclined plane is smooth.
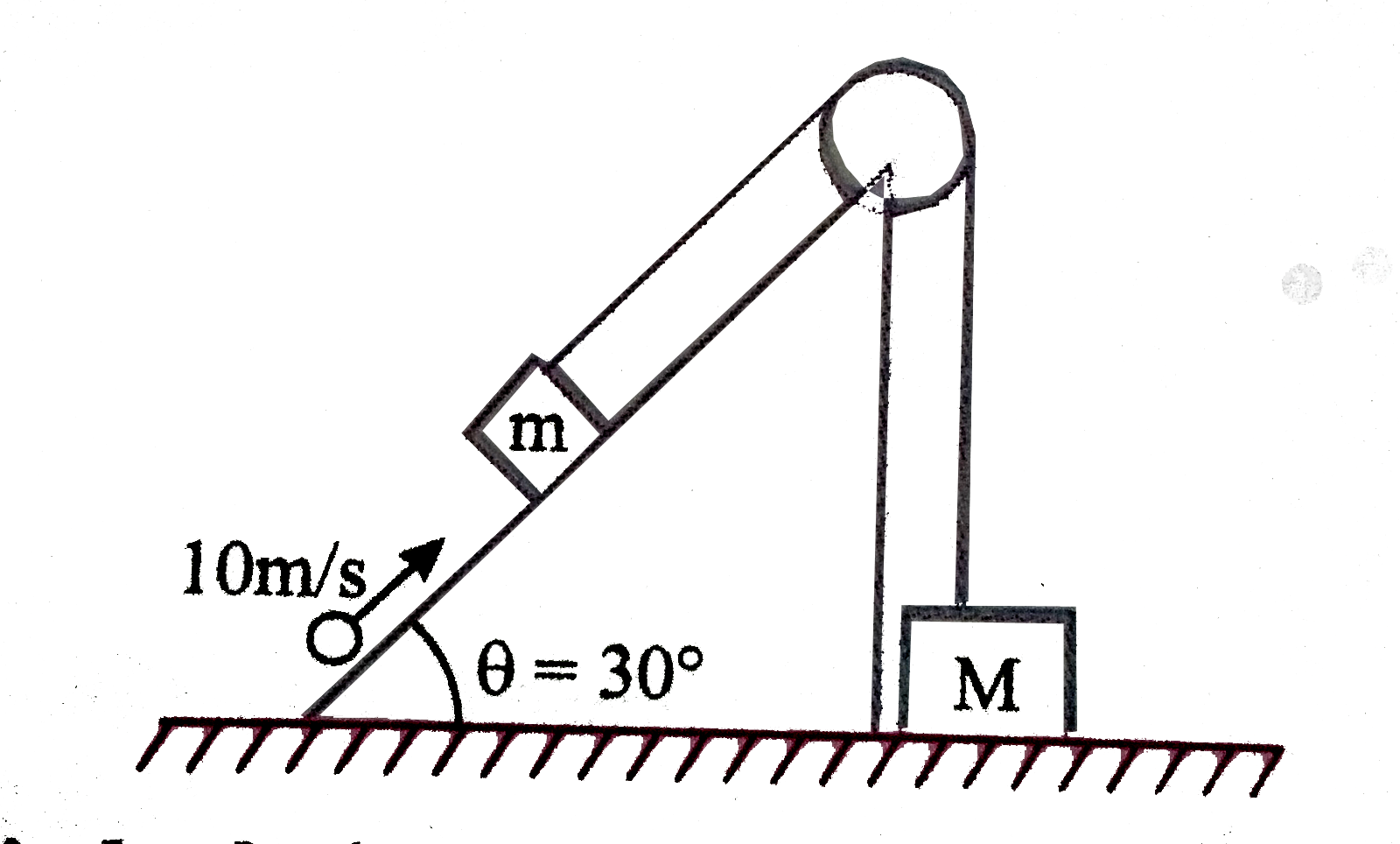
Find velocity of (m+shell) just after collision.
View SolutionThree blocks A,B and C of mass 10 kg each are hanging on a string passing over a fixed frictionless pulley as shown in figure. The tension in the string connecting blocks B and C is (g=10m//s^(2))
View SolutionTwo blocks of masses m and 3m are connected by an inextensible string and the string passes over a fixed pulley which is massless and frictionless. A bullet of mass m moving with a velocity 'u' hits the hanging block of mass 'm' and gets exbedded in it. Find the height through which block A rises after the collision.
View SolutionTwo identical blocks A and B each of mass 2 kg hanging stationsary by a light inextensible flexible string passing over a light and frictionless pulley. A shot C, of mass 1 kg moving vertically with velocity 9 m/s collides with block B and get stuck to it. Calculate : Time after which block B starts moving downward Maximum height reached by B Loss of mechanical energy upto that instant
View SolutionTwo identical masses are attached by a light string that passes over a small pulley, as shown . The table and the pulley are frictionless. The hanging block is moving

View SolutionA block of mass m_(1)=2 kg is kept on a smooth horizontal table. Another block of mass m_(2)=1 kg is connected to m_(1) by a light inextensible string passing over a smooth pulley as shown in the figure. If the blocks are released, what will be the (a) acceleration of each block (b) tension in the string?
View Solution
