An L shaped uniform rod of mass 2M and length 2L(AB=BC=L) is held as shown in Fig. with a string fixed between C and wall so that AB is vertical and BC is horizontal. There is no friction between the hinge and the rod at A.
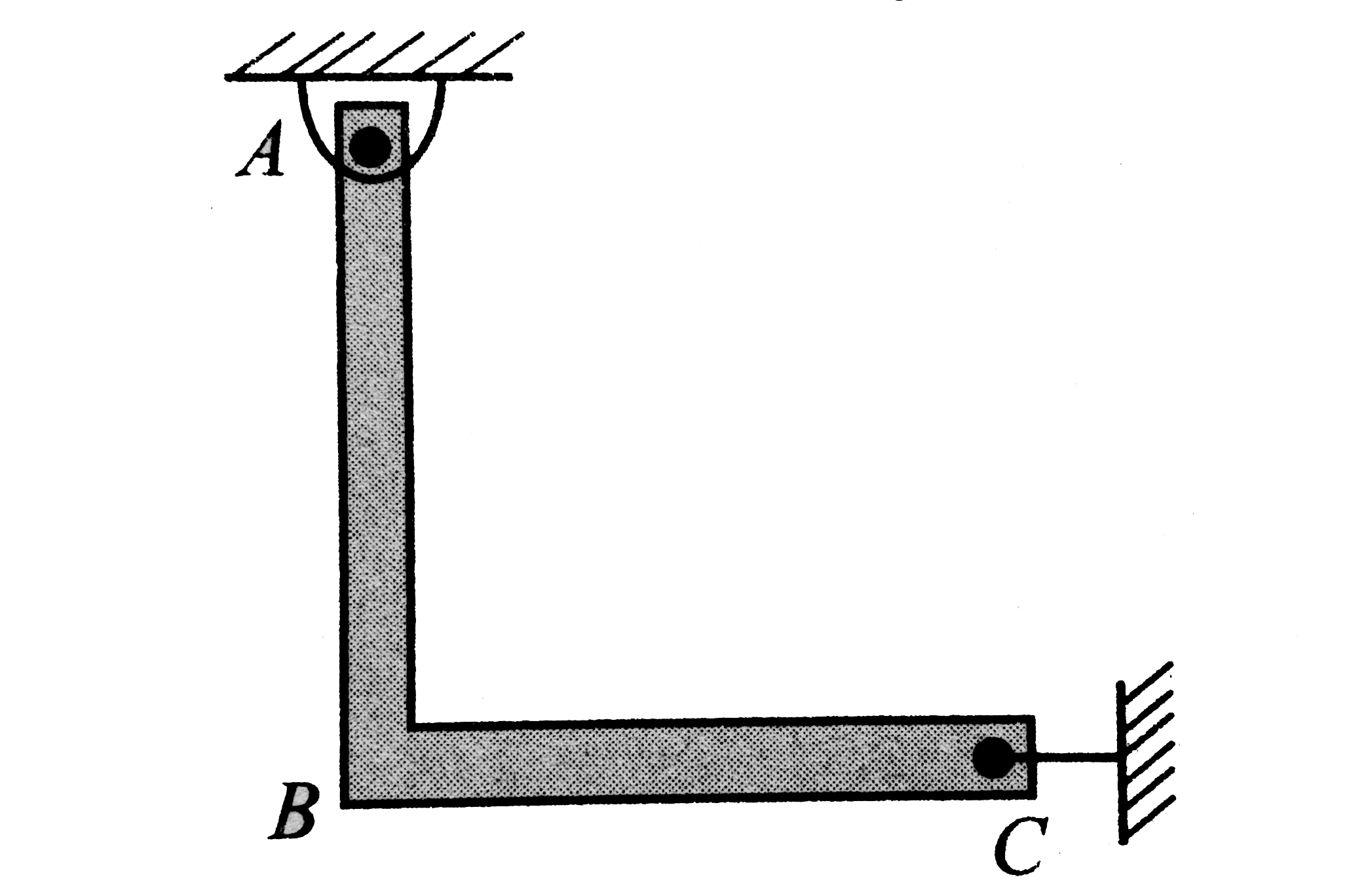
If the string is burnt, find the angle between AB and the vertical at equilibrium position.
tan−1(13)
tan−1(14)
tan−1(3)
tan−(−1)(12)
The correct Answer is:A
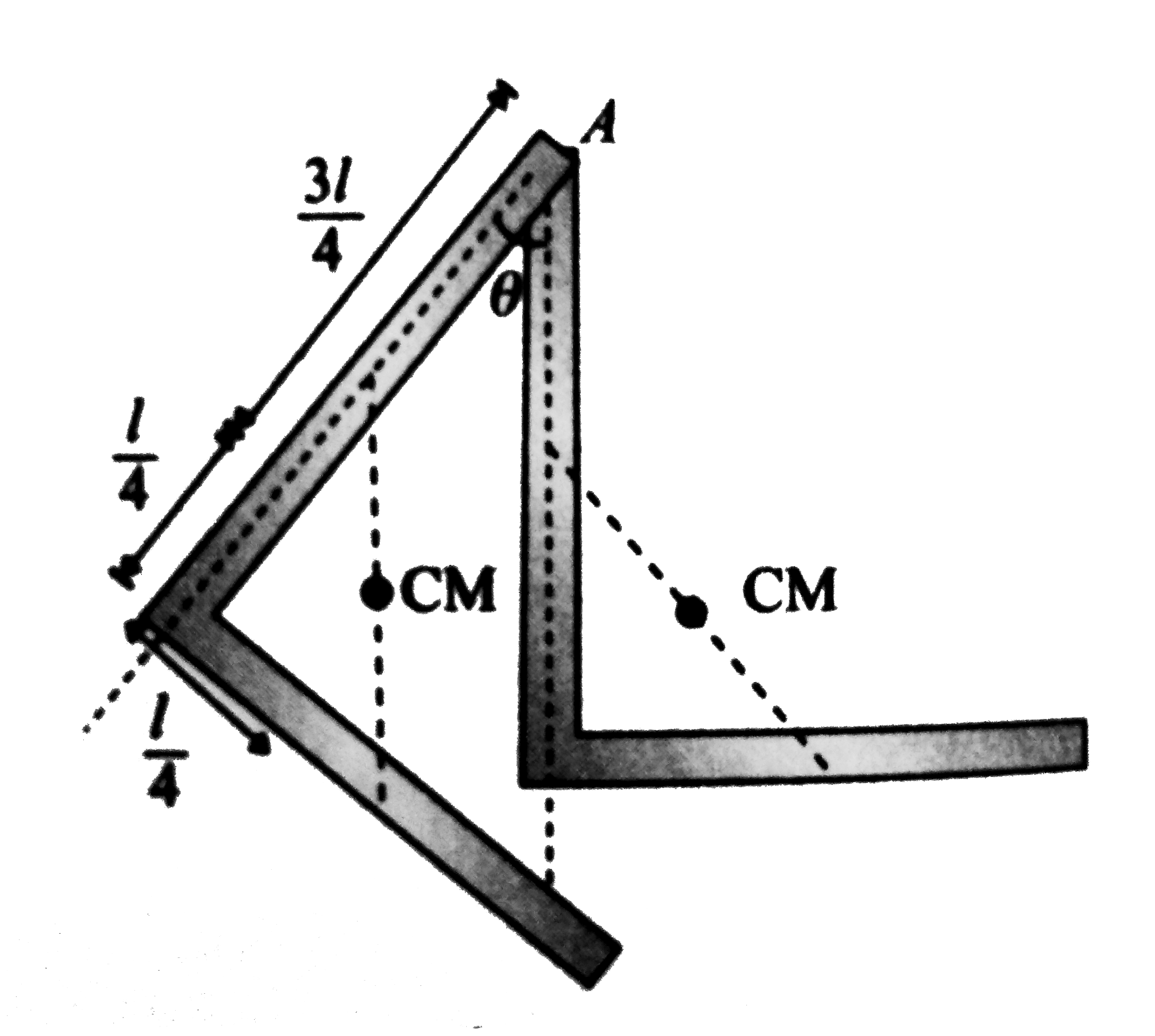
D is the CM of the rod
In triangle ADE:
tanθ=L/43L/4
⇒tanθ=13⇒θ=tan−1(1/3)
here D is the centre of mass. In equilibrim, D will be vertically below A. so i equilibrium AB will make angle θ as calculated above with the vertical.
Topper's Solved these Questions
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Multiple Correct
9 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger
2 Videos
Similar Questions
An L shaped uniform rod of mass 2M and length 2L(AB=BC=L) is held as shown in Fig. with a string fixed between C and wall so that AB is vertical and BC is horizontal. There is no friction between the hinge and the rod at A.
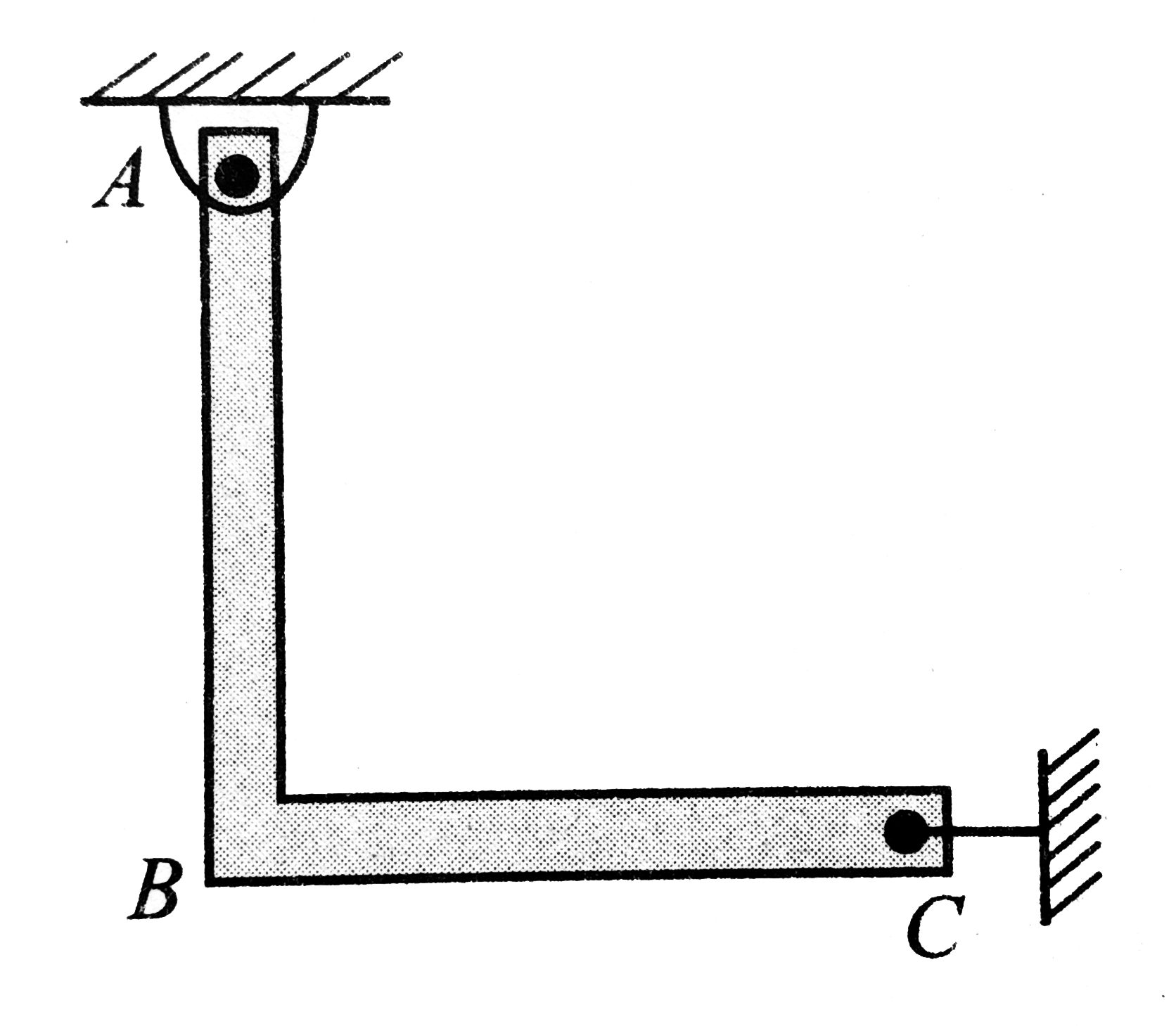
Find the tension in the string
View SolutionAn L shaped uniform rod of mass 2M and length 2L(AB=BC=L) is held as shown in Fig. with a string fixed between C and wall so that AB is vertical and BC is horizontal. There is no friction between the hinge and the rod at A.
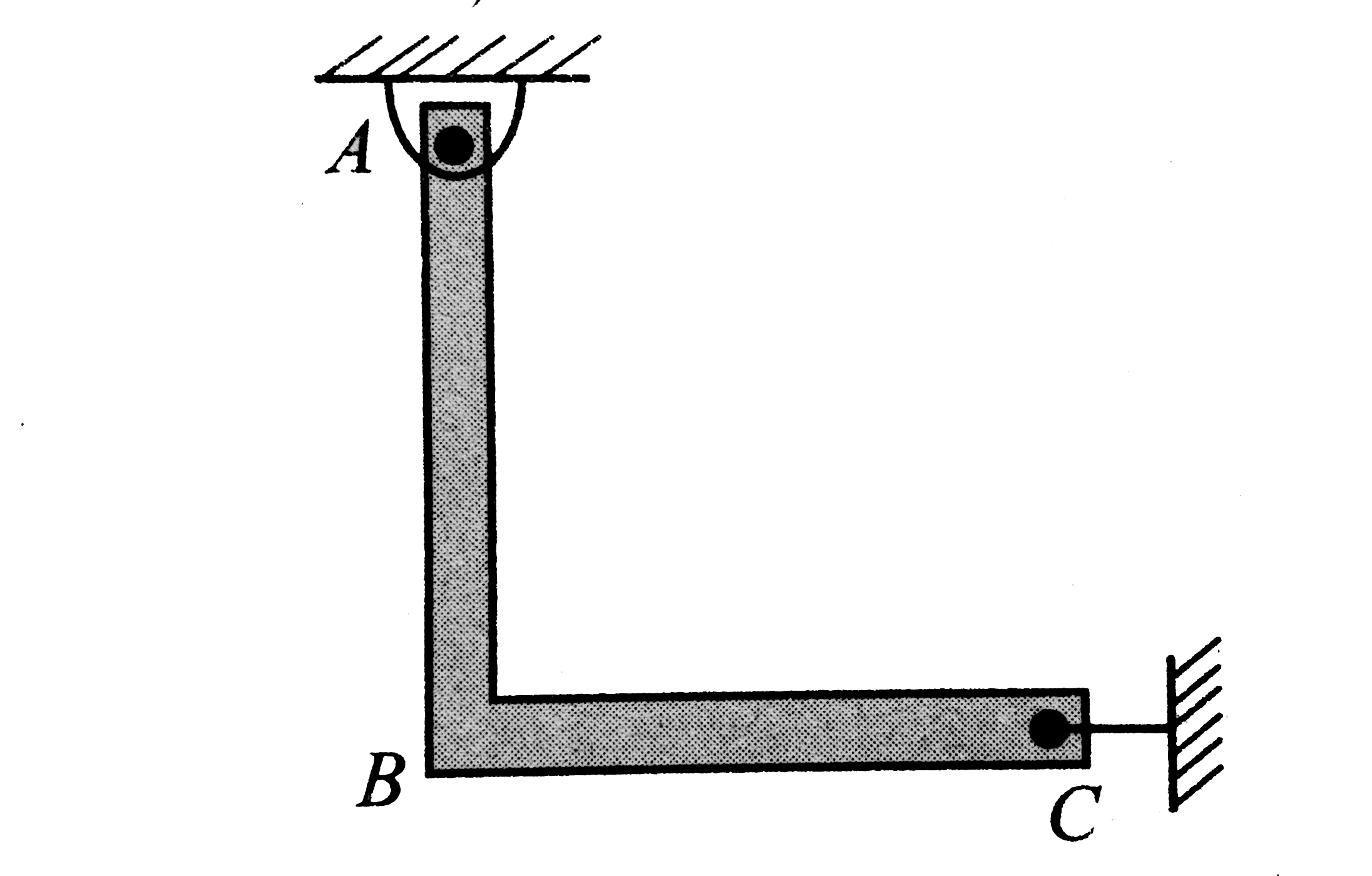 What will be the reaction between hinge and rod at point A?
What will be the reaction between hinge and rod at point A?
View Solution
Knowledge Check
An L shaped uniform rod of mass 2M and length 2L(AB=BC=L) is held as shown in Fig. with a string fixed between C and wall so that AB is vertical and BC is horizontal. There is no friction between the hinge and the rod at A.

Find the tension in the stringAMg3BMg4CMgDMg2An L shaped uniform rod of mass 2M and length 2L(AB=BC=L) is held as shown in Fig. with a string fixed between C and wall so that AB is vertical and BC is horizontal. There is no friction between the hinge and the rod at A.
 What will be the reaction between hinge and rod at point A? A√65Mg4B2MgC√17Mg4D√17Mg2
What will be the reaction between hinge and rod at point A? A√65Mg4B2MgC√17Mg4D√17Mg2A uniform L-shaped rod of mass 2m and length 2L, hangs from a hinge as shown (AO is horizontal and OB is vertical). If it is released from the position shown, then find the maximum angular velocity acquired by the end.
A√3glB√3gl(√2−1)C√3g2l(√2−1)D√3g2l(√2+1)
A uniform rod of mass M and length L is hinged at its end. The rod is released from its vertical position by slightly pushing it. What is the reaction at the hinge when the rod becomes horizontal, again vertical.
View SolutionA uniform rod of mass m and length L is suspended with two massless strings as shown in the figure. If the rod is at rest in a horizontal position the ratio of tension in the two strings T1T2 is:

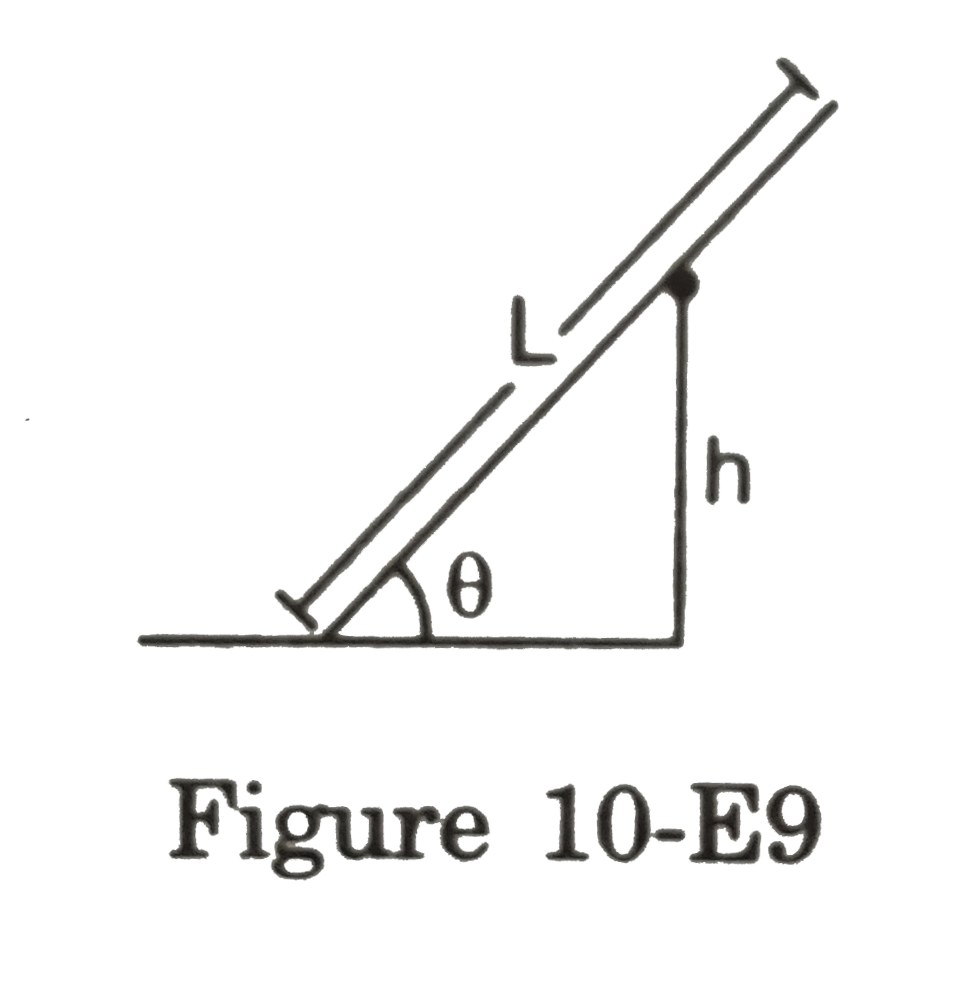
View SolutionA uniform rod of length L rests against a smooth roller as shown in figure. Find the friction coefficient between the ground and the lower end if the minimum angle that rod can make with the horizontal is θ.
View SolutionA conical pendulum, a thin uniform rod of length l and mass m, rotates uniformly about a vertical axis with angular velocity ω(the upper end of the rod is hinged). Find the angle θ between the rod and the vertical.
View SolutionA uniform rod of mass m and length L is suspended with two massless strings as shown in the figure. If the rod is at rest in a horizontal position the ratio of tension in the two strings T1T2 is:

View Solution
