A uniform ladder 5.0m long rests against a frictionless, vertical wall with its lower end 3.0m to from the wall. The ladder weighs 160N. The coefficient of static friction between the foot of the ladder and the ground is 0.40. A man weighing 740N climbs slowly up the ladder.
How far along the ladder can the man climb before the ladder starts to slip?
3m
5m
2.7m
1.25m
The correct Answer is:A
To solve the problem step by step, we will analyze the forces and torques acting on the ladder and find out how far the man can climb before the ladder starts to slip.
Step 1: Understand the Setup
- The ladder is 5.0 m long and rests against a frictionless wall.
- The base of the ladder is 3.0 m away from the wall.
- The weight of the ladder is 160 N, and the man weighs 740 N.
- The coefficient of static friction between the ladder and the ground is 0.40.
Step 2: Calculate the Angle of the Ladder
Using the geometry of the situation:
- The height of the wall where the ladder touches can be found using the Pythagorean theorem:
h=√(5.02)−(3.02)=√25−9=√16=4.0 m
- The angle θ can be calculated using:
tan(θ)=h3.0=4.03.0
Thus, θ=tan−1(43).
Step 3: Calculate the Maximum Static Friction Force
The normal force N at the base of the ladder can be calculated by balancing the vertical forces:
- The total weight acting downwards is the weight of the ladder plus the weight of the man:
N=160N+740N=900N
- The maximum static friction force fmax is given by:
fmax=μs⋅N=0.40⋅900N=360N
Step 4: Establish Torque Equilibrium
To find how far the man can climb before the ladder starts to slip, we need to set up a torque balance about the point where the ladder touches the ground.
Assuming the man climbs a distance x up the ladder:
- The torque due to the man’s weight (740 N) about the base is:
τman=740⋅x⋅cos(θ)
- The torque due to the weight of the ladder (acting at its center, which is at 2.5 m) is:
τladder=160⋅2.5⋅cos(θ)
- The torque due to the friction force (360 N) is:
τfriction=360⋅3.0⋅sin(θ)
Step 5: Set Up the Torque Equation
Setting the sum of torques about the base to zero for equilibrium:
740⋅x⋅cos(θ)+160⋅2.5⋅cos(θ)=360⋅3.0⋅sin(θ)
Step 6: Solve for x
Rearranging the equation to solve for x:
x=360⋅3.0⋅sin(θ)−160⋅2.5⋅cos(θ)740⋅cos(θ)
Step 7: Substitute Values
Using sin(θ)=45 and cos(θ)=35:
x=360⋅3.0⋅45−160⋅2.5⋅35740⋅35
Calculating the values:
x=360⋅3.0⋅0.8−160⋅2.5⋅0.6740⋅0.6
x=864−240444≈1.41m
Final Answer
The man can climb approximately 1.41 m along the ladder before it starts to slip.
- The ladder is 5.0 m long and rests against a frictionless wall.
- The base of the ladder is 3.0 m away from the wall.
- The weight of the ladder is 160 N, and the man weighs 740 N.
- The coefficient of static friction between the ladder and the ground is 0.40.
Using the geometry of the situation:
- The height of the wall where the ladder touches can be found using the Pythagorean theorem:
h=√(5.02)−(3.02)=√25−9=√16=4.0 m
- The angle θ can be calculated using:
tan(θ)=h3.0=4.03.0
Thus, θ=tan−1(43).
The normal force N at the base of the ladder can be calculated by balancing the vertical forces:
- The total weight acting downwards is the weight of the ladder plus the weight of the man:
N=160N+740N=900N
- The maximum static friction force fmax is given by:
fmax=μs⋅N=0.40⋅900N=360N
To find how far the man can climb before the ladder starts to slip, we need to set up a torque balance about the point where the ladder touches the ground.
- The torque due to the man’s weight (740 N) about the base is:
τman=740⋅x⋅cos(θ)
- The torque due to the weight of the ladder (acting at its center, which is at 2.5 m) is:
τladder=160⋅2.5⋅cos(θ)
- The torque due to the friction force (360 N) is:
τfriction=360⋅3.0⋅sin(θ)
Setting the sum of torques about the base to zero for equilibrium:
740⋅x⋅cos(θ)+160⋅2.5⋅cos(θ)=360⋅3.0⋅sin(θ)
Rearranging the equation to solve for x:
x=360⋅3.0⋅sin(θ)−160⋅2.5⋅cos(θ)740⋅cos(θ)
Using sin(θ)=45 and cos(θ)=35:
x=360⋅3.0⋅45−160⋅2.5⋅35740⋅35
x=360⋅3.0⋅0.8−160⋅2.5⋅0.6740⋅0.6
x=864−240444≈1.41m
The man can climb approximately 1.41 m along the ladder before it starts to slip.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer11videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Multiple Correct9videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger2videos
RIGID BODY DYNAMICS 2
Similar Questions
A ladder of length 50 m rests against a vertical wall, at a height of 30 m from the ground. Find the inclination of the ladder with the horizontal. Also find the distance between the foot of the ladder and the wall.
View SolutionA uniform ladder of length 3.25 m and weight 250 N is placed against a smooth vertical wall with its lower end 1.25 m from the wall. If coefficient of friction between the ladder and the floor is 0.3, then, find the frictional force acting on the ladder at the point of contact between ladder and floor.
View Solution
Knowledge Check
- Question 1 - Select One
A uniform ladder 5.0m long rests against a frictionless, vertical wall with its lower end 3.0m to from the wall. The ladder weighs 160N. The coefficient of static friction between the foot of the ladder and the ground is 0.40. A man weighing 740N climbs slowly up the ladder.
What is the actual frictional force when the man has climbed 1.0m along the ladder?A360NB171NC900ND740N - Question 2 - Select One
A uniform ladder 5.0m long rests against a frictionless, vertical wall with its lower end 3.0m to from the wall. The ladder weighs 160N. The coefficient of static friction between the foot of the ladder and the ground is 0.40. A man weighing 740N climbs slowly up the ladder.
What is the maximum frictional force that the ground can exert on the ladder at its lower end?A360NB171NC900ND740N - Question 3 - Select One
A ladder, 10 metre long rests with one end against a smooth vertical wall and the other end on the ground which is rough, the coefficient of friction being 12. The foot of the ladder being 2 metre from the wall. A amn whose weight is 4 times that of the ladder can ascend before it be givens to slip is
A34(10√6−1)B54(10√6−1)C23(5√2−1)DNone
A uniform ladder of length l rests against a smooth, vertical wall (figure). If the mass of the ladder is m and the the coefficient of static friction between the ladder and the ground is μs=0.375 and the minimum angle θmin at which the ladder does not slip.
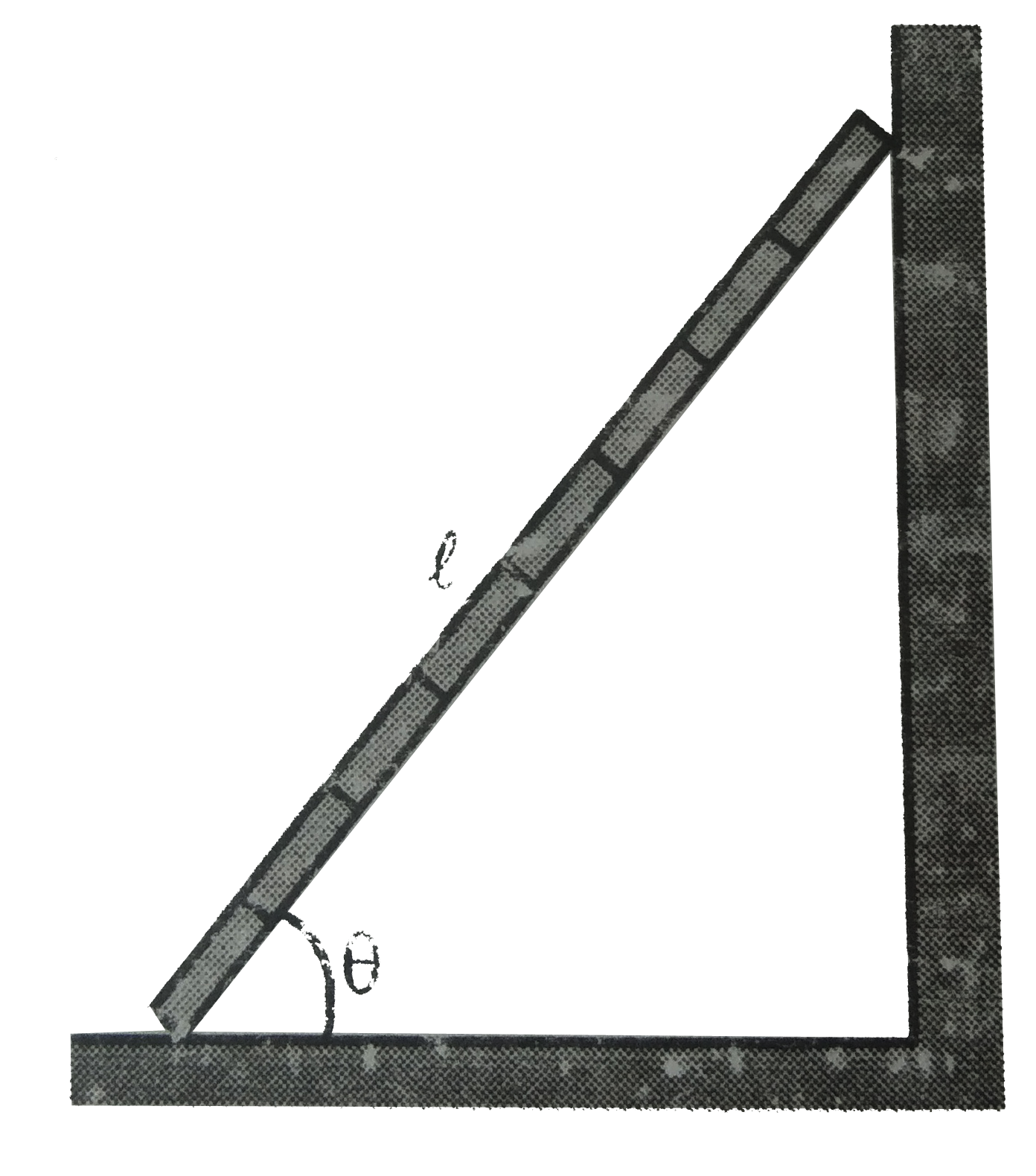
A uniform ladder at rest, leading against a smooth wall. Th ground is rough.View SolutionA ladder 10m long rests against a vertical wall. If the foot of the ladder is 6m away from the wall and the ladder just reaches the top of the wall, how high is the wall?
View SolutionA ladder 5 m long leans against a vertical wall. The bottom of the ladder is 3m from the wall. If the bottom of the ladder is pulled 1 m farther from the wall, how much does the top of the ladder slide down the wall
View Solution(i) A uniform ladder of length L and weight w rests against a vertical wall and makes an angle θ with the horizontal ground. If the coefficient of friction at the point of contact of the ladder with the wall and ground is μ, show that the greatest height x, measured along the ladder from the foot to which a man of weight w may climb without the ladder slipping is given by
xL=μ(W+w)W(1+μ2)(μ+tanθ)−w2W
(ii) If the wall be smooth and coefficient of friction between ladder and ground be 0.25, show that
x=L4(1−wW)tanθ−wL2WView SolutionA ladder rests against a frictionless vertical wall, with its upper end 6m above the ground and the lower end 4m away from the wall. The weight of the ladder is 500N and its CG at 1/3rd distance from the lower end. Wall's reaction will be (in newton)
View Solution

