How are stationary waves formed in closed pipes ? Explain the various modes of vibrations and obtain relations for their frequencies.

Text Solution

Verified by Experts

A pipe, Which is closed at one end and the other is opened is called closed pipe. When a sound wave is sent through a closed pipe, which gets reflected at the closed end of the pipe.Then incident and reflected waves are in same frequency, travelling in the opposition direction are superimposed stationary waves are formed.
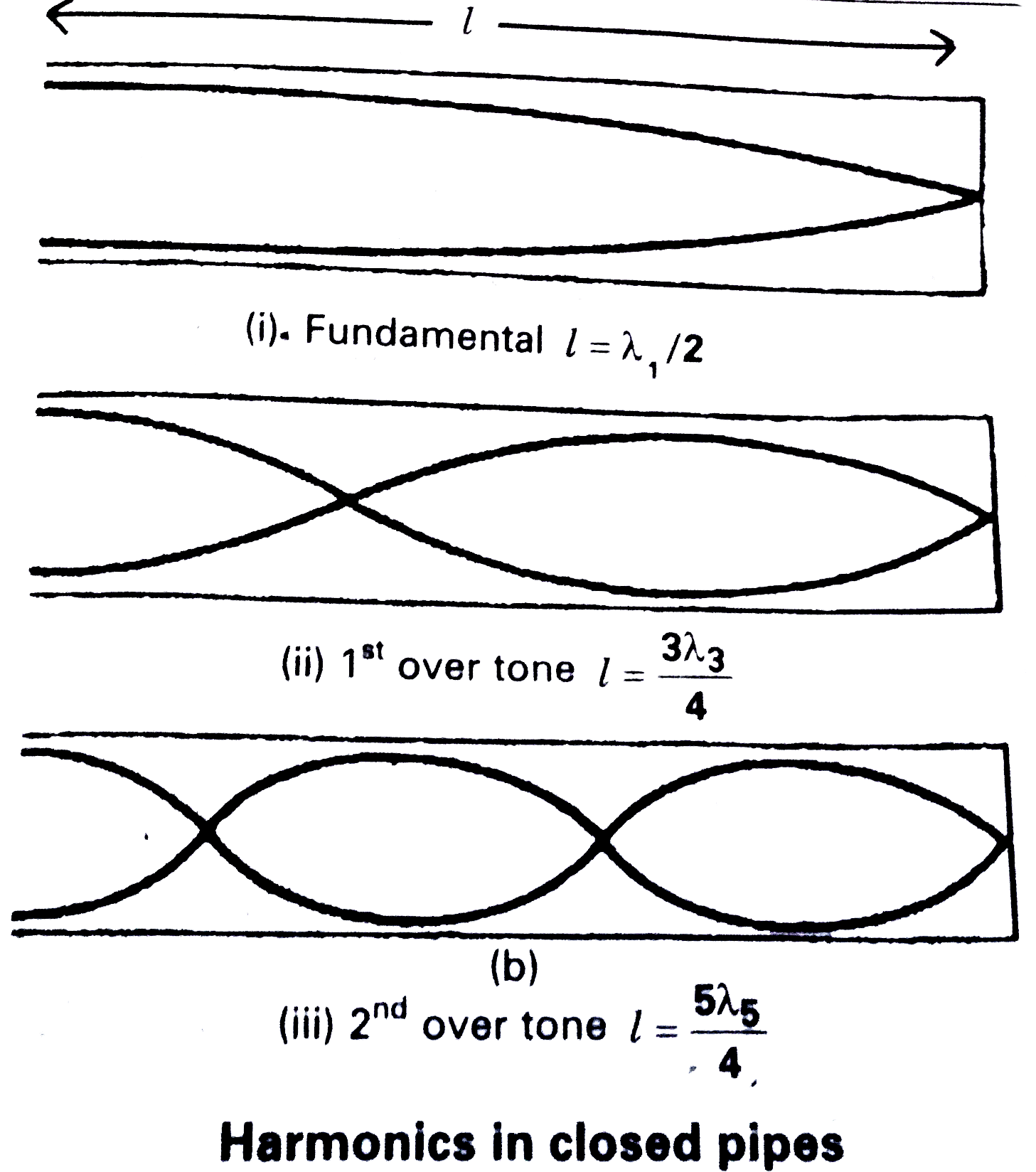
To form the stationary wave in closed pipe, which has atleast a node at closed end and antinode at open end of the pipe, it is known as first harmonic in closed pipe. Then length of the pipe ( l ) is equal to one fourth of the wave length.
$∴ l = \frac{λ_{1}}{4} \Rightarrow λ_{1} = 4 l$
If $' v_{1}'$ is fundamental frequency then
$v_{1} = \frac{υ}{λ_{1}}$ where $' υ'$ is velocity of sound in air
$v_{1} = \frac{υ}{4 l} = v$ ...(1)
To form the next harmonic in closed pipe, two nodes and two antinodes should be formed. So that there is possible to form third harmonic in closed pipe. Since one more node and antinode should be included.
Then length of the pipe is equal to $\frac{3}{4}$ of the wavelength
$∴ l = \frac{3 λ_{3}}{4}$ where $' λ_{3}'$ is wavelength of third harmonic
$λ_{3} = \frac{4 l}{3}$
If $' v_{3}'$ is third harmonic frequency ( first overtone )
$∴ v_{3} = \frac{υ}{λ_{3}} = \frac{3 υ}{4 l}$
$v_{3} = 3 v$ ...(2)
Similarly the next overtone in the close pipe is only fifth harmonic it will have three nodes and 3 antinodes between the closed end and open end.
Then length of the pipe is equal to $\frac{5}{4}$ of wave length $(λ_{5})$
$∴ l = \frac{5 λ_{5}}{4}$ where $' λ_{5}'$ is wave length of fifth harmonic .
$λ d_{5} = \frac{4 l}{5}$
If $' v_{5}'$ is frequency of fifth harmonic ( second overtone )
$v_{5} = \frac{υ}{λ_{5}} = \frac{5 υ}{4 l}$
$v_{5} = 5 v$ ...(3)
$∴$ The frequencies of higher harmonics can be determined by using the same procedure . Therefore from the Eq (1) , (2) and(3)only odd harmonics are formed.
Therefore the ratio of the frequencies of harmonics in closed pipe can be written as
$v_{1} : v_{3} : v_{5} = v : 3 v : 5 v$
$v_{1} : v_{3} : v_{5} = 1 : 3 : 5$

Updated on:21/07/2023

Stationary waves in closed pipe will produce
Aall the harmonics.
Ball the even harmonics.
Call the odd harmonics.
Dno harmonics.
when a stationary wave is formed then its frequency is
Asame as that of the individual waves
Btwice that of the individual waves
Chalf that of the individual waves
D $\sqrt{2}$ that of the individual waves
when a stationary wave is formed then its frequency is
Asame as that of the individual waves
Btwice that of the individual waves
Chalf that of the individual waves
D $\sqrt{2}$ that of the individual waves