Two parallel plate capacitors of capacitance C each are connected in series with a battery of emf ε. Then, one of the capacitors is filled with a dielectric of dielectric constane K.
i. Find the change in electric field in the two capacitors, if any
ii. What amount of charge flows through the battery?
iii Find the change in the energy stored in the circuit, if any.
i. Two capacitors A and B initially have same charge Q and potential V=Q/C. The electric field between the capacitor plates is given by E=V/d. Since the two capacitors are connected series with the battery, the sum of patentials across the capacitors must be equal to (ε),i.e.,
ε=2V=2QC (i)
ii. When one of the capacitors, say A, if filled will a dielectric, the capacity of A increases to C'=KC, that of B remains unchanged, i.,C. Suppose, charge on the capacitors becomes (A)' and potentials across A and B become VA and V'B, respectively, with V'A+V'B=ε. Hence, we have
V'A=Q'C'=Q'KC
and V'B=Q'C'
Hence,
ε=Q'C'(1+1K) (ii)
From Eqs. (i) and (ii) we get
Q'=2K1+KQ
Since K>1,so Q'>Q. Also
V'A=2K1+KV
and V'B=2K1+KV
Thus the electric field (or potential difference) in capacitor A increase by a factor of 2K/(1+K) while that in B increases by ka factor of 2K/(1+K). The amount of charge that flows into the circuit is given by
ΔQ=Q'−Q(2K1+K−1)Q=K−1k+1Q
12K−1K+1Cε
iii. Initially, the energy is given by
Ui=2×12CV2=CV2
Finally, the energy is
Uf=12C'V+(A)2+12CV'2B
=12KC(21+K)2V2+12C(2K1+K)2V2
=4K1+K(12CV2)=2K1+KUi
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Exercise 4.113videos
CAPACITOR AND CAPACITANCE
- BOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Exercise 4.232videos
CAPACITOR AND CAPACITANCE
- BOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer5videos
CAPACITOR AND CAPACITANCE
- BOOK - CENGAGE PHYSICSCHAPTER - ATOMSEXERCISE - QUESTION BANK40videos
ATOMS
- BOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type51videos
CENGAGE PHYSICS DPP
Similar Questions
Two parallel plate air capacitance C, were connected in series to a battery with emf ξ. Then one of the capacitors was filled up with uniform dielectric with permittivity ε. How many times did the electric field strength in that capacitor decrease ? What amount of charge flows throgh the battery ?
View SolutionTwo parallel plate capacitors, each of capacitance 40 muF, are connected is series. The space between the plates of one capacitor is filled with a dielectric material of dielectric constant K =4. Find the equivalent capacitacne of the system.
View Solution
Knowledge Check
- Question 1 - Select One
Two identical parallel plate capacitors of capacitance C each are connected in series with a battery of emf, E as shown. If one of the capacitors is now filled with a dielectric of dielectric constant k, the amount of charge which will flow through the battery is (neglect internal resistance of the battery)
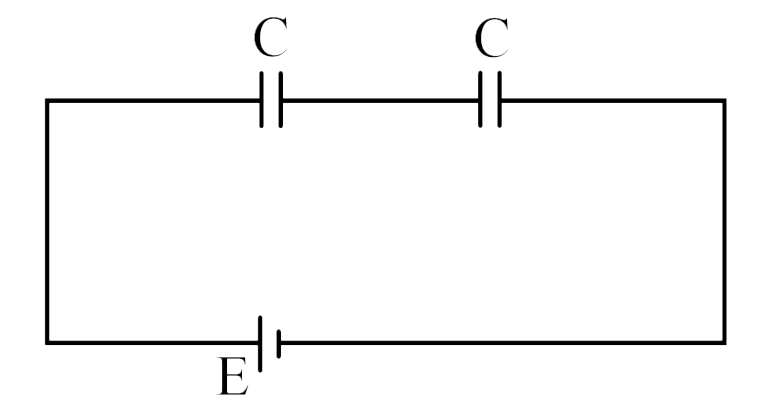 Ak+12(k−1)CEBk−12(k+1)CECk−2k+2CEDK+2k−2CE
Ak+12(k−1)CEBk−12(k+1)CECk−2k+2CEDK+2k−2CE - Question 2 - Select One
Two parallel plate air capacitance of same capacity C are connected in series to a battery of emf E. Then one of the capacitors is completely filled with dielectric material of constant K. The change in the effective capacity of the series combination is
AC2[K−1K+1]B2C[K−1K+1]CC2[K+1K−1]DC2[K−1K+1]2 - Question 3 - Select One
Two parallel plate air filled capacitors each of capacitance C, are joined in series to a battery of emf V. The space between the plates of one of the capacitors is then completely filled up with a uniform dielectric having dielectric constant K. The quantity of charge which flows through the battery is -
ACV2(K−1K+1)BCV2(K+1K−1)CCV(K−1K+1)DCV(K+1K−1)
Two parallel plate air capacitors, each of capacitance C=50 were connected is series ot emf ε=100V. Then one of the capacitors was filled with a uniforme dielectirc of relative permittivity ε=5 Compare the before and after the insertion of the dielectric? What amout of charge flows from the battery?
View SolutionA capacitor of some capacitance is charged by a 12V battery.Now the space between the plates of capacitors is filled with a dielectric of dielectric constant K=0.5 and again it is charged. The magnitude of the charge will
View SolutionTwo identical parallel plate air capacitors are connected in series to a battery of emf V. If one of the capacitor is completely filled with dielectric material of constant K, then potential difference of the other capacitor will become
View SolutionA parallel plate capacitor of capacitance 10μF is connected across a battery of emf 5 mV. Now, the space between the plates of the capacitors is filled with a deielectric material of dielectric constant K=5. Then, the charge that will flow through the battery till equilibrium is reached is
View SolutionTwo capacitors of capacitance C are connected in series. If one of them is filled with dielectric substance K, what is the effective capacitance?
View Solution

