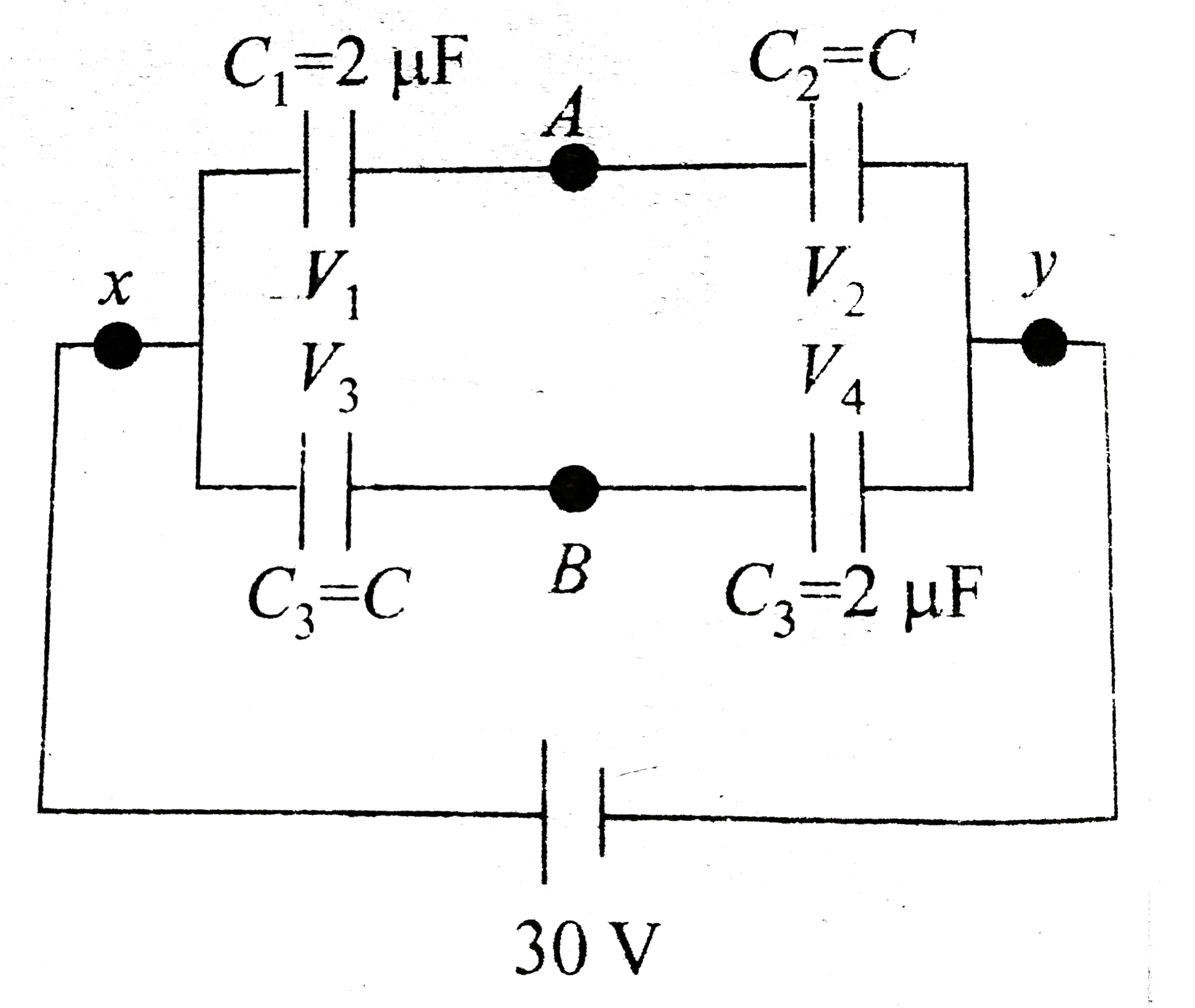
 .
.
Let us now connect two more capacitors in the circuit. One of them, C5, is connected in the part of the circuit between X and A. It connected between either in series or in parallel with C1. The other, C6, is connected between AandB. It is observed XandY has the the same value C5 is.
Capacitance C5 will be.
32μC
28μC
24μC
16μC
The correct Answer is:C
Potential differnce across C4 is V4. As discussed above
V4=V1
V4=17.5V
Consider any branch say, XAY
Since C1 and C2 are in series, charge on C2 is also 35μC.
Using Q=CV for C2
C2=C=35V2=3512.5=2.8μF
Equivalent capacitance of branch XAY is the series equivalent of C3=C=2.8μF and C4=2μF i.e., 1.17μF. There branches are in parallel between X and Y, Hence equivalent capacitance between X and Y is 1.17+1.17=2.34μF.
Capacitor C5 is connected in the part of circuit between X and A either in series or parallel with C1=2μF. Let the equivalent capacitance of C1 and C5, i.e., the equivalent capacitance between X and A be C'1.
Capacitor C6 is connected between A and B Obviously, the circuit then becomes a Wheatstone bridge. Further, since equivalent capacitance between X and Y is independent of the value of C6, it implies that bridge is in the balanced condition and potentials at A and B are now equal, so that
C'1C3=C2C4 or C'12.8=2.82 (C2=2.8μF, as or determined earlier)
or C1=3.92μF
C'1 is the equivalent capacitance of C1=2μF and C5, Since C'1>C1, we can conclude that C1 and C5 are in parallel, So each individual capacitance .Hence, C1 and C5 are in parallel, so
C'1=C1+C5 or C5=C'1−C1=3.92−2=1.92μf
V'1+V2=30
 .
.
 .
.
Since the bridge is in a balanced condition, A and B at same potential and C6 has zero charge. C'1 and C2 are in series. Therfore, charge on C'1 and C2 has the same value, so that potential differnce will be in the inverse ratio of capacitance
[Q=CV or V=QChence for sameQ,V∝1C']
V'1V'2=2.83.92≈0.71 or V'1=0.71V2
From Eq. (iv), 0.71V2+V2=30 or V2=17.5V
Hence V'1=30−V2=12.5V
Since C'1, is parallel equivalet of C1 and C5, so potential differnce across each is V'1=12.5V.
Hence, charge on C5 is
C5V'1=1.92×12.5=24μC.
Topper's Solved these Questions
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Multile Correct
11 Videos
- ATOMSBOOK - CENGAGE PHYSICSCHAPTER - ATOMSEXERCISE - QUESTION BANK
40 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
Similar Questions
Let us now connect two more capacitors in the circuit. One of them, C5, is connected in the part of the circuit between X and A. It connected between either in series or in parallel with C1. The other, C6, is connected between AandB. It is observed XandY hass the the same malue C5 is.
Capacitance C5 is.
View SolutionTwo capacitors with capacitances C1andC2 are connected in series. Find the resultant capacitance.
View Solution
Knowledge Check
 .
.
Let us now connect two more capacitors in the circuit. One of them, C5, is connected in the part of the circuit between x and A. It connected between either in series or in parallel with C1. The other, C6, is connected between AandB. It is observed xandy has the the same value C5 is.
Capacitance C5 is.A1.24μF in series with C1B1.92μF ih parallel with C1C2.28μF in series with C1D2.56μF in parallel with C1
 .
.
Let us now connect two more capacitors in the circuit. One of them, C5, is connected in the part of the circuit between X and A. It connected between either in series or in parallel with C1. The other, C6, is connected between AandB. It is observed XandY has the the same value C5 is.
Capacitance C5 will be.A32μCB28μCC24μCD16μCIf By mistake Battey is connected between B and C Galvanometer is connected across A and C then .
AWe cannot get balanced pointBExperiment will be less accurateCExperiment can e done in similar mannerDExperiment can be done in similar manner but now K2 should be pressed first then K1 .
Two capacitors with capacitances C1andC2 are connected in parallel. Find the resultant capacitance.
View SolutionTwo capacitors with capacitances C1andC2 are connected in parallel. Find the resultant capacitance.
View SolutionFour identical capacitors are connected as shown in diagram. When a battery of 6V is connected between A and B, the charges stored is found to be 1.5μC. The value of C1 is
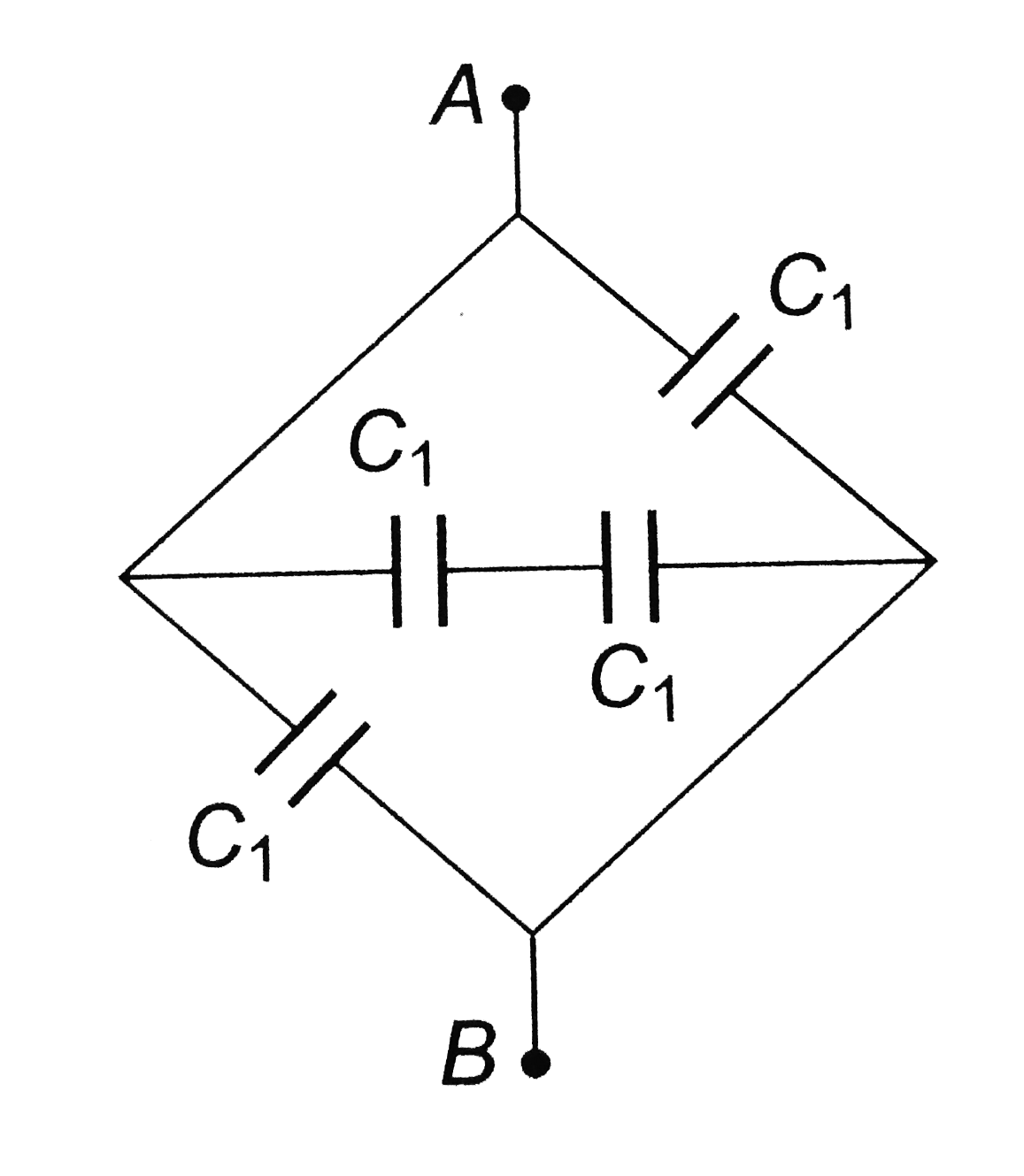
View SolutionFour identical capacitors are connected as shown in diagram. When a battery of 6V is connected between A and B, the charges stored is found to be 1.5μC. The value of C1 is

View SolutionThree capacitors Catcb<Cc are connected in series. Their resultant capacitance will be
View Solution
