The temperature coefficient of resistance of conductor varies as If is resistance at T = 0and R is resistance at T, then
The correct Answer is:C
In the steady state no current flow through C.
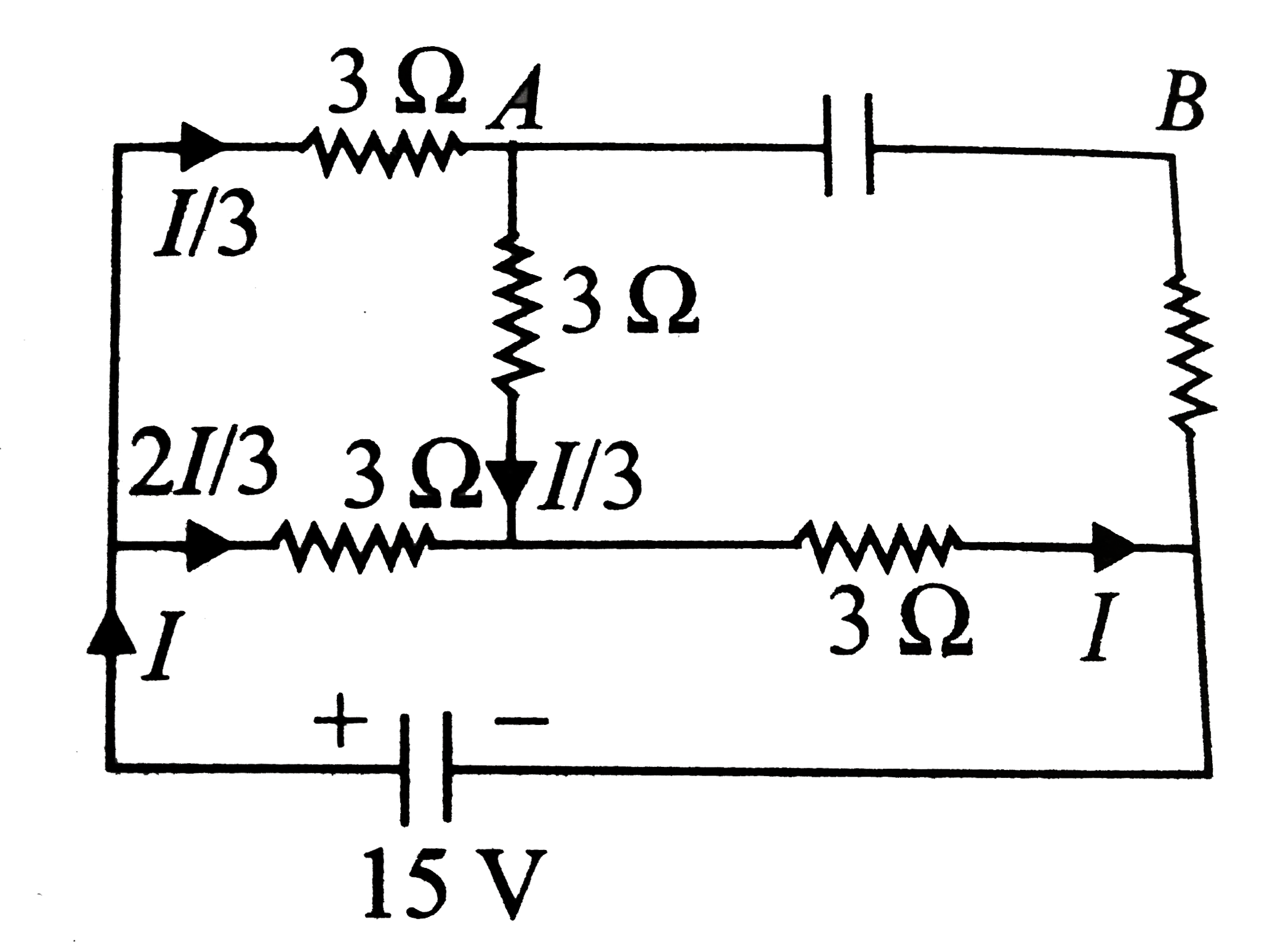
or
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Multiple Correct16videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Comprehension35videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Subjective29videos
ELECTRIC CURRENT AND CIRCUIT
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits15videos
ELECTRIC CURRENT & CIRCUITS
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s38videos
ELECTRIC FLUX AND GAUSS LAW
Similar Questions
Obtain a general relationship between temperature coefficient of resistance α1 and α2 at temperature T1.∘C and T2.∘C for a given conductor.
View SolutionA conductor has a temperature independent resistance R and a total heat capacity C. At the moment t=0 it is connected to a DC voltage V. Find the times dependence of the conductors temperature t assuming the thermal power dissipated into surrounding space to vary as q=k(T−T0) where k is a constant T0 is the surrounding temperature (equal to conductor's temperature at the initial moment).
View Solution
Knowledge Check
- Question 1 - Select One
At 0∘C the electron of conductor of a conductor B is n times that of conductor A temperature coefficient of resistance for A and B are α1andα2 respectively the temperature coefficient of resistance of a circuit segment constant A and B in series is
Anα1+nα21+nBnα1−nα21+nCα1+nα21+nDα2+nα11+n - Question 2 - Select One
The temperature (T) dependence of resistivity (rho) of a semiconductor is represented by :
A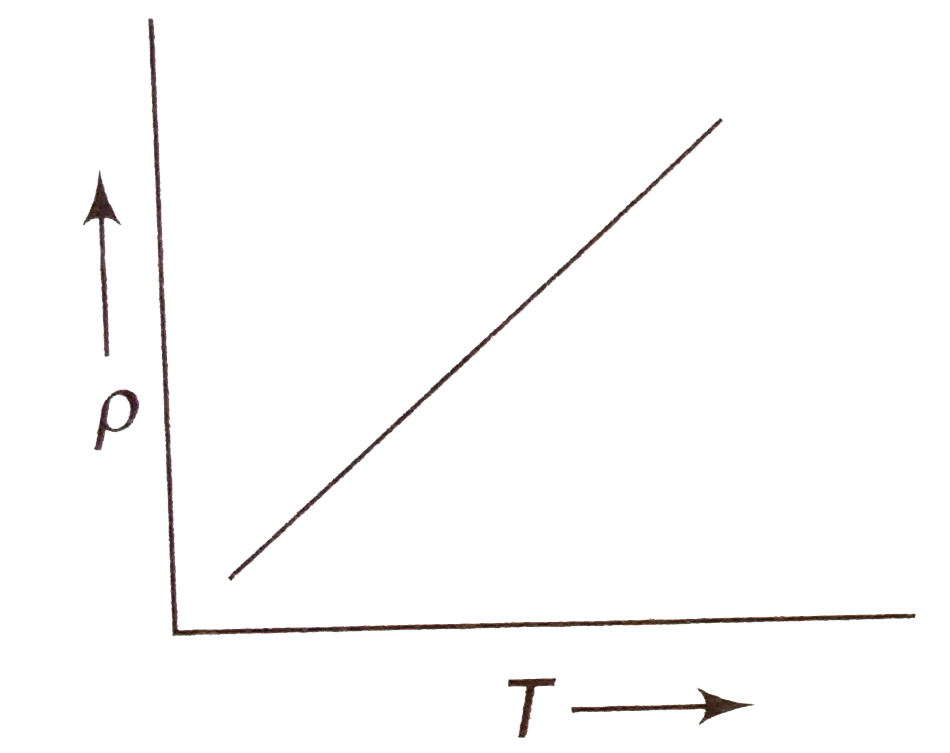 B
B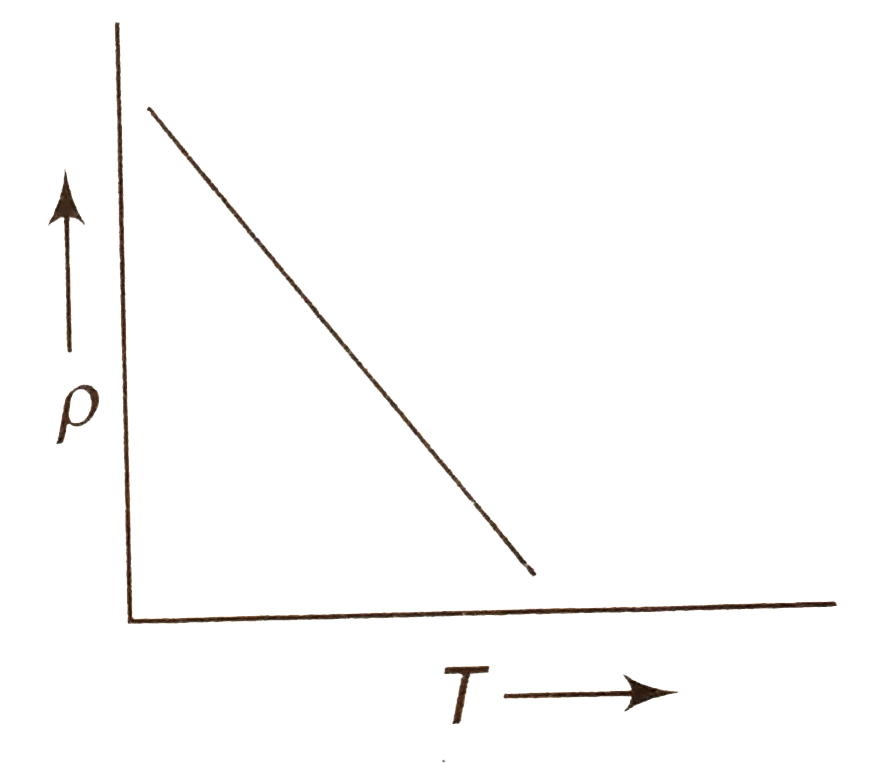 C
C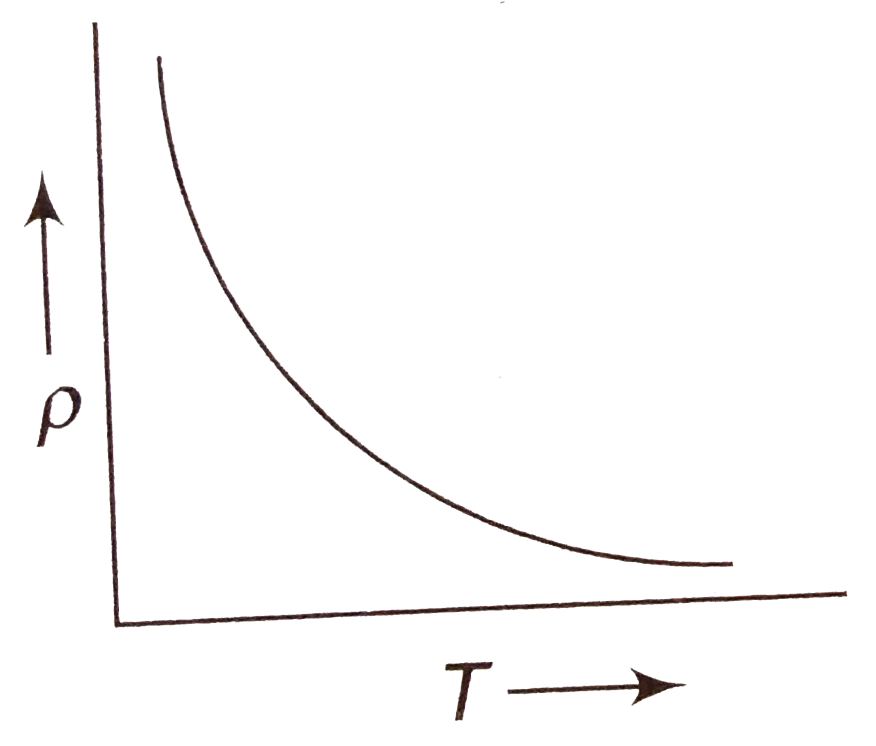 D
D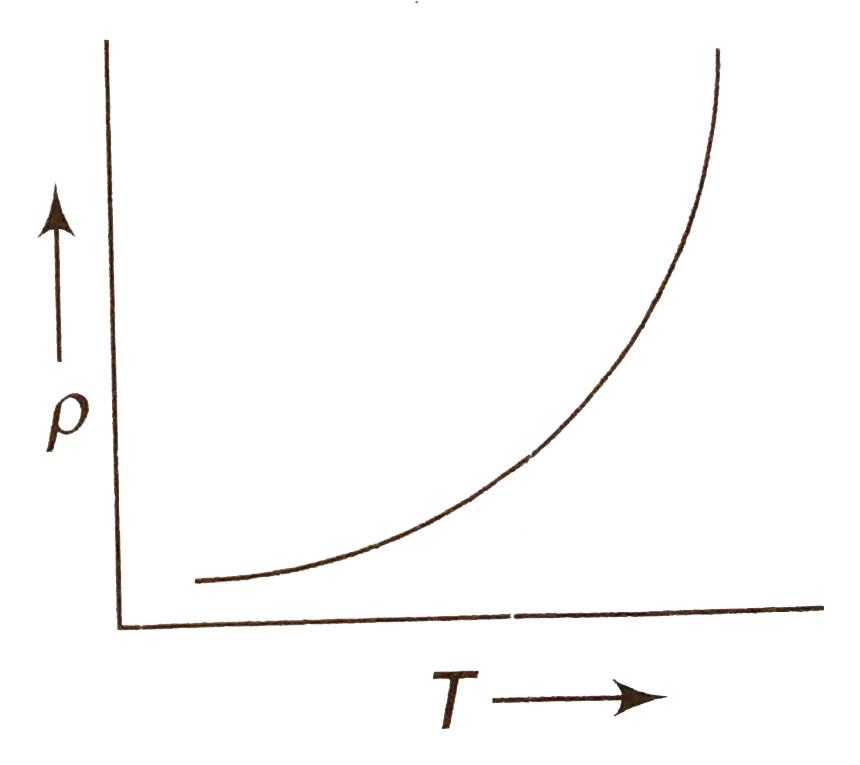
- Question 3 - Select One
Choose the most appropriate option.
The resistance R of a conductor varies with temperature t as shown in figure. If the variation is represented by Rt=R0(1+αt+βt2).
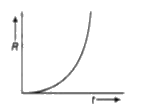
ThenAαandβ bothe negativeBα is positive and β is negativeCαandβ both are positiveDα is negative and β is negative
A plarinum resistance thermometer is condtructed which resds ,00 at ice point and 1000 at steam point. Let tpde¬etemoeratureonthissca≤and≤ttde¬ethetemperatureonametcuryonamercurythemomentersca≤.Theresistanceoftheplat∈umcoilvareswithtasR_1 = R_0 (1+alphat + betat^2).DeriveanexpressionfortheresistanceasafunctionofT_p.
View SolutionA conductor in the shape of a cylinder of length l and cross sectional radius r is connected to a cell of emf V. The resistivity of the material of the conductor is ρ and does not change much with temperature. The emissivity of the curved surface of the conductor is e. [Take emissivity of the flat circular surfaces to be zero]. In steady state the temperature of the conductor is T when the environmental temperature is T0. The difference between TandT0 is much smaller than the environmental temperature. Stefan’s Constant is σ.
Find the steady state temperature T for the conductor.
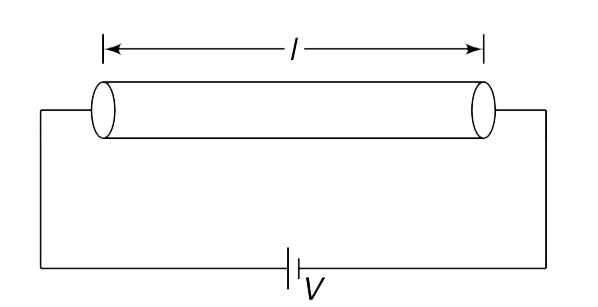 View Solution
View SolutionResistance of a resistor at temperature t∘C is Rf=R0(1+αt+βt2) . Here R0 is the resistance at 0∘C .The temperature coefficient of resistance at temperature t∘C is
View SolutionThe corfficient of linear expansion for a certain metal varies with temperature as α(T). If L0 is the initial elgnth of the metal and the temperature of metal is changed from T0 to T(T0>T), then
View SolutionResistance of a resistor at temperature t∘CisRt=R0(1+αt+βt2), where R0 is the resistance at 0∘C. The temperature coefficient of resistance at temperature t∘C is
View Solution

