A loop of flexible conducting wire of length 0.5 m lies in a magnetic field of 1.0 T perpendicular to the plane of the loop. Show that when a current as shown in Fig. 1.112 is passed through the loop, it opens into a circle. Also calculate the tension developed in the wire if the current is 1.57 A.

Video Solution

Text Solution

Verified by Experts

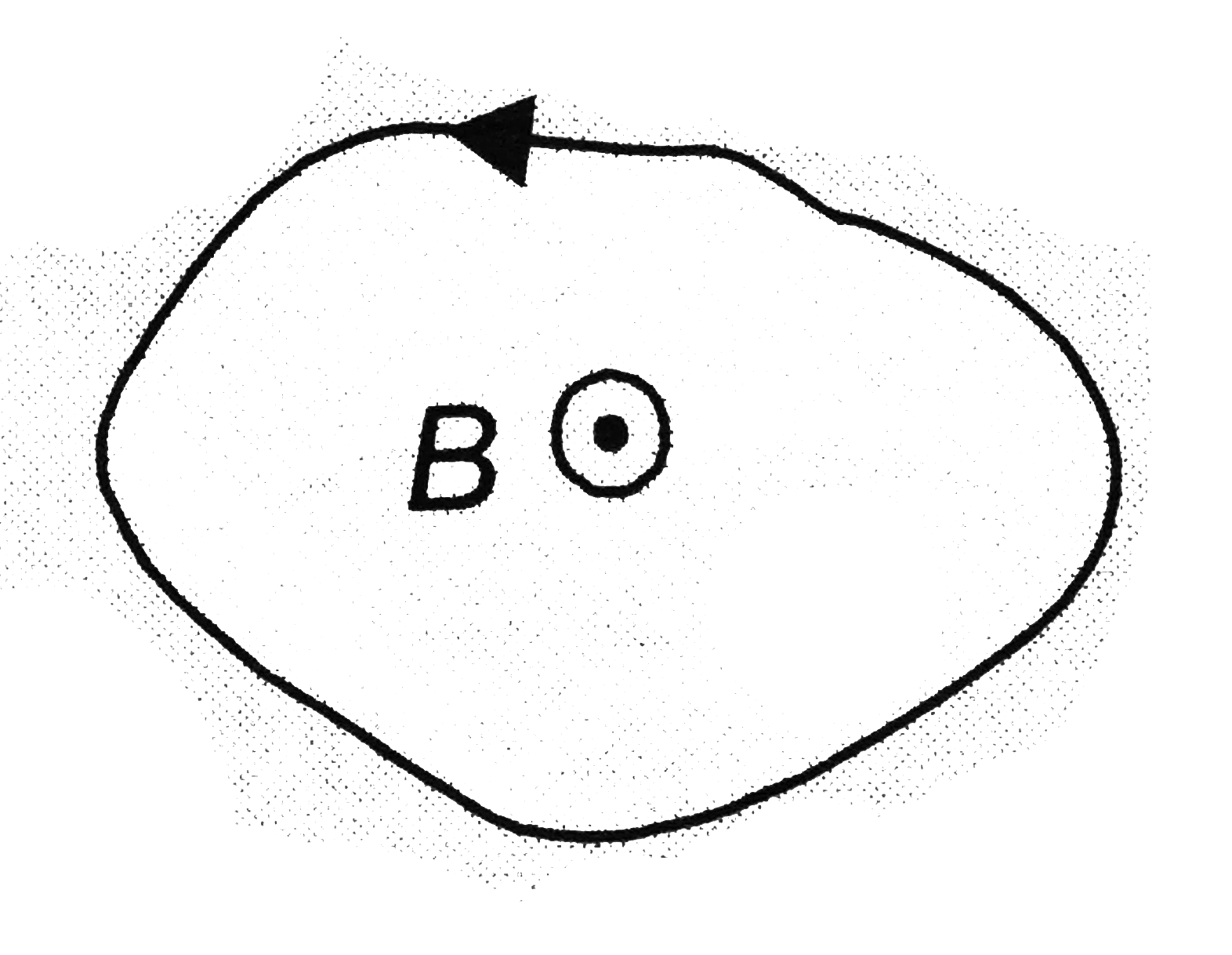
The loop may be divided into a large number of small length elements. When a current I is passed through the loop placed in the magnetic field such that the plane of the loop is perpendicular to field, then force on each element is
$d \to F = I d \to l \times \to B = I d l B {sin 90}^{\circ}$
$= I d l b$

Perpendicular to current element $I \to d l$ as well as magnetic field $\to B$ . Hence, the loop opens into a circle.
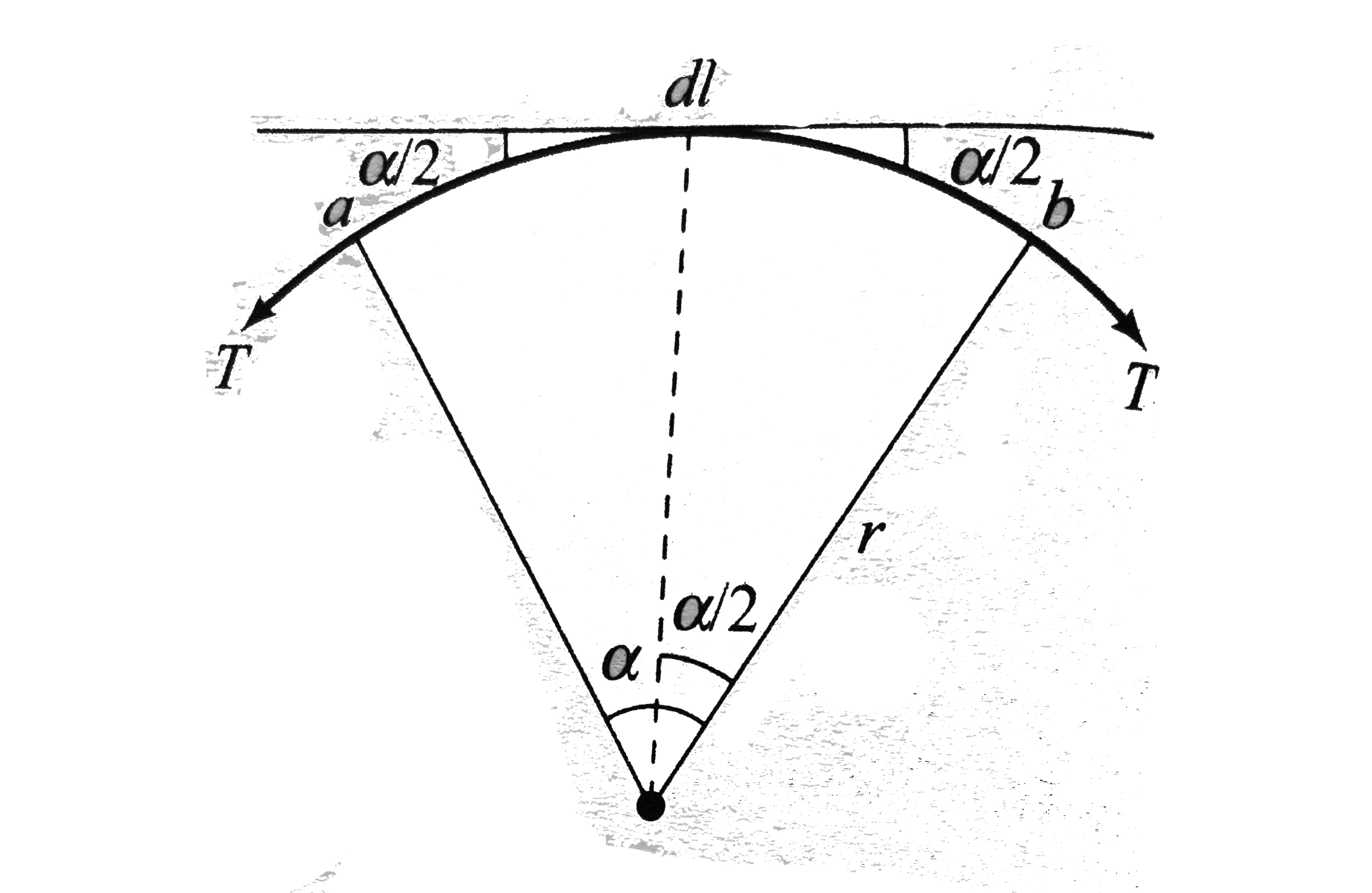
Consider an element of length dl of circle of radius r, making an angle $α$ at centre. If T is the tension in the wire, then force toward centre
$2 T sin α .2 = I B d l$
$T = \frac{I B d l}{α} = I B r ⟨ . α = d \frac{l}{r})$
$= I B (\frac{l}{2 π}) (∴ l = 2 π r)$
$= 1.57 \times 1 \times (\frac{0.5}{2 \times 3.14}) = 0.125 N$
.

Updated on:21/07/2023

A loop of flexible conducting wire lies in a magnetic field of 2.0 T with its plane perpendicular to the field. The length of the wire is 1 m. When a current of 1.1A is passed through the loop, it opens into a circle, then the tension developed in the wire is
A0.15 N
B0.25 N
C0.35 N
D0.45 N
A loop of flexible conducting wire of length l lies in magnetic field B which is normal to the plane of loop. A current l is passed through the loop. The tension developed in the wire to open up is
A $\frac{π}{2} B i l$
B $\frac{B i l}{2}$
C $\frac{B i l}{2 π}$
D $B i l$
The magnetic field perpendicular to the plane of a loop of area $0.1 m^{2}$ is 0.2 T . Calculate the magnetic flux through the loop (in weber)
A0.01
B0.02
C0.03
D0.04