Calculating the magnetic moment ( in Am2) of a thin wire with a current I=8A, wound tightly on a half a tore (see figure). The diameter of the cross section of the tore is equal to d=5cm, and the number of turns is N=500.
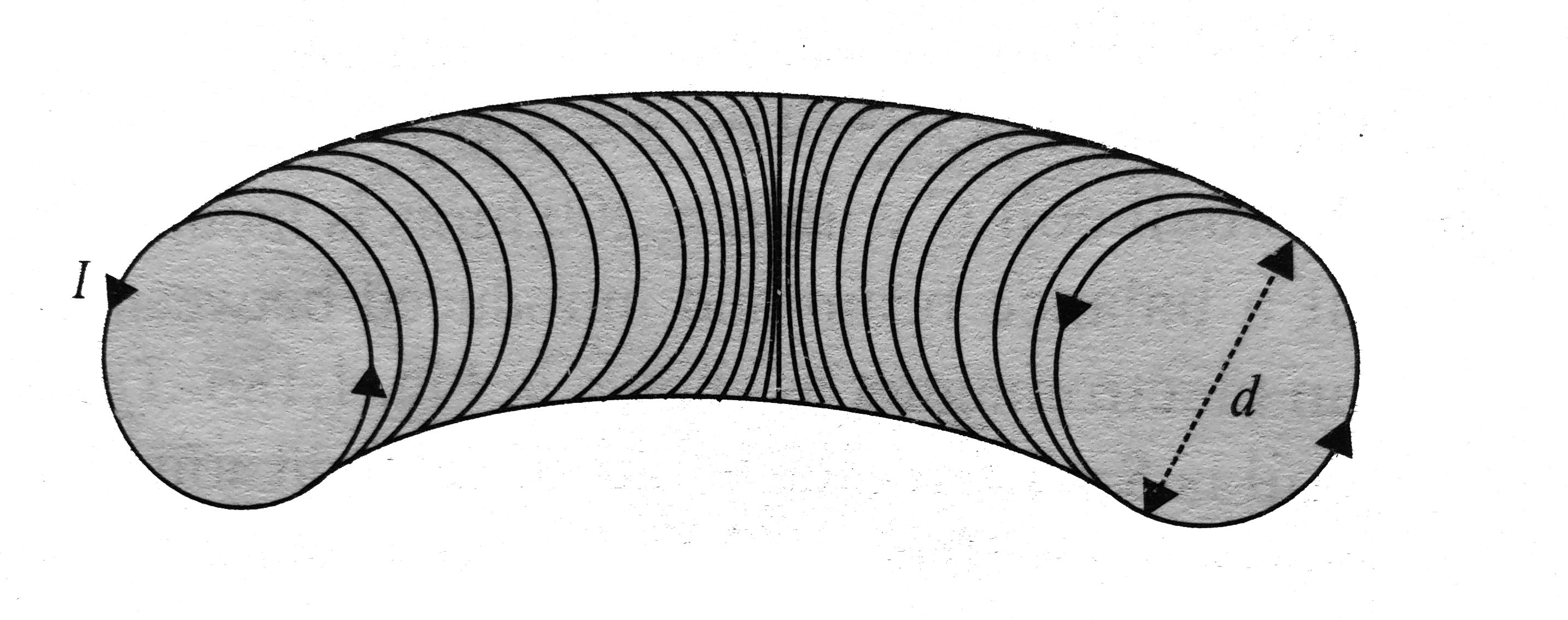
The correct Answer is:5
The magnetic moment of circular current is given by AI, I being
the curculating current and A is the area of cross-section, the
direction is perpendicular to the plane of current. Now, for an
element of toroid of length rdq, its magnetic moment is along the
direction of arrow as shown, of magnitude (perpendicular to its
cross-section)=currentxareaxnumber of turns in the length rdq.
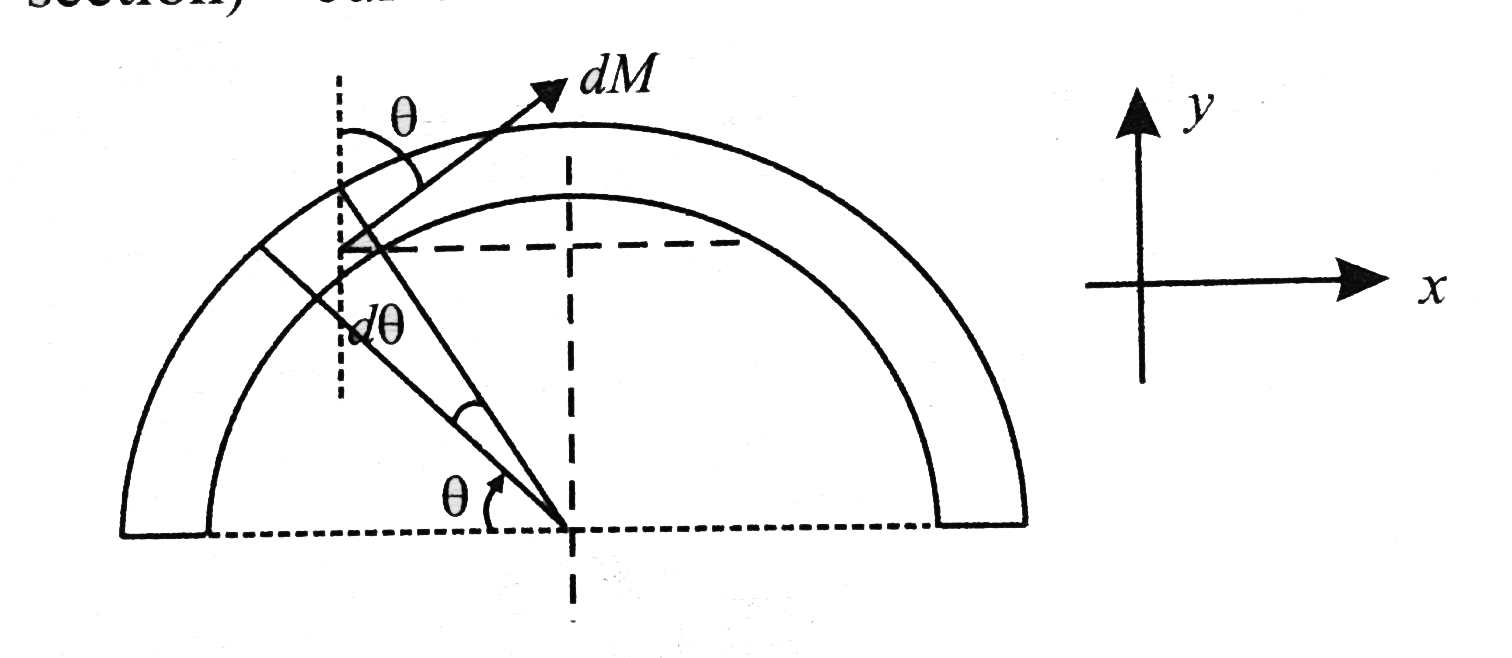
dM=Iπd24(Nπrrdθ)=N4d2Idθ
Resolving dM into components along x and y, we get dMsinθ
and dMcosθ, components along y form neightbouring elements
cancel out to zero, and components along x are added. so
M=∫dMsinθ=∫π0N4,d2Isinθdθ=Nd2I2
putting the given values we get M=5Am2.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Archives Fill In The Blank6videos
MAGNETIC FIELD AND MAGNETIC FORCES
- BOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Archives True /false4videos
MAGNETIC FIELD AND MAGNETIC FORCES
- BOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Linked Comprehension28videos
MAGNETIC FIELD AND MAGNETIC FORCES
- BOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank31videos
KINETIC THEORY
- BOOK - CENGAGE PHYSICSCHAPTER - Magnetism and MatterEXERCISE - Question Bank50videos
Magnetism and Matter
Similar Questions
Calculate the magnetic moment of a thin wire with a current I=0.8A, wound tightly on half a toroid (figure).the diameter of the cross-section of the tore is equal to d=5.0cm,the number of turns is N=500.
 View Solution
View SolutionA thin solenoid is made of a large number of turns of very thin wire tightly wound in several layers. The radii of innermost and outermost layers are a and b respectively and the length of the solenoid is L(L>>a,). The total number of turns is N. Calculate the self inductance of the solenoid. Neglect edge effects.
View Solution
Knowledge Check
- Question 1 - Select One
An electric motor winds 200 turns of a thin coper wire on a unifrom of diameter 10 cm, in 2 seconds.then the length of the wire that is wound over the rod in one second is _______ m.
A30B10πC20πDNone of these - Question 2 - Select One
Four wires of equal length 2 m are arranged as shown in the figure. Wires , 2, 3 and 4 are of equal cross-sectional area and wire 1 is of half the cross section of these wires. By how much distance pointer at point 5 must be moved to get null point ?
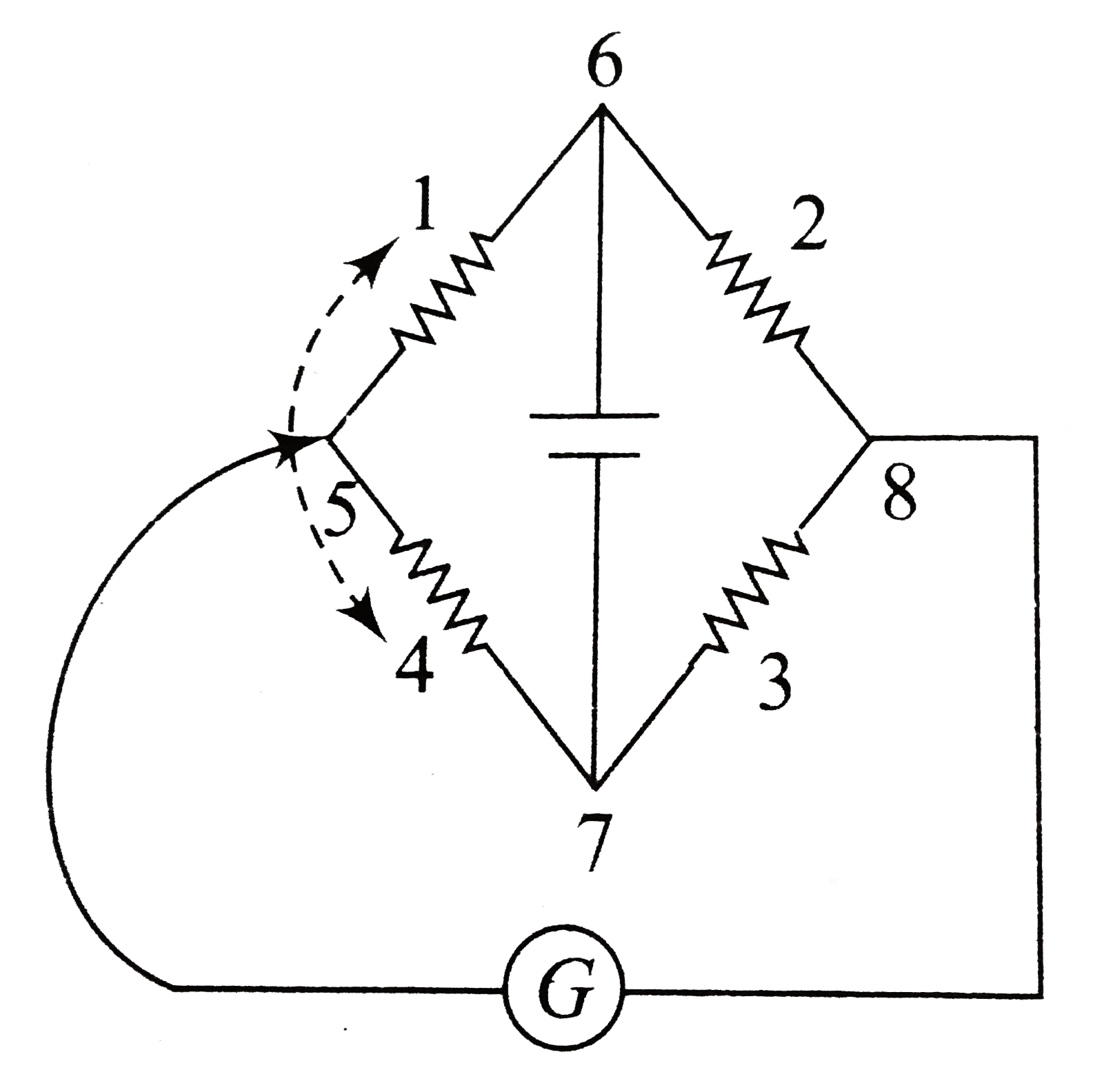 A0.5m toward point 6B0.5 m toward point 7C1 m toward point 6D1 m toward point 7
A0.5m toward point 6B0.5 m toward point 7C1 m toward point 6D1 m toward point 7
A thin wire is wound very tightly in one layer on the surface of a sphere of paramagnetic material (μr≈1). The planes of all the turns can be assumed to be perpendicular to the same diameter of the sphere. The turns over the entire surface of the sphere. The radius of the sphere is R, the total number of turns is N, and the current in the winding is I. Find the magnetic induction at the centre of the sphere. (Given μ0NI=20R)
View SolutionA long one− layer solenoid tightly would of wire with resistivity ρ has n turns per unit length. The thickness of the wire insulation is negligible. The cross− sectional radius of the solenoid is equal to a. Find the phase difference between current and alternating voltage fed to the solenoid with frequency v.
View SolutionA wire carrying current of 5 A is wound on a toroid in 4,000 turns, Calculate the magnetic field inside the core of toroid and outside the toroid if its inner radius is 15 cm and the outer radius is 18 cm.
View Solution(a) Find magnetic field inside a long solenoid, carrying current (1/π) A and having number of turns per unit length 10. Also calculate magnetic field at ends.
(b) A long solenoid is fabricated by closely winding a wire of diameter 10mm over a cylinderical non-magnetic frame so that the successive turns nearly touch each other. Find magnetic field at the centre and ends of solenoid if it carriers a current (4/π) A?
(c) A single-layer coil (solenoid) has length l and cross-section radius R. The number of turns per unit length is equal to n. Find the magnetic induction at the centre of the coil when a current i flows through it.
(d) A solenoid of length 0.4m and diameter 0.6m consists of a single layer of 1000 turns of the fine wire carrying a current of 5mA. Calculate the magnetic field on the axis at the middle and at the ends of the solenoid.View SolutionA thin ring made of magnetic has a mean diameter d=30cm and supports a winding of N=800 turns. The cross-sectional area of the ring is equal to S=5.0cm2. The ring has a cross-cut of width b=2.0mm. When the winding carries a certain current, the permeability of the magnectic equals μ=1400. Neglectign the disipation of magnetic flux at the gap edges, find:
(a) the ratio of magnetic energies in the gap and in the magnetic,
the inductance of the system, do it two ways: using the flux and using the energy of the field.View SolutionA closely wound solenoid 80cm long has 5 layers of windings of 400 turns each .The diameter of the solenoid is 1.8cm .If the current carried is 8A ,the magnetic field near the centre of the solenoid is ...
View Solution

