A conducting loop rotates with constant angular velocity about its fixed diameter in a uniform magnetic field, whose direction is perpendicular to that fixed diameter.

A

(a) The emf will be maximum at the moment when flux is zero

B

(b) The emf will be $0$ at the moment when flux ismaximum

C

( c) The emf will be maximum at the moment when plane of the loop is parallel to the magnetic field

D

(d) The phase difference between the flux and the emf $π / 2$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C, D

(a,b,c,d): $ϕ = B a cos θ, θ = ω t$
$e = \frac{- d ϕ}{d t} = B a ω sin θ$
at $θ = \frac{π}{2}, ϕ = 0$ and $e$ is maximum. At $θ = 0, ϕ$ is maximum and $e$ is zero. Emf is maximum where $θ = \frac{π}{2}$ and for this plane of loop is parallel to magnetic field. Clearly phase difference between flux and emf is $π / 2$ .

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROMAGNETIC INDUCTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
When a 'J' shaped conducting rod rotating in its own plane with constant angular velocity omega, avout one of its end P, in a uniform magnetic field $\to B$ directed normally into the plane of paper) then magnetic of emf induced across it will be

A $B ω \sqrt{L^{2} + l^{2}}$
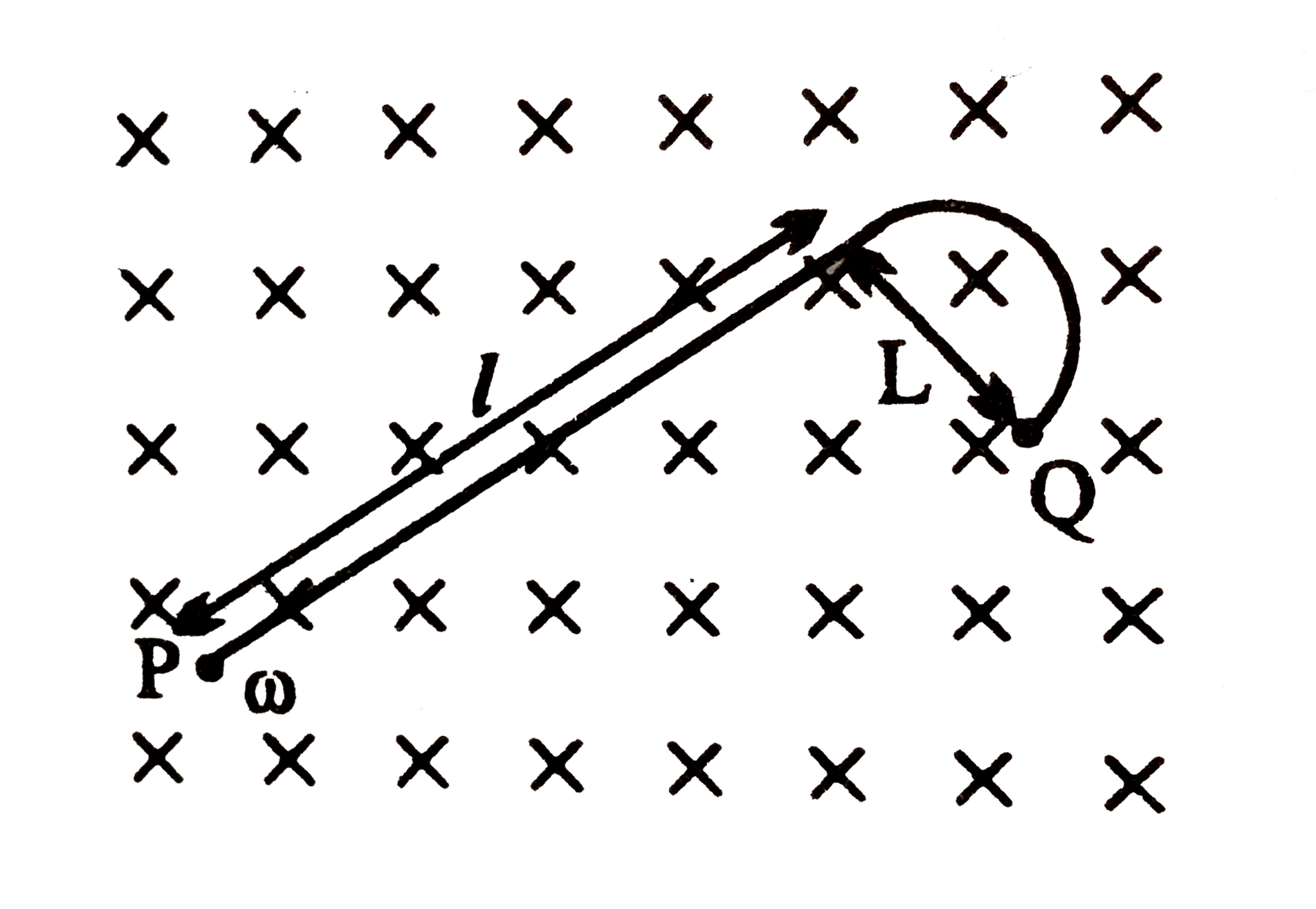B $\frac{1}{2} B ω L^{2}$
C $\frac{1}{B} ω (L^{2} + l^{2})$
D $\frac{1}{2} B ω l^{2}$
Question 2 - Select One
When a 'j' shaped conducting rod is rotating in its own plane with constant angular velocity $ω$ about one of its ends P in a uniform magnetic field $\to B$ ( directed normally into the plane of paper ) the magnitude of emf induced across it will be

A $B ω \sqrt{L^{2} + l^{2}}$
B $\frac{1}{2} B ω l L^{2}$
C $\frac{1}{2} B ω (L^{2} + l^{2})$
D $\frac{1}{2} B ω l^{2}$
Question 3 - Select One
A conducting rod of length $2 l$ is rotating with constant angular speed $w$ about its perpendicular bisector. A uniform magnetic field $B$ exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is

A $B ω l^{2}$
B $\frac{1}{2} B ω l^{2}$
C $\frac{1}{8} B ω l^{2}$
Dzero

CENGAGE PHYSICS-ELECTROMAGNETIC INDUCTION-Exercises Multiple Correct

Home
Ask Doubt
Profile