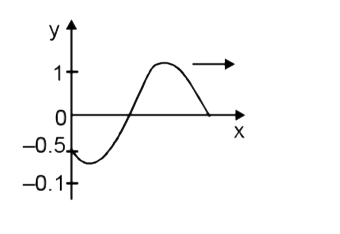
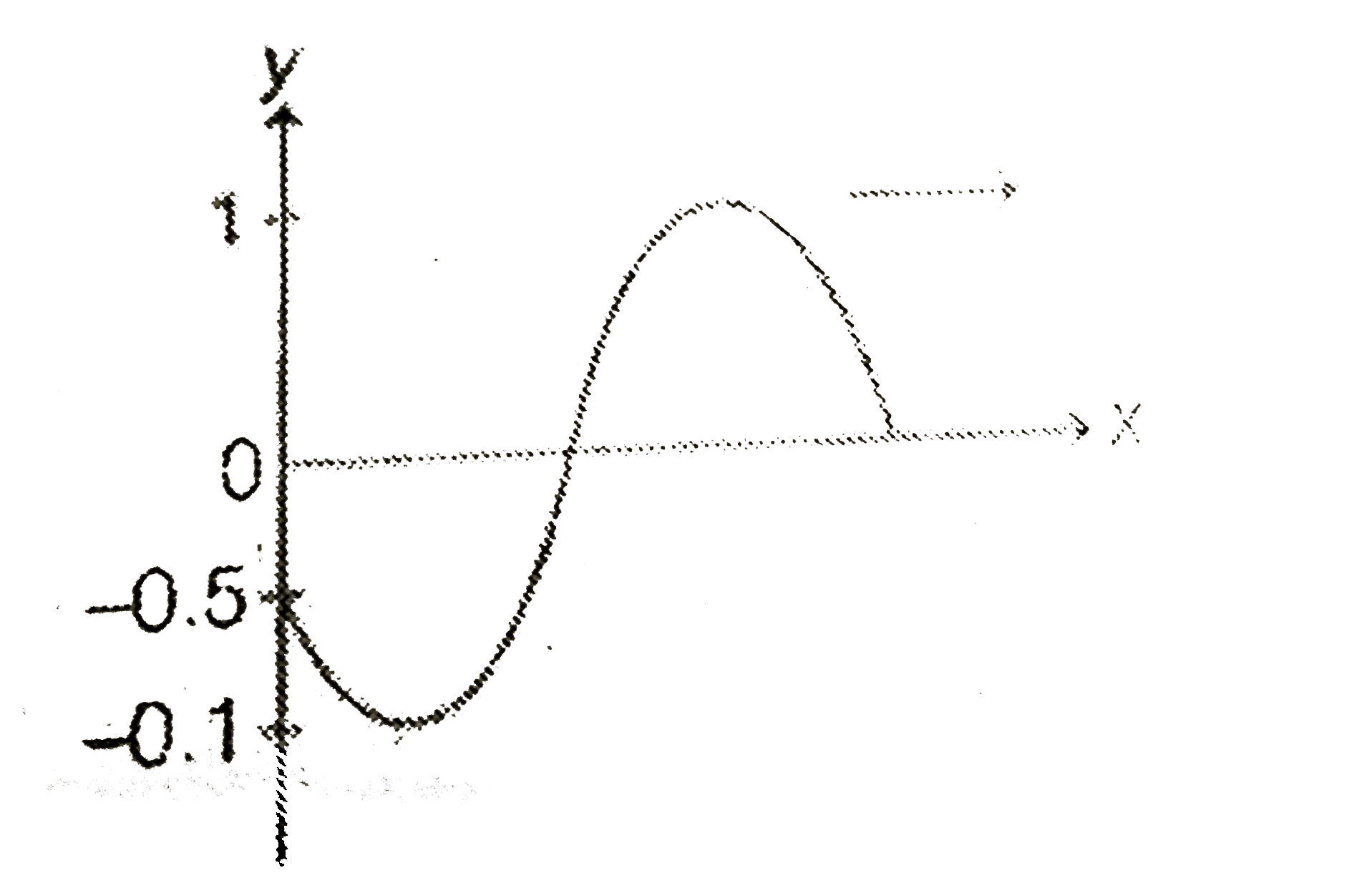
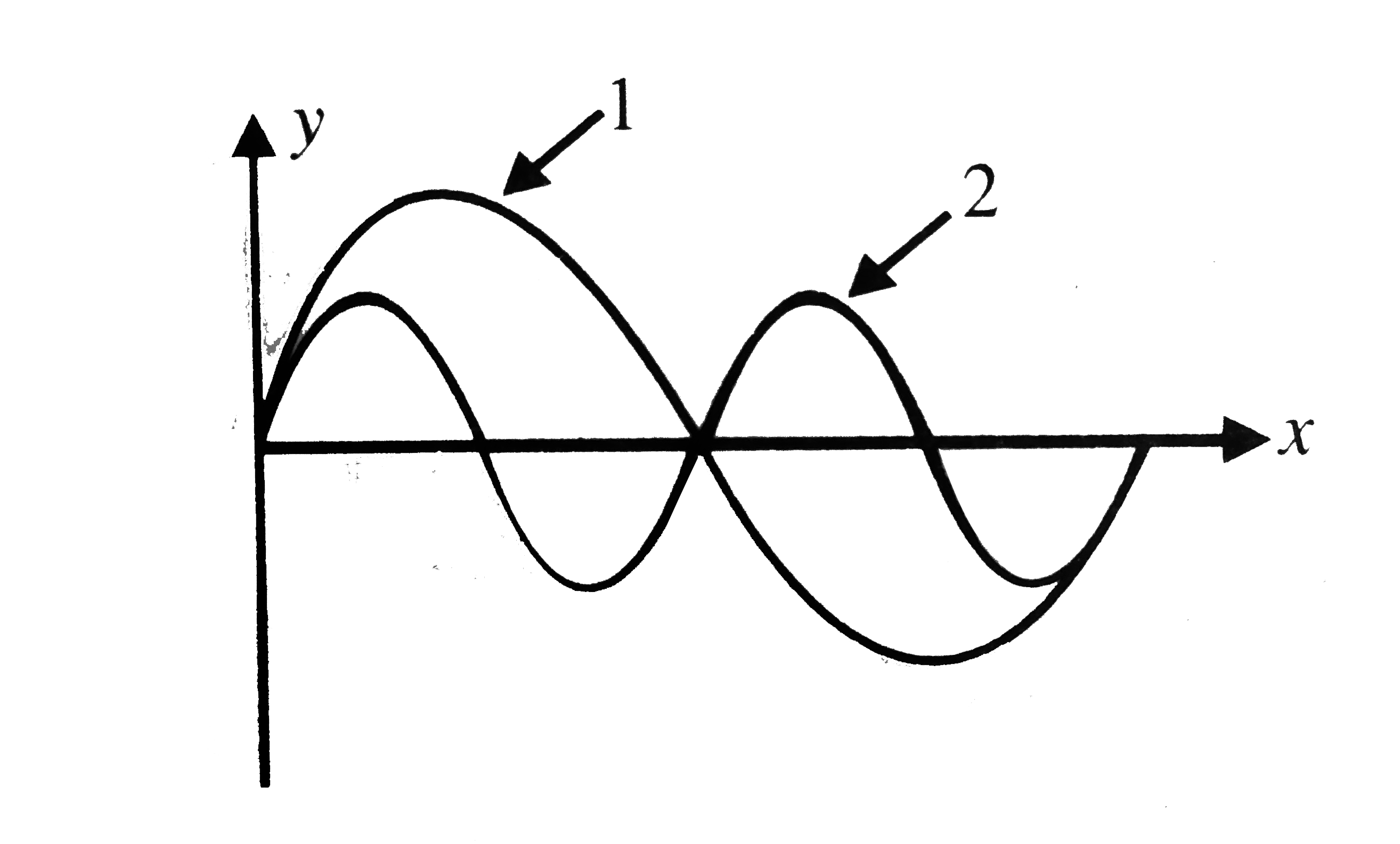
Figure .shows two snapshots of medium particle supporting a plane progressive wave travelling along positive x-axos, corresponding to instants $t = 0.002 s$ and $t = 0.008 s$ , respectively shown by curves numbered $1$ and $2$ .
Assume that the interval between the two snapshots is less than the time period.

The frequency of the wave

A

$52 s^{- 1}$

B

$205 s^{- 1}$

C

$104 s^{- 1}$

D

$54 s^{- 1}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:c

From the graph it is clear
wave velocity $v = \frac{(8 - 3)}{0.006}$
$v = \frac{5 \times 10^{3}}{6} m / s$
And wave velocity $v = f λ$
$\frac{5 \times 10^{3}}{6} = f (9 - 1) \Rightarrow f = \frac{5 \times 10^{3}}{6 \times 8} = 104 s^{- 1}$ Let $y = A sin (ω t - k x + ϕ)$
for curve (1):
$- \frac{1}{\sqrt{2}} = 1 sin (ω + ϕ) = - \frac{1}{\sqrt{2}}$
$\Rightarrow sin (0.002 ω + ϕ) = \frac{5 π}{4}$ or (7pi)/(4) $< b r > b u t a s t h e v e l o c i t y i s d o w n w a r d < b r > s o$ 0.002omega+phi=(5pi)/(4) $< b r > F or c u r v e (2) : < b r >$ 1=1 sin (omegaxx0.008+phi) $< b r >$ implies0.008omega+phi=2pi+(pi)/(2) $F r o m E q s . (i) and (i i), < b r >$ 6omega=1250pi $< b r >$ impliesf=(1250pi)/(12pi)=104 Hz $< b r >$ v=f lambda=(1250)/(12)xx8=(2500)/(3)m//s

|

Updated on:21/07/2023

Class 11 PHYSICS TRAVELLING WAVES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
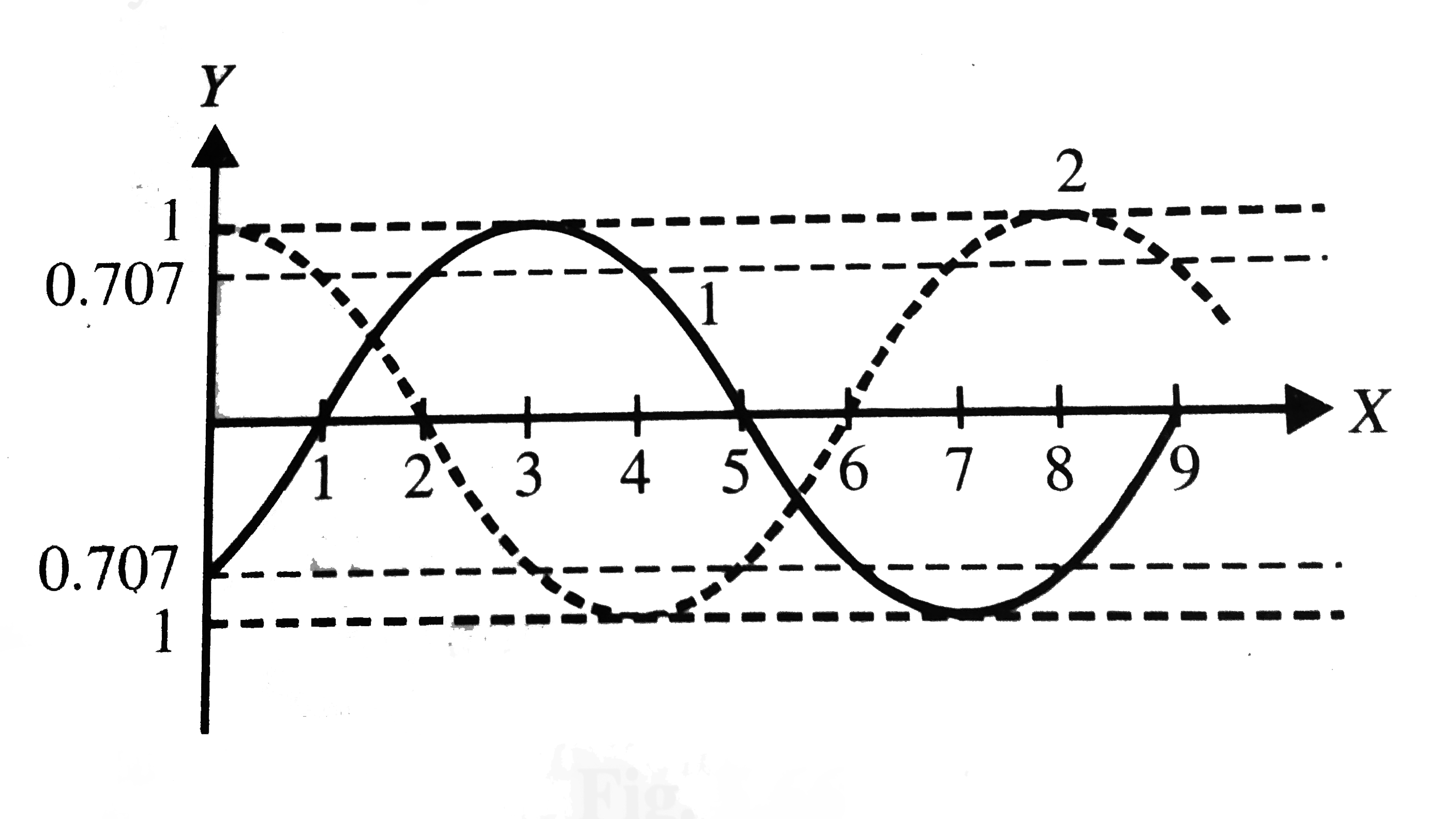
Figure. shows two snapshots of medium particle supporting a plane progressive wave travelling along positive $x -$ axos, corresponding to instants $t = 0.002 s$ and $t = 0.008 s$ , respectively shown by curves numbered $1$ and $2$ .
Assume that the interval between the two snapshots is less than the time period.

Velocity of the wave is
A $\frac{1700}{3} m / s$
B $\frac{1700}{5} m / s$
C $\frac{2500}{7} m / s$
D $\frac{2500}{3} m / s$
Question 2 - Select One
the equation of a wave travelling along the positive x - axis ,as shown in figure at t = 0 is given by

A $sin (k x - ω t + \frac{π}{6})$
B $sin (k x - ω t - \frac{π}{6})$
C $sin (ω t - k x + \frac{π}{6})$
D $sin (ω t - k x - \frac{π}{6})$
Question 3 - Select One
The equation of a wave travelling along the positive x-axis, as shown in figure at t=0 is given by :-
.
A $sin (k x - ω t + \frac{π}{6})$
B $sin (k x - ω t - \frac{π}{6})$
C $sin (ω t - k x + \frac{π}{6})$
D $sin (ω t - k x - \frac{π}{6})$

CENGAGE PHYSICS-TRAVELLING WAVES-Comprehension

Home
Ask Doubt
Profile