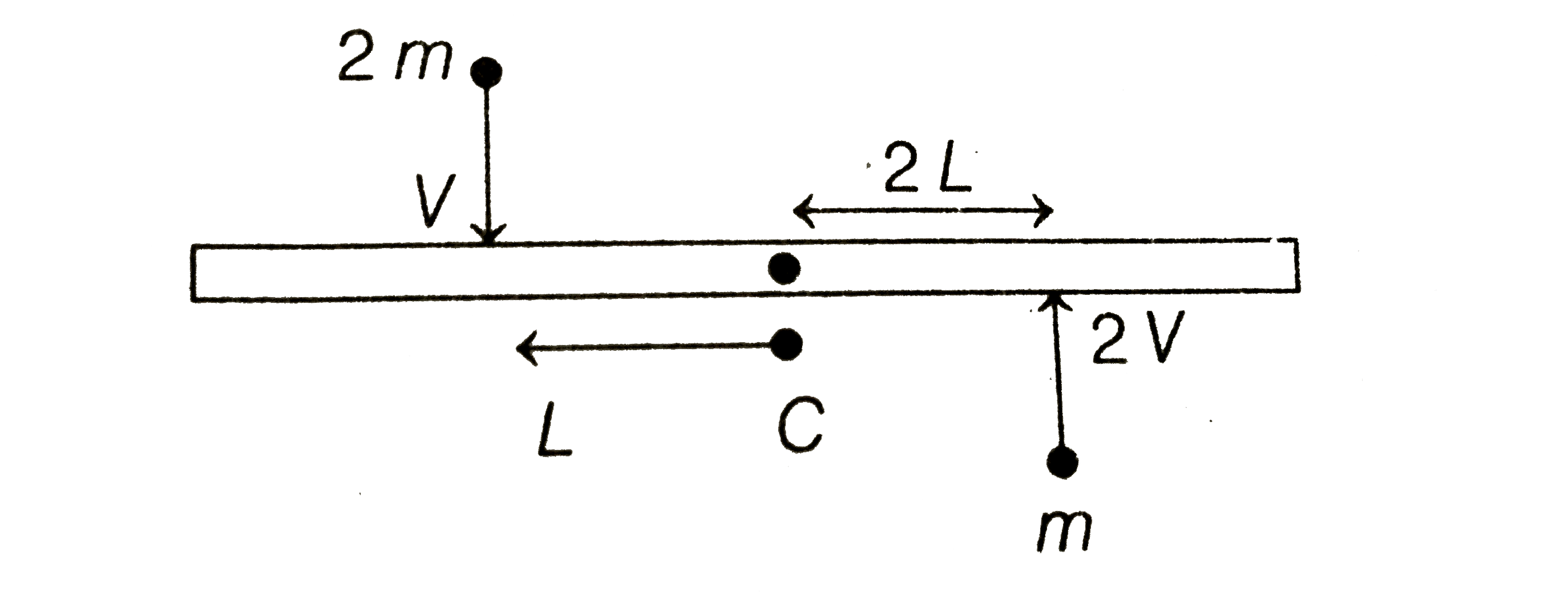
A uniform rod of length '6L' and mass '8 m' is pivoted at its centre 'C'. Two masses 'm' and '2m' with speed 2v, v as shown strikes the rod and stick to the rod. Initially the rod is at rest. Due to impact, if it rotates with angular velocity 'ω' then 'ω' will be
v5L
zero
8v6L
11v3L
The correct Answer is:A
A rod of length 6L and mass 8m is given in figure,

When two masses strike the rod, then angular momentum imparted to rod,
L1+L2=2mv(L)+m(2v)(2L)
= 6mvL
Now, after striking of masses to rod the angular momentum of comP,lete rod about the centre C,
LrodIω
where, ω = angular velocity of the rod
and I = moment of inertia of the rod.
The moment of inertia of rod
I=Ml212=8m(6L)212=24mL2
∴ M = 8m andl= 6L (given)
Now, moment of inertia of two masses after the striking to l rod,
I=2m(L)2=2mL2
and I2=m(2L)2=4mL2
∴ The net moment of the inertia about the centre of rod,
I=24mL2+2mL2+4mL2
!I=30mL2
By putting this value in Eq. (i), we get
Lrod=30ML2ω
From the law of conservation of angular momentum,
L1+L2=Lrod
!6mvL=30mL2ω
!ω=v5L
Hence, the anguler velocity of the rod is v5L
Topper's Solved these Questions
Similar Questions
A uniform rod of length l is spinning with an angular velocity ω=2vl while its centre of mass moves with a velocity v. Find the velocity of the end of the rod.
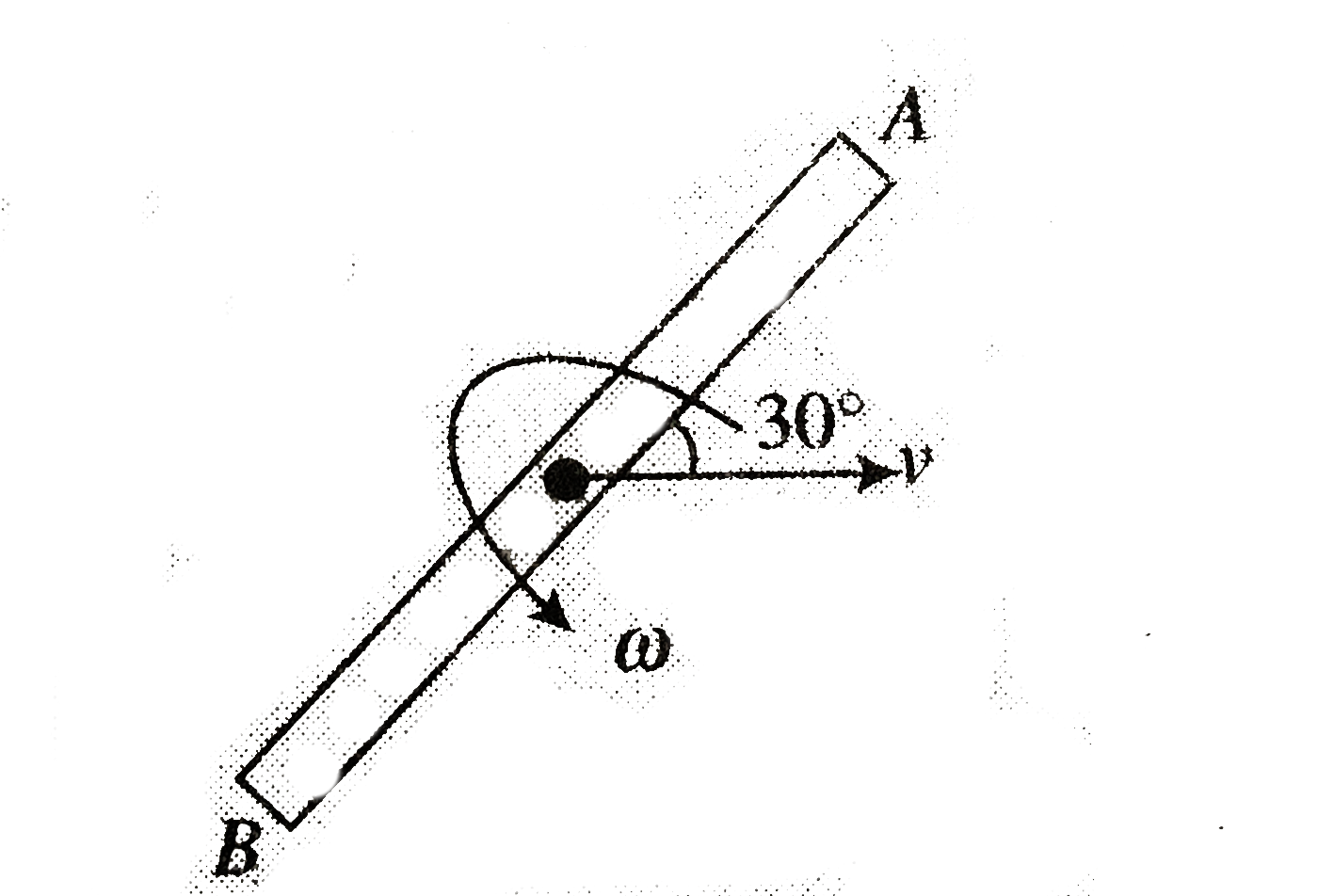
View SolutionA uniform rod of length l is spinning with an angular velocity ω=2vl while its centre of mass moves with a velocity v. Find the velocity of the end of the rod.
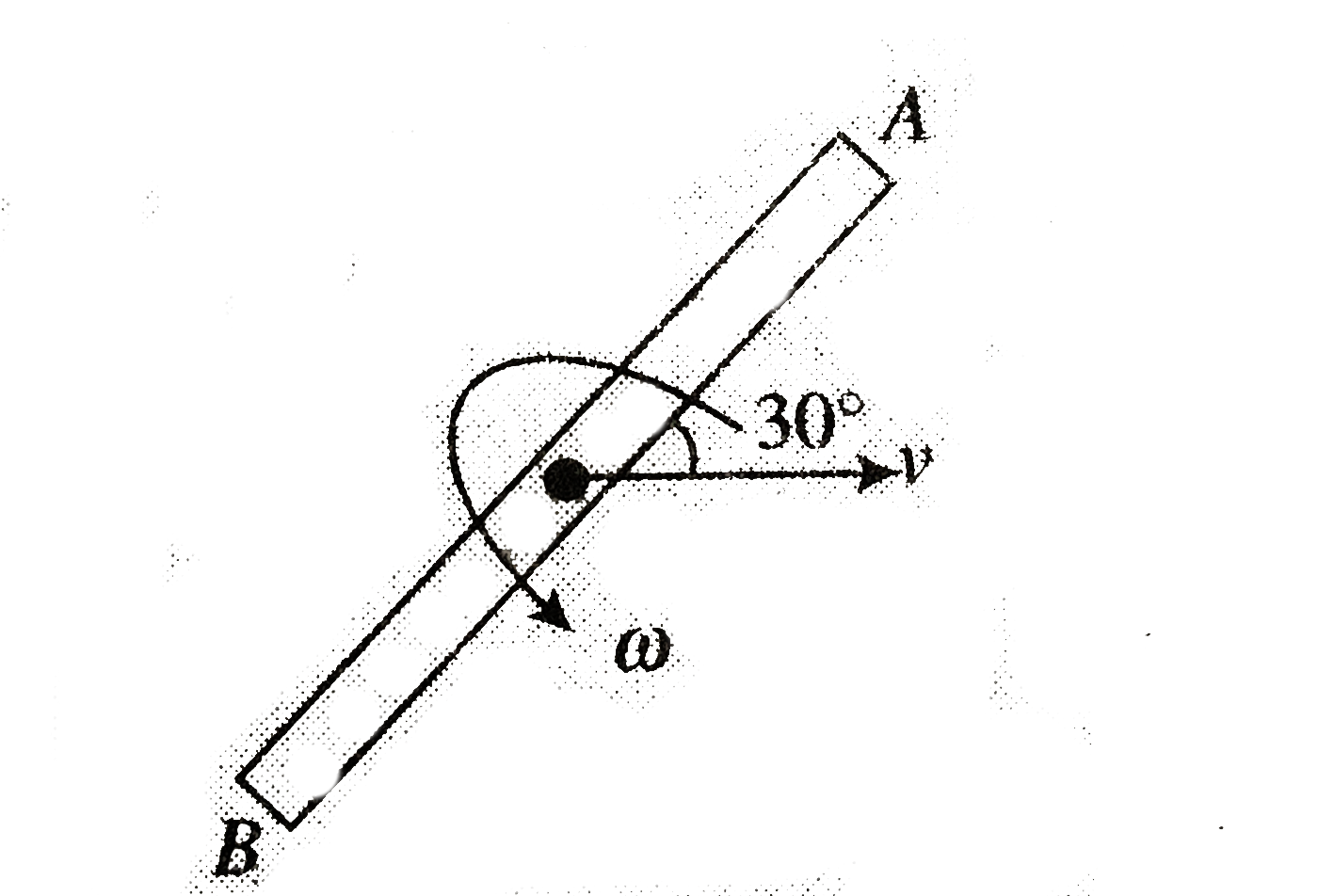
View Solution
Knowledge Check
A uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the velocity of the centre of mass C and the angular, velocity of the system about the centre of mass after the collision.A2mvM+m,3Mv(M+2m)LBMvM+m,6Mv(M+4m)LC2mvM+m,3Mv(M+4m)LDmvM+m,6Mv(M+4m)LA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision. Find the velocity of the centre of mass C of the system constituting 'the rod plus the particle'.
A2MvM−mB2mvM+mCMvM+mDmvM+mA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the angular momentum of the particle and of then about the centre of mass C before the collision.AM2mvl4(M+m)2,m2Mvl4(m+M)BM2mvl2(M+m)2,M2Mvl2(m+M)CM2mvl(M+m)2,m2Mvl(m+M)D2M2mvl(M+m)2,2m2Mvl(m+M)
A uniform rod AB of length 2l and mass 2m is suspended freely at A and hangs vertically at rest when a particle of mass m is fired horizontally with speed v to strike the rod at its mid point. If the particle is brought to rest by the impact , find : (a) the impulsive reaction at A , (b) the initial angular speed of the rod and (c) the maximum angle the rod makes with the vertical in the subsequent motion .
[mv/4 , 3v/8l , cos−1(1−3v232lg)]
View SolutionA uniform rod of length λ lies on a smooth horizontal table A particle moving on the table has a mass m and a speed v before the collision and it sticks to the rod after the collision. The rod has a mass M then find out.
The velocity of the centre of mass C and the angular velocity of the system about the centre of mass after the collision.
View SolutionA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the velocity of the particle with respect to C before the collision
View SolutionA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the velocity of the rod with respect to C before the collision
View SolutionA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the velocity of the particle with respect to C before the collision
View Solution
