Detrmine the maximum acceleration of the train in which a box lying on the floor will remain stationary given that the coefficient of static friction between the box and the train s floor is 0.15 given g=10m/s2 .
To determine the maximum acceleration of the train in which a box lying on the floor will remain stationary, we can follow these steps:
Step 1: Understand the Forces Acting on the Box
The box experiences two main forces:
- The gravitational force acting downwards, which is Fg=mg.
- The normal force acting upwards, which balances the gravitational force, N=mg.
Step 2: Identify the Frictional Force
The frictional force that prevents the box from sliding is given by:
Ff=μsN
where μs is the coefficient of static friction.
Step 3: Set Up the Equation for Maximum Frictional Force
Since the normal force N is equal to the weight of the box, we can substitute:
Ff=μsmg
Step 4: Relate Frictional Force to Acceleration
For the box to remain stationary relative to the train, the frictional force must equal the force required to accelerate the box:
Ff=ma
where a is the acceleration of the train.
Step 5: Combine the Equations
Setting the two expressions for the frictional force equal gives:
ma=μsmg
Step 6: Solve for Maximum Acceleration
We can cancel m from both sides (assuming m≠0):
a=μsg
Step 7: Substitute the Given Values
Now, substitute the values for μs and g:
- μs=0.15
- g=10m/s2
Thus, we have:
a=0.15×10=1.5m/s2
Conclusion
The maximum acceleration of the train in which the box will remain stationary is:
a=1.5m/s2
---
The box experiences two main forces:
- The gravitational force acting downwards, which is Fg=mg.
- The normal force acting upwards, which balances the gravitational force, N=mg.
The frictional force that prevents the box from sliding is given by:
Ff=μsN
where μs is the coefficient of static friction.
Since the normal force N is equal to the weight of the box, we can substitute:
Ff=μsmg
For the box to remain stationary relative to the train, the frictional force must equal the force required to accelerate the box:
Ff=ma
where a is the acceleration of the train.
Setting the two expressions for the frictional force equal gives:
ma=μsmg
We can cancel m from both sides (assuming m≠0):
a=μsg
Now, substitute the values for μs and g:
- μs=0.15
- g=10m/s2
a=0.15×10=1.5m/s2
The maximum acceleration of the train in which the box will remain stationary is:
a=1.5m/s2
Similar Questions
Determine the maximum acceleration of the truck in which a box lying on the floor of its back will remain stationary, given that the coefficient of static friction between the box and truck's floor is 0.2.
View SolutionDetermine the maximum acceleration of the truck in which a box lying on the floor of its back will remain stationary , given that the coefficient of stastic friction between the box and trucks 's floor is 0⋅2 .
View Solution
Knowledge Check
Determine the maximum acceleration of the train in , which a box lying on its floor will remain stationary, given that the coefficient of static friction between the -box and the train floor is 0.15 (given g=10m/s2)
A1m/s2B1.5m/s2C2m/s2D0Maximum acceleration of the train in which a 50 kg box lying on its floor will ramain stationary is (Given: Co-efficient of static friction between the box and the train's floor is 0.3andg=10ms−2)
A5.0ms−2B3.0ms−2C1.5ms−2D15ms−2Maximum acceleration of the train in which a box lying on its floor will ramain stationary, is
[μs=0.15,g=10m/s2]A70m/s2B10.5m/s2C7.1m/s2D1.5m/s2
A wooden box lying at rest on an inclined surface of a wet wood is held at static equilibrium by a constant force →F applied perpendicular to the incline. If the mass of the box is 1 kg, the angle of inclination is 30∘ and the coefficient of static friction between the box and the inclined plane is 0.2, the minimum magnitude of →F is (Use g=10m/s2)
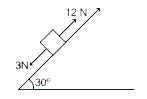
View SolutionWhen a maximum force of 3 N is applied on a body kept on rough inclined plane of shown in the figure, then body remains stationary. The maximum external force up the inclined plane that does not move the block is 12 N. The coefficient of static friction between the block and the plane is [Take, g = 10 m/s2]
View SolutionA box of mass 2 kg is placed on the roof of a car. The box would remain stationary until the car attains a maximum acceleration. Coefficient of static friction between the box and the roof of the car is 0.2 and 1g=10 ms−2.
The maximum acceleration of the car, for the box to remain stationary, is
View SolutionA box of mass 2 kg is placed on the roof of a car. The box would remain stationary untill the car attains a maximum acceleration. Coefficient of static friction between the box and the roof of the car is 0.2 and g = 10ms−2. This maximum acceleration of the car, for the box to remain stationary, is
View SolutionA block kept on a rough inclined plane ,as shown in the figure, remains at rest upto a maximum force 2N down the inclined plane. The maximum external force up the inclined plane that does not move the block is 10N.The coefficient of static friction between the block and the palbe is : [Taking g=10m/s2].

View Solution
