Discuss the bending of a cyclist while describing a curved path Find the relation for angle of bending of the cyslist
To solve the problem of the bending of a cyclist while describing a curved path and to find the relation for the angle of bending, we can follow these steps:
Step 1: Understand the Forces Acting on the Cyclist
When a cyclist is moving along a curved path, two main forces act on them:
- The gravitational force (mg) acting downwards.
- The normal force (N) acting perpendicular to the surface of the road.
Step 2: Analyze the Situation
The cyclist bends at an angle (θ) with respect to the vertical. This bending helps to balance the forces acting on the cyclist while they navigate the curve.
Step 3: Resolve the Normal Force
The normal force can be resolved into two components:
- The vertical component: Ncosθ
- The horizontal component: Nsinθ
Step 4: Apply Newton's Second Law
1. Vertical Forces: The vertical component of the normal force must balance the weight of the cyclist:
Ncosθ=mg
2. Horizontal Forces: The horizontal component of the normal force provides the necessary centripetal force to keep the cyclist moving in a circular path:
Nsinθ=mv2r
Step 5: Find the Relation for Angle of Bending
To find the relation for the angle of bending (θ), we can divide the two equations obtained from the vertical and horizontal forces:
NsinθNcosθ=mv2rmg
This simplifies to:
tanθ=v2rg
Step 6: Conclusion
The relation for the angle of bending of the cyclist while describing a curved path is:
θ=tan−1(v2rg)
When a cyclist is moving along a curved path, two main forces act on them:
- The gravitational force (mg) acting downwards.
- The normal force (N) acting perpendicular to the surface of the road.
The cyclist bends at an angle (θ) with respect to the vertical. This bending helps to balance the forces acting on the cyclist while they navigate the curve.
The normal force can be resolved into two components:
- The vertical component: Ncosθ
- The horizontal component: Nsinθ
1. Vertical Forces: The vertical component of the normal force must balance the weight of the cyclist:
Ncosθ=mg
Nsinθ=mv2r
To find the relation for the angle of bending (θ), we can divide the two equations obtained from the vertical and horizontal forces:
tanθ=v2rg
The relation for the angle of bending of the cyclist while describing a curved path is:
θ=tan−1(v2rg)
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Advanced problems for competitions
20 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISES
40 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Curiosity Question
1 Video
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension
4 Videos
- MATHEMATICAL TOOLSBOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks
5 Videos
Similar Questions
Knowledge Check
The inward bending of a cyclist while turning is due to
AGet reaction forceBGet suitable centripetal forceCMinimise frictional forceDAll of theseA cyclist bends while taking turn to
Areduce friction,Bgenerate required centripetal forceCreduce apparent weight,Dreduce speed. .A cyclist bends while taking turn to
Areduce frictionBgenerate required centripetal forceCreduce apparent weightDreduce speed
Why does a cyclist lean to one side while going along a curve ? In what direction does he lean ?
View SolutionWhy does a cyclist lean inward when moving along a curved path?Determine the angle through which a cyclist bends from the vertical while negotiating a curve.
View SolutionBending OF Cyclist || Banking OF Roads
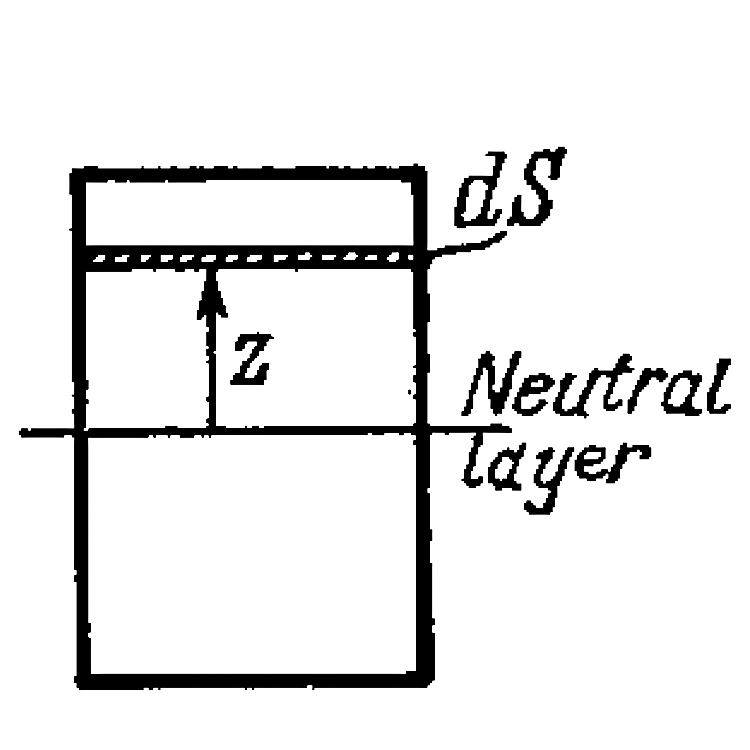
View SolutionThe bending of an elastic rod is described by the elastic curve passing through centres of gravity of rod's cross-sections. At small bendings the equation of this curve takes the form
N(x)=EId2ydx2,
where N(x) is the bending moment of the elastic forces in the cross-section corresponding to the x coordinate, E is Young's modulus, I is the moment of inertia of the cross-section relative to the axis pasing through the neutral layer (I=∫z2dS, figure)
Suppose one end of a steel rod of a square cross-section with side a is embedded into a wall, the protruding section being of length l (figure). Assuming the mass of the rod to be negligible, find the shape of the elastic curve and the deflection of the rod λ, if its end A experiences
(a) the bending moment of the couple N0, ,
(b) a force F oriented along the y axis.

View SolutionThe relation tanθ=v2/rg gives the angle of banking of the cyclist going round the curve . Here v is the speed of the cyclist , r is the radius of the curve , and g is the acceleration due to gravity . Which of the following statements about the relation is true ?
View Solution