The bending of an elastic rod is described by the elastic curve passing through centres of gravity of rod's cross-sections. At small bendings the equation of this curve takes the form
$N (x) = E I \frac{d^{2} y}{{d x}^{2}}$ ,
where $N (x)$ is the bending moment of the elastic forces in the cross-section corresponding to the x coordinate, E is Young's modulus, I is the moment of inertia of the cross-section relative to the axis pasing through the neutral layer $(I = \int z^{2} d S$ , figure)

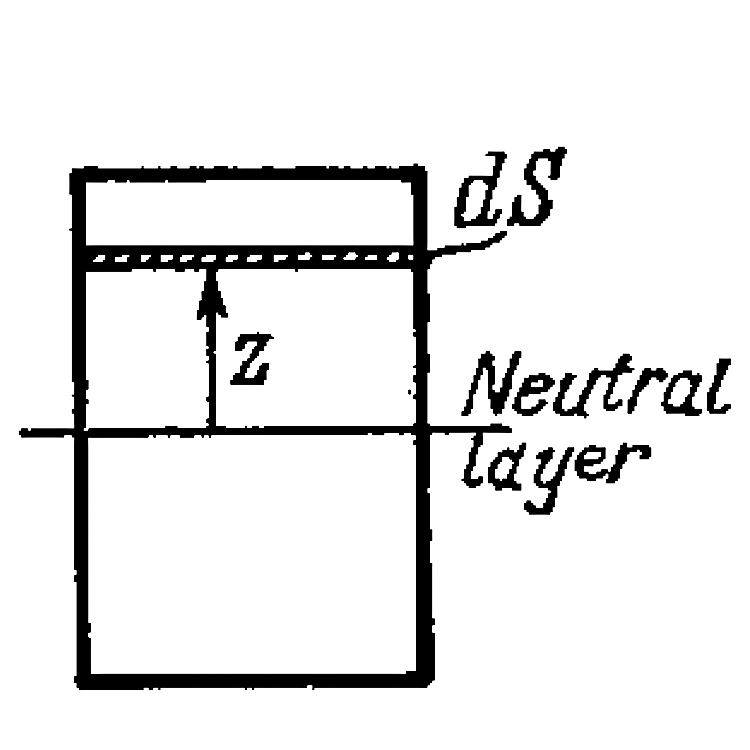
Suppose one end of a steel rod of a square cross-section with side a is embedded into a wall, the protruding section being of length l (figure). Assuming the mass of the rod to be negligible, find the shape of the elastic curve and the deflection of the rod $λ$ , if its end A experiences
(a) the bending moment of the couple $N_{0}$ , ,
(b) a force F oriented along the y axis.

Text Solution

Verified by Experts

We use the equation given above and use the result that when y is small
$\frac{1}{R} \approx \frac{d^{2} y}{{d x}^{2}}$ . Thus, $\frac{d^{2} y}{{d x}^{2}} = \frac{N (x)}{E I}$
(a) Here $N (x) = N_{0}$ is a constant. Then integration gives,
$\frac{d y}{d x} = \frac{N_{0} x}{E I} + C_{1}$
But $(\frac{d y}{d x}) = 0$ for $x = 0$ , so $C_{1} = 0$ . Integrating again,
$y = \frac{N_{0} x^{2}}{2 E I}$
where we have used $y = 0$ for $x = 0$ to set the constant of integration at zero. This is the equation of a parabola. The sage of the free end is
$λ = y (x = l) = \frac{N_{0} l^{2}}{2 E l}$
(b) In this case $N (x) = F (l - x)$ because the load F at the extremely is balanced by a similar force at F directed upward and they constitute a couple. Then
$\frac{d^{2} y}{{d x}^{2}} = \frac{F (l - x)}{E I}$ .
Integrating, $\frac{d y}{d x} = \frac{F (l x - x^{/} 2)}{E I} + C_{1}$
As before $C_{1} = 0$ . Integrating again, using $y = 0$ for $x = 0$
$y = \frac{F (\frac{l x^{2}}{2} - \frac{x^{3}}{6})}{E I}$ here $λ = \frac{F l^{3}}{3 E l}$
Here for a square cross section
$I = a / 2 \int - a / 2 z^{2} a d z = a^{4} / 12$ .

Updated on:21/07/2023