Calculate the height upto which an insect can crawl up a fixed bowl in the from of a hemisphere of radius r Given coefficient of friction =1√3
 .
.
B is botton of the bowl of radius r = OB . The insect can crawl up the bowl From B to P through a height
BA=h
As is clear from R=Wcosα
and F=Wsinα where W is weight of insect and F is force of friction
μ=FR=WsinαWcosα=tanα1√3
In △OPA,tanα=PAOA
∴tanα=√r2−Y2y=1√3
r2−yyy2=13
y2=3r24,y=√3r2
h=BA=OB=OB−OA=r−y
h=r−√3r2=0.134r
h=13.4% of r.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - EXERCISES
40 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Additional Examples / Exercises
6 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Short Answer Questions
26 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension
4 Videos
- MATHEMATICAL TOOLSBOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks
5 Videos
Similar Questions
Calculate the height upto which an insect can crawl up a fixed bowl in the form of a hemisphere of radius r. Given coefficient of friction is 1/√3.
View SolutionWhat is the maximum velocity with which a vehicle can negotiate a turn of radius r safely when coefficient of friction between tyres and road is μ ?
View Solution
Knowledge Check
If you want to pile up sand onto a circular area of radius R. The greatest height of the sand pile that can be created without spilling the sand onto the surrounding. Area, if μ is the coefficient of friction between sand particle is:
Aμ2RBμRCRDRμSand is piled up on a horizontal ground in the form of a regualr cone of a fixed base of radius R.The coefficient of static friction between sand layers is μ.The maximum volume of sand that can be piled up, without the sand slipping on the surface is
AμR33πBμR3πCπR33μDμπR33A particle is placed at rest inside a hollow hemisphere of radius R. The coefficient of friction between the particle and the hemisphere is μ=1√3. The maximum height up to which the particle can remain stationary is
AR2B(1−√32)RC√32RD3R8
An engine of one metric ton is going up an inclined plane of slope 1 in 2 at the rate of 36kmh−1 If the coefficient of friction is 1/√3 calculate the power of the engine in k W .
View SolutionAn insect is at the bottom of a hemispherical ditch of radius 1 m. It crawls up the ditch but starts slipping after it is at height h from the bottom . If the coefficient of friction between the ground and the insect is 0.75 , then h is : (g=10ms−2)
View SolutionA uniform cylinder of radius r and radius of gyration is kept on a fixed wedge of inclination θ. When we push the wedge with a constant acceleration a, the cylinder rolls up the wedge. Find coefficient of friction between the cylinder and wedge.
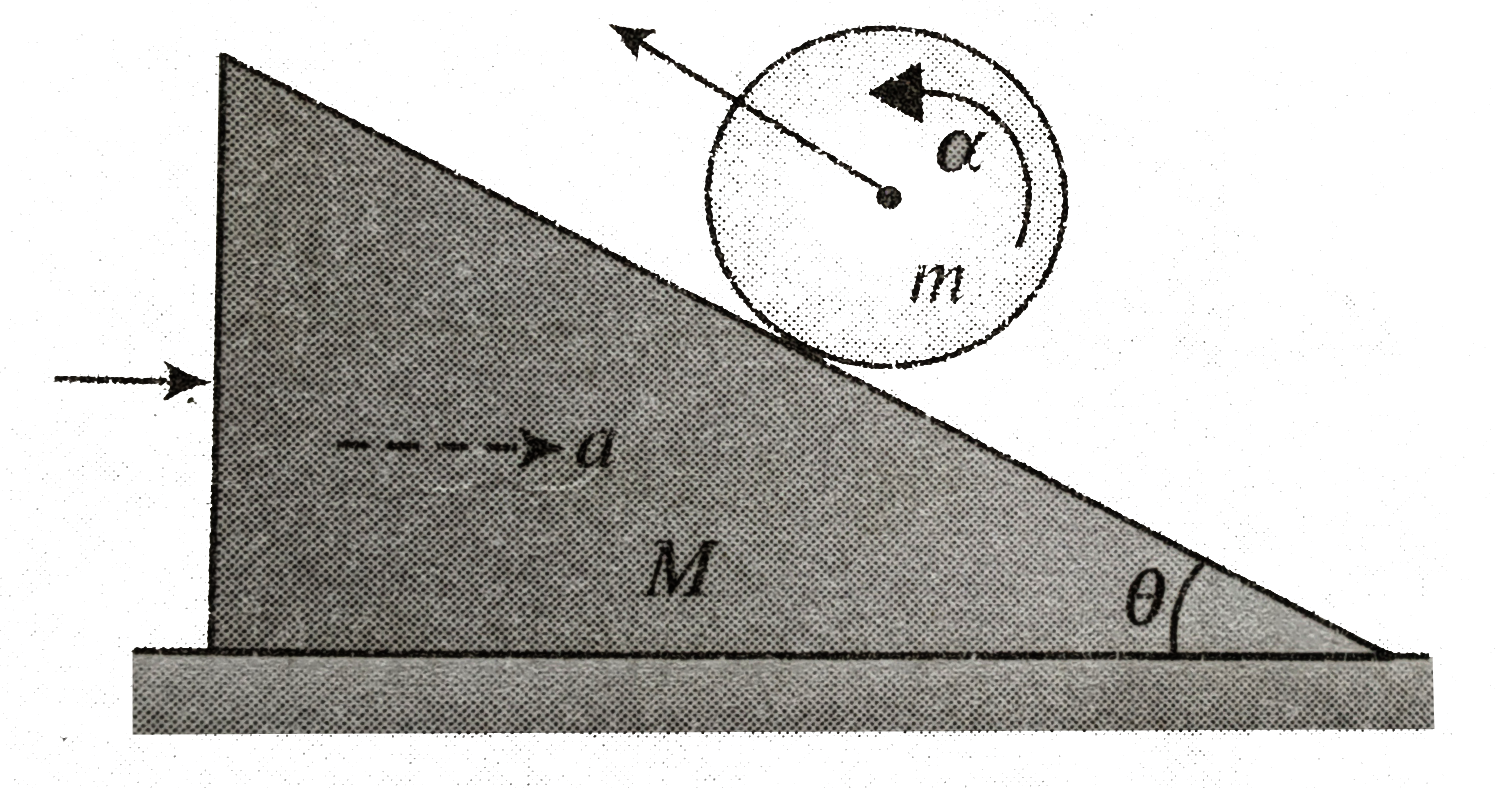
View SolutionThe greatest height h of the sand pile that can be erected without spilling the sand onto the surrounding circular area of radius R (If μ is the coefficient of friction between sand particles) is
View SolutionA partical is placed at rest inside a hollow hemisphare of redius R. The coefficient of friction between the partical and the homisphere is μ=1√3. The maximum height up to which the partical can remain stationary is
View Solution
