Find the centre of mass of a uniform triangula lamina.
To find the center of mass of a uniform triangular lamina, we can follow these steps:
Step 1: Understand the Geometry of the Triangle
We start by considering a uniform triangular lamina with vertices A, B, and C. For simplicity, we can place the triangle in a coordinate system. Let’s assume:
- Vertex A is at (0, 0)
- Vertex B is at (b, 0)
- Vertex C is at (c, h) where c is the x-coordinate of vertex C and h is the height of the triangle.
Step 2: Divide the Triangle into Strips
To find the center of mass, we can divide the triangular lamina into horizontal strips. Each strip will be parallel to the base AB.
Step 3: Determine the Center of Mass of Each Strip
For a small strip of height dy at a height y from the base, the width of the strip can be determined using similar triangles. The width of the strip at height y can be calculated as:
Width=b−ch⋅y+c
The center of mass of each strip will be at its midpoint, which is at y and the x-coordinate can be calculated based on the width.
Step 4: Find the Overall Center of Mass
The center of mass of the entire triangle can be found by averaging the x-coordinates and y-coordinates of all the strips. The x-coordinate of the center of mass (x_cm) can be calculated as:
xcm=1A∫xdA
where A is the area of the triangle and dA is the differential area element.
The y-coordinate of the center of mass (y_cm) can be calculated similarly:
ycm=1A∫ydA
Step 5: Use the Formula for the Centroid of a Triangle
For a uniform triangular lamina, the center of mass (or centroid) can be directly calculated using the formula:
xcm=xA+xB+xC3
ycm=yA+yB+yC3
Substituting the coordinates of the vertices:
- xA=0,yA=0
- xB=b,yB=0
- xC=c,yC=h
We get:
xcm=0+b+c3=b+c3
ycm=0+0+h3=h3
Conclusion
Thus, the center of mass of the uniform triangular lamina is located at:
(b+c3,h3)
We start by considering a uniform triangular lamina with vertices A, B, and C. For simplicity, we can place the triangle in a coordinate system. Let’s assume:
- Vertex A is at (0, 0)
- Vertex B is at (b, 0)
- Vertex C is at (c, h) where c is the x-coordinate of vertex C and h is the height of the triangle.
To find the center of mass, we can divide the triangular lamina into horizontal strips. Each strip will be parallel to the base AB.
For a small strip of height dy at a height y from the base, the width of the strip can be determined using similar triangles. The width of the strip at height y can be calculated as:
Width=b−ch⋅y+c
The center of mass of the entire triangle can be found by averaging the x-coordinates and y-coordinates of all the strips. The x-coordinate of the center of mass (x_cm) can be calculated as:
xcm=1A∫xdA
where A is the area of the triangle and dA is the differential area element.
ycm=1A∫ydA
For a uniform triangular lamina, the center of mass (or centroid) can be directly calculated using the formula:
xcm=xA+xB+xC3
ycm=yA+yB+yC3
- xA=0,yA=0
- xB=b,yB=0
- xC=c,yC=h
xcm=0+b+c3=b+c3
ycm=0+0+h3=h3
Thus, the center of mass of the uniform triangular lamina is located at:
(b+c3,h3)
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE B
2 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE C
2 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - SOLVED EXAMPLES TYPE A
2 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
Knowledge Check
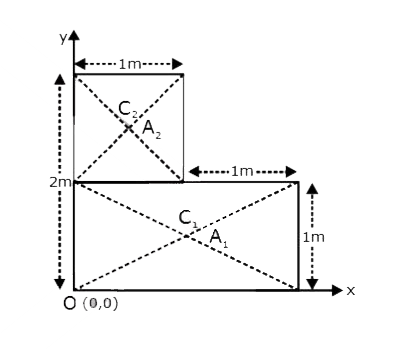
Find the centre of mass of a uniform I - shaped lamina (a thin flat plate) with dimensions as shown in figure. The mass of lamina is 3 kg
 A(1,1)B(32,12)C(56,56)D(32,32)
A(1,1)B(32,12)C(56,56)D(32,32)
Find the centre of mass of a uniform triangula lamina.
View SolutionFind the centre of mass of a uniform L shaped lamina (a thin flat plate) with dimension as shown in Fig. The mass of the lamina is 3kg.

View SolutionFind the centre of mass of as uniform L -shaped lamina (a thin flat plate) with dimensions as shown in figure. The mass of lamina is 3kg.

View SolutionFind the centre of mass of a uniform L-shaped lamina (a thin flat plate) origine with dimensions as shown in figure. The mass of lamina is 3 kg.
View SolutionFind the centre of mass of a uniform solid cone.
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionFind the centre of mass of a uniform plate having semicircular inner and outer boundaries of radii R1andR2.
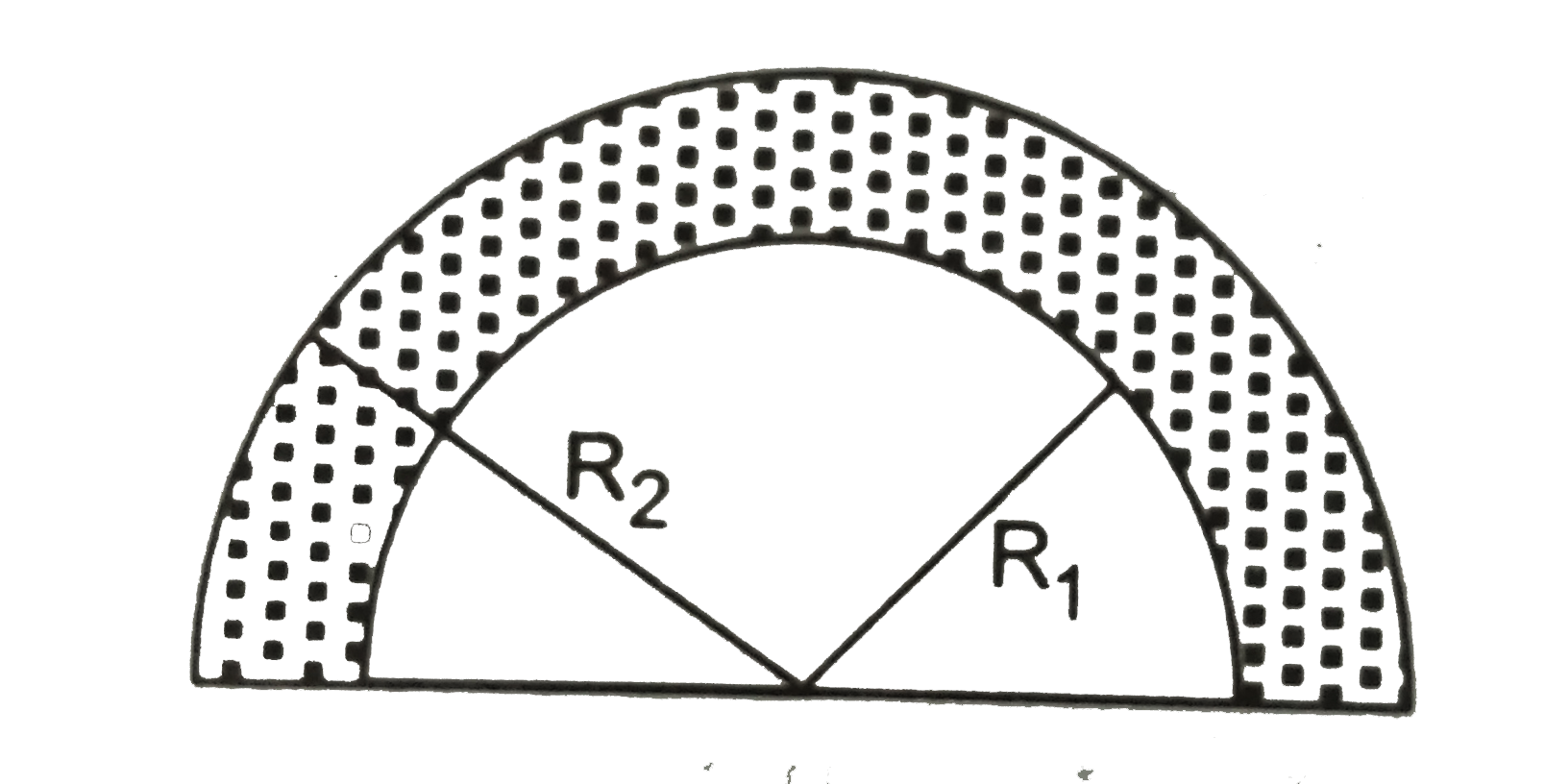
View Solution
