Figure shows for containers of olive oil. The pressure at depth h is
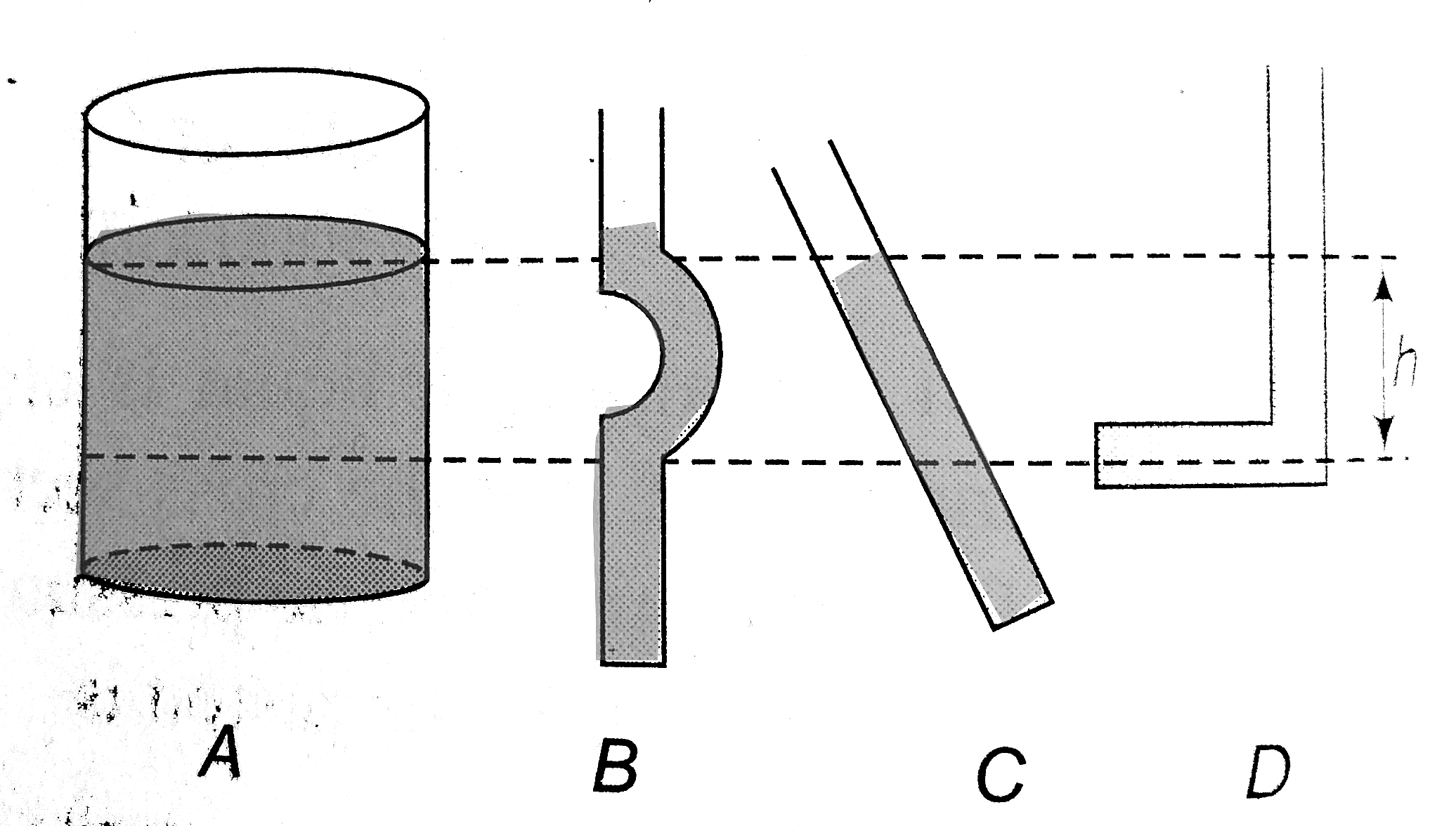
least in B and C both
greatest in A
greatest in D
equal in all the containers
The correct Answer is:D
(d) P=hρg
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Buoyancy And Floatation40videos
FLUID MECHANICS
- BOOK - A2ZCHAPTER - FLUID MECHANICSEXERCISE - Continuity Equation And Bernoulli'S Theorem32videos
FLUID MECHANICS
- BOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Chapter Test30videos
GENERAL KINEMATICS AND MOTION IN ONE DIMENSION
Similar Questions
Four container of honey are shown in the figure. The pressure at depth h is
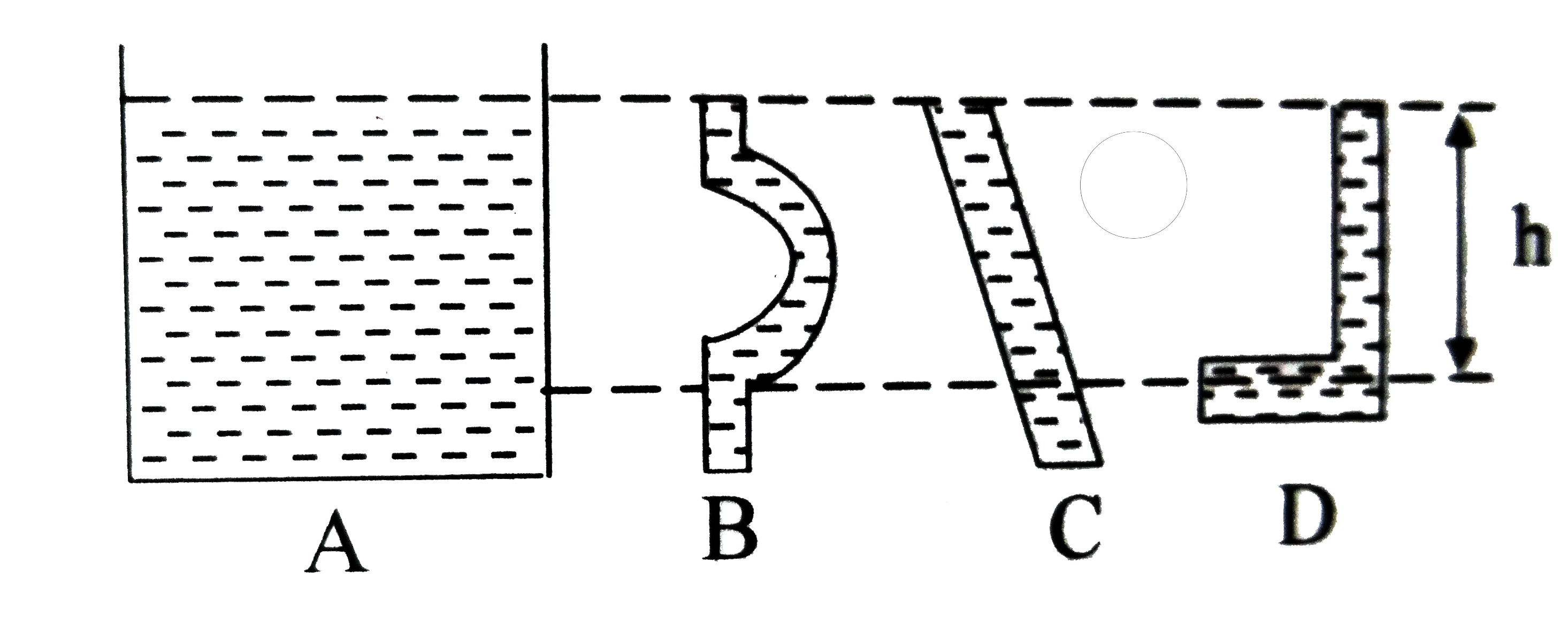 View Solution
View SolutionFigure (b) shows the variation of pressure (p) with depth (h) along a vertical wall supporting two liquids of different densities as shown in Fig. (a). Identify the correct variation of pressure in the liquid of density ρ2.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Consider a liquid at rest in a container which opens into atmosphere. At any point A at a depth h below the surface of the liquid,
(i) the gauge pressure at point A is ρgh.
(ii) the gauge pressure at point a is Pa+ρgh.
(iii) The pressure at point A is ρgh.
(iv) The pressure at point A is Pa +ρgh.
The correct alternative isA(i) and (iii)B(ii) and (iv)C(iii) and (iv)D(i) and (iv) - Question 2 - Select One
The top surface of an incompressible liquid is open to the atmosphere. The pressure at a depth h1 below the surface is p1 . What is the pressure p2 at depth h2=2h1 compare with p1 ?
Ap2>2p1Bp2=2p1Cp2<2p1Dp2=p1 - Question 3 - Select One
Estimate the pressure deep inside the sea at a depth h below the surface. Assume that the density fo water is ρ0 at sea level and its bulk modulus is B.P0 is the atmosphere pressure at sea level P is the pressure at depth 'h'
AP=P0−BIn(1−ρ0ghB)BP=P0+BIn(1−ρ0ghB)CP=P0−BIn(1+ρ0ghB)DP=P0+BIn(1+ρ0ghB)
What do you mean by Gauge pressure at a depth h inside the liquid?
View SolutionOil enters the bend of a pipe in the horizontal plane with velocity 4ms−1 and pressure 280×103Nm−2 as shown in the figure. Find the pressure of oil at the point Q in (kNm2)
(Take specific gravity of oil as 0.9 and sin37∘=0.6.)
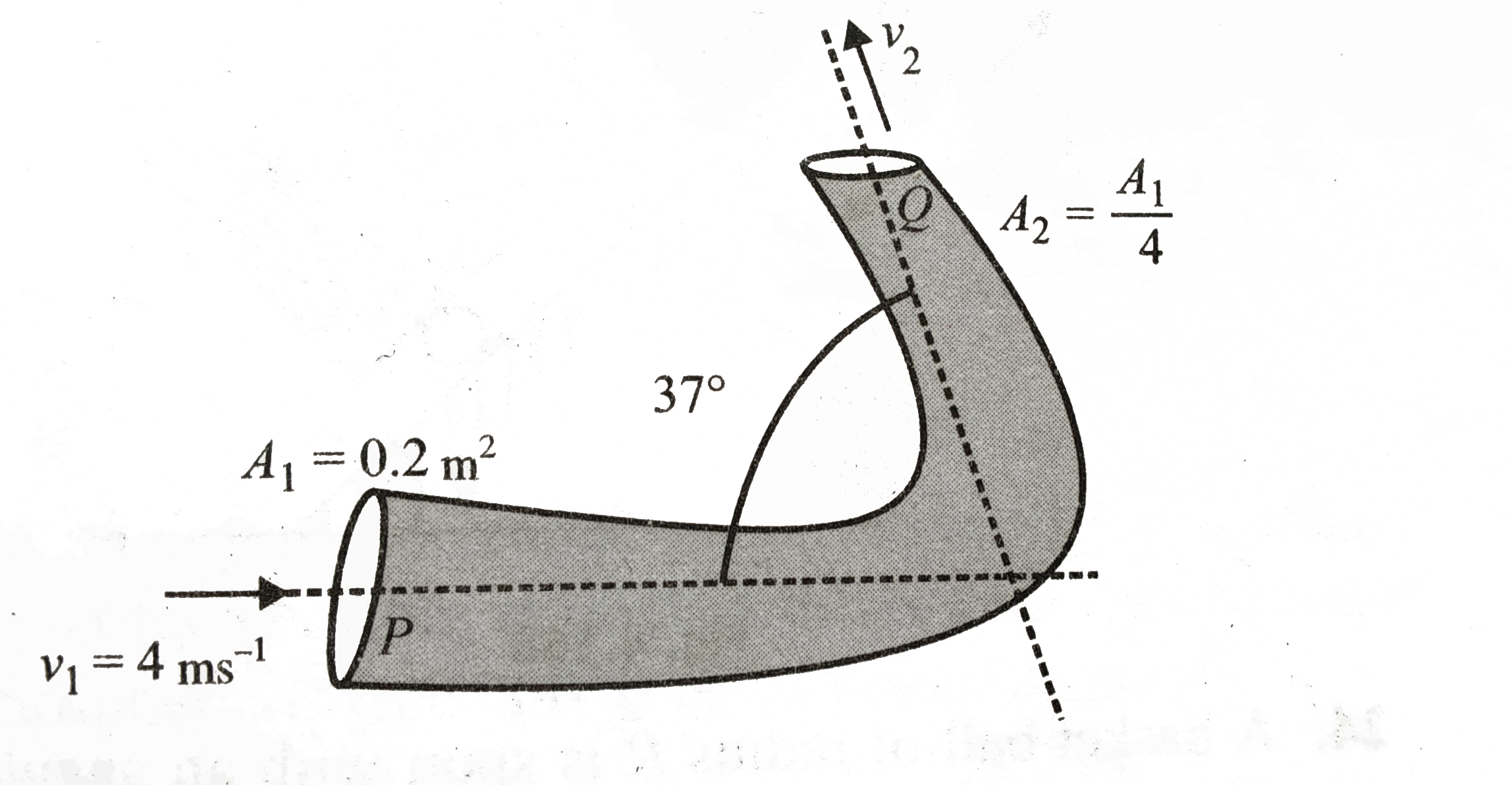 View Solution
View SolutionEssential oils contain
View SolutionA container shown in figure contains a liquid to depth H, and of density ρ The gauge pressure at point P is :
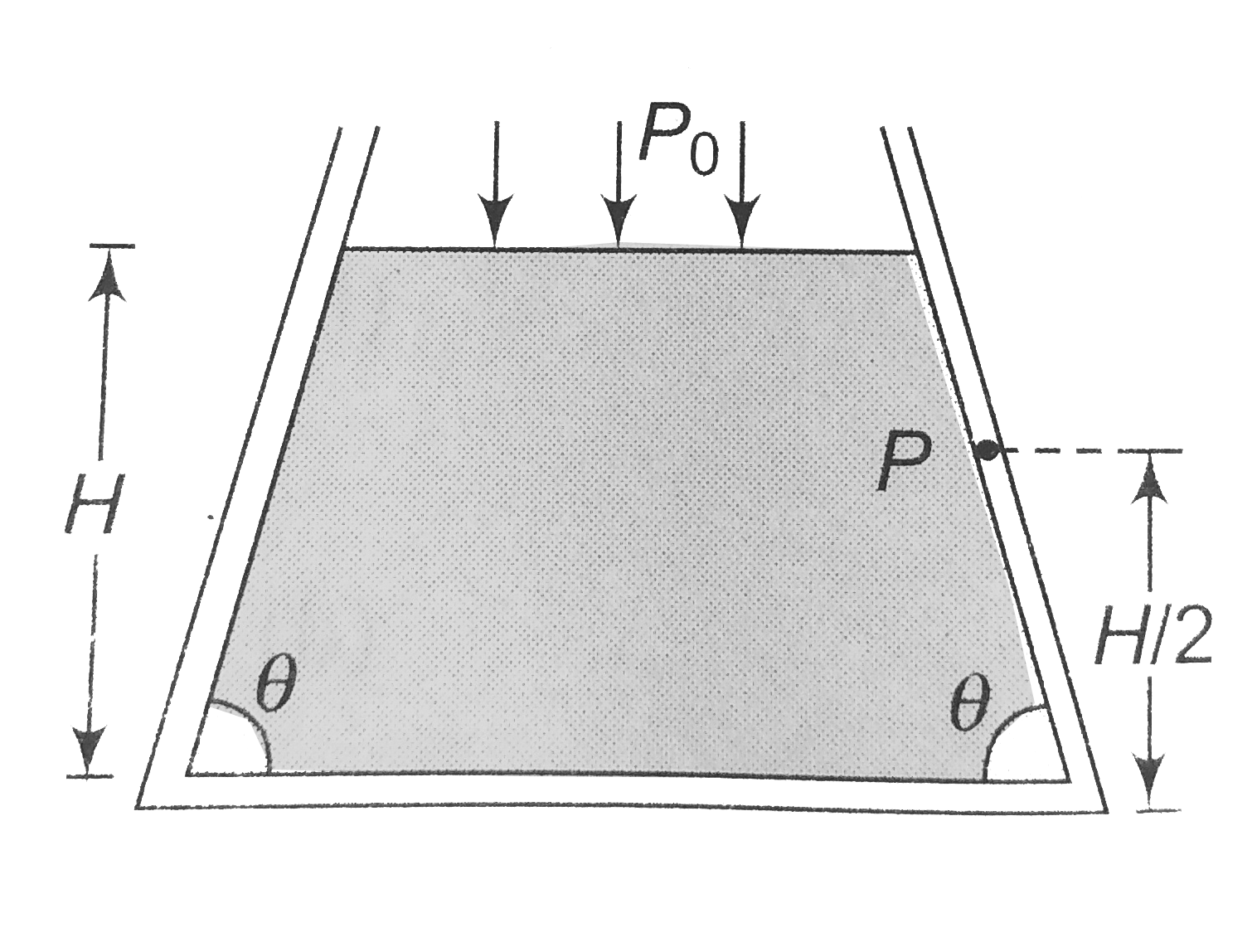 View Solution
View SolutionIn the figure shown, a light container is kept on a horizontal rough surface of coefficient of friction μ=ShV. A very small hole of area S is made at depth 'h'. Water of volume 'V' is filled in the container. The friction is not sufficient to keep the container at rest. The acceleration of the container initially is
 View Solution
View Solution

