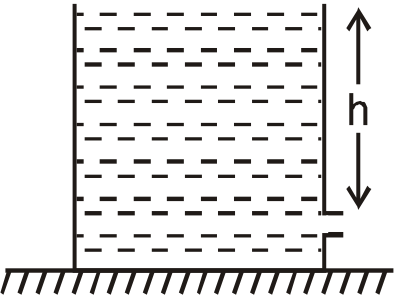
In the figure shown, a light container is kept on a horizontal rough surface of coefficient of friction μ=ShV. A very small hole of area S is made at depth 'h'. Water of volume 'V' is filled in the container. The friction is not sufficient to keep the container at rest. The acceleration of the container initially is
VShg
g
zero
ShVg
Let the density of water be ρ, then the force by escaping liquid on container =ρS(√2gh)2
∴ acceleration of container a=2ρSgh−μρVgρV=(2ShV−μ)g
Now μ=ShV∴A=ShVg
Topper's Solved these Questions
Similar Questions
A radioactive element decays by β−emission. A detector records n beta particles in 2s and in next 2s it records 0.75n beta particles. Find mean life correct to nearest whole number. Given ln |2|=0.6931, ln |3|=1.0986.
View SolutionWhen p−n junction diode is forward biased then
View SolutionWater is filled in a container upto height 3m. A small hole of area 'a' is punched in the wall of the container at a height 52.5 cm from the bottom. The cross sectional area of the container is A. If a//A=0.1 then v^2 is (where v is the velocity of water coming out of the hole)
View SolutionAn empty container has a circular hole of radius r at its bottom. The container is pused into water very slowly as shown. To what depth the lower surface of container (from surface of water) can be pushed into water such that water does not flow into the container?
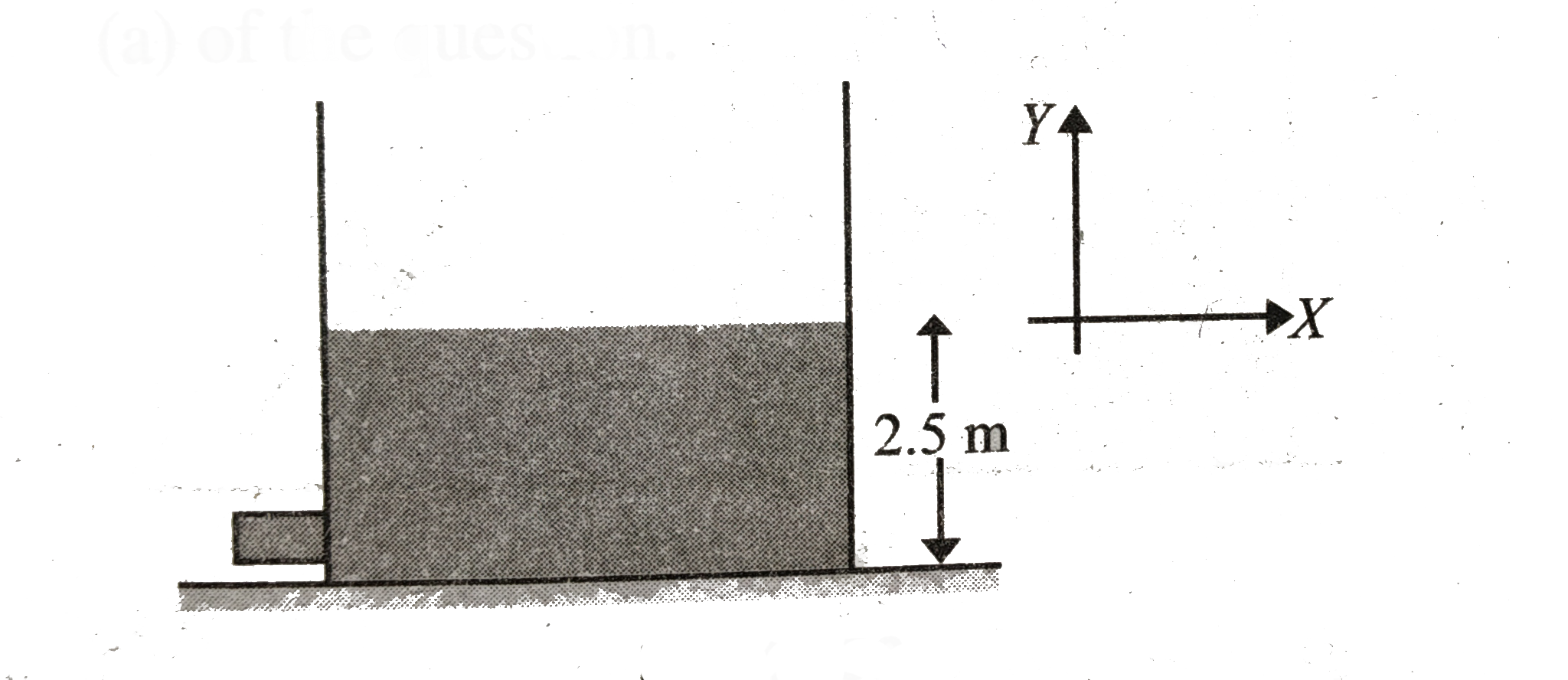
View SolutionWater is filled to a height of 2.5 m in a container lying rest on a horizontal surface as shown in the figure. The bottom of the container is square shaped of side sqrt(3) and its top is open. There is a very small hole in the vertical wall of the container near the bottom. A small cap closes the hole. The hole gets opened when pressure near the bottom becomes more than 4 xx 10^(4) pascal a, What minimum acceleration can be given to the container in the positive x -direction so that the cap will come out of the hole? Assume water does not spill out of container when the container accelerates. Find the velocity of efflux as soon as the container starts moving with the acceleration calculated in part a. of the question.
View SolutionA container filled with liquid up to height h is placed on a smooth horizontal surface. The container is having a small hole at the bottom. As the liquid comes out from the hole, the container moves in a backward direction with acceleration a and finally, when all the liquid is drained out, it acquires a velocity v . Neglect mass of the container. In this case
View SolutionLiquid of density rho is filled in the container of negligible mass having area A. Area of hole 'a' is very small (alt lt A) . Co-efficient of friction between container and surface is equal to ((a)/(A)) . As liquid is coming out of vessel, instantaneous acceleration of the container.
View SolutionAn empty container has a circular hole of radius r at its bottom. The container is pushed into water very slowly as shown. To what depth the loweer surface of container (from surface of water) can be pushed into water such that water does not flow into the container ?

(Surface tension of water =T, density of water =ρ)
View SolutionA container contains three liquds as shown in the figure. The specific gravity of liquid 1 and liquid 2 are 0.8 and 1.2 respectively. A small hole having area 10^(-6) m^(2) at point P is as shown in the figure. Initially hole is closed. The cross-sectional area of container is 50 cm^(2) . assume all liquids ae ideal and neglect any type of friction between liquid and container. (Given that g=9.8 m//s^(2) and h=28 cm ) The value of h_(2)//h_(1) is
View SolutionA hole is made at a depth h on the wall of a container containing a liquid. The velocity of efflux of the liquid through the hole is
View SolutionTwo holes are made at a depth of h and 5 h from the water surface in a water container kept on a horizontal surface as shownn in figure. For which value of r, equalt amount of water will come out of the two holes? Here, l is the length of side of the square hole and r is the radius of circular hole.
View SolutionWater is filled in a container upto height H as shown in the figure . A small hole is board on the surface of a container at the depth h from the surface of water . What will be the distance of a point along the horizontal where the jet of the water strikes the ground ? For which value of h will this distance be maximum ? Also find this maximum distance.

View SolutionA tank, which is open at the top, contains a liquid up to a height H. A small hole is made in the side of the tank at a distance y below the liquid surface. The liquid emerging from the hole lands at a distance x from the tank :

View SolutionA container contains three liquds as shown in the figure. The specific gravity of liquid 1 and liquid 2 are 0.8 and 1.2 respectively. A small hole having area 10^(-6) m^(2) at point P is as shown in the figure. Initially hole is closed. The cross-sectional area of container is 50 cm^(2) . assume all liquids ae ideal and neglect any type of friction between liquid and container. (Given that g=9.8 m//s^(2) and h=28 cm ) The value of h_(2)//h_(1) is
View SolutionWater is filled to a height of 2.5m in a container lying rest on a horizontal surface as shown in the figure. The bottom of the container is square shaped of side √3 and its top is open. There is a very small hole in the vertical wall of the container near the bottom. A small cap closes the hole. The hole gets opened when pressure near the bottom becomes more than 4×104 pascal
a, What minimum acceleration can be given to the container in the positive x-direction so that the cap will come out of the hole? Assume water does not spill out of container when the container accelerates.
Find the velocity of efflux as soon as the container starts moving with the acceleration calculated in part a. of the question.
View Solution
