In a region, potential energy varies with X as $U (x) = 30 - {(x - 5)}^{2}$ Joule, where x is in meters. A particle of mass 0.5 kg is projected from x=11 m towards origin with a velocity 'u' . u is the minimum velocity, so that the particle can reach the origin. (x=0). find the value of u/2 in meter/second. (Take $\sqrt{44} = 6.5$ )

Video Solution

Text Solution

Verified by Experts

Draw U v/s x graph. There is a maxima of potential energy between x = 11 to x = 0. So to bring the particle from x = 11 to x = 0, the particle has to cross the maxima (x = 5) and to just cross the point x = 5, velocity at x = 5 should be $0^{+}$

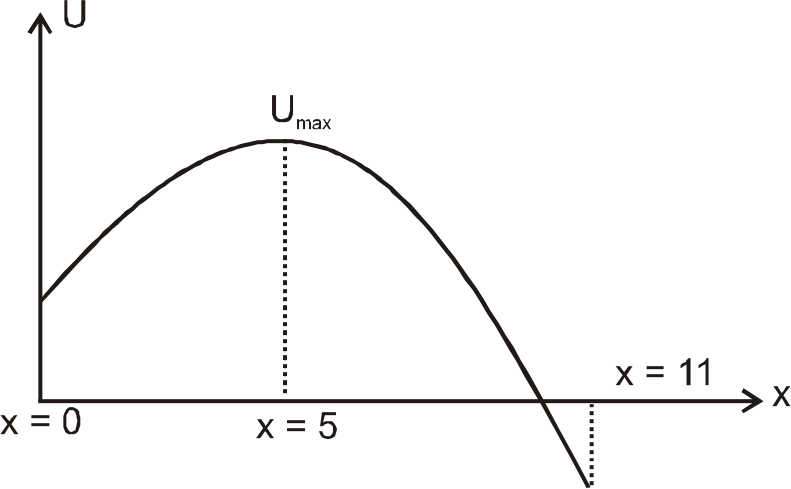
$\Rightarrow$ Applying energy conservation between x=11 to x=5
$k_{i} + U_{i} = k_{f} + U_{f}$
$\frac{1}{2} (0.5) u^{2} + (30 - {(11 - 5)}^{2}) = 0^{+} + (30 - {(5 - 5)}^{2})$
$u = 12 m / sec \Rightarrow \frac{u}{2} = 6 m / sec$

Updated on:21/07/2023

The potential energy of a particle is given by formula $U = 100 - 5 x + 100 x^{2}, w h e r e$ U and are .
AAt $0.05 m$ form the origin is 50 ms^(2).
BAt 0.05 m from the mean position is 100 ms^(2).
CAt 0.05 m feom the origin is 150 ms^(2).
DAt $0.05 m$ from the mean position is $200 m s^{- 1}$
The potential energy of particle of mass 1kg moving along the x-axis is given by $U (x) = 16 (x^{2} - 2 x)$ J, where x is in meter. Its speed at x=1 m is $2 m / s$ . Then,
Athe motion of the particle is uniformly accelerated
Bthe motion of the particle is periodic but not simple harmonic.
Cthe motion of the particle is simple harmonic having equilibrium position at x=1m
Dkinetic energy of the particle is conserved
The potential energy of a particle of mass 1 kg moving in X-Y plane is given by $U = (12 x + 5 y)$ joules, where x an y are in meters. If the particle is initially at rest at origin, then select incorrect alternative :-
AMagnitude of its acceleration is $13 m / s^{2}$
BIt never crosses y-axis
CIt never crosses x-axis
DIt moves in a circle.