A particle of mass m collides elastically with the pan of mass (M = 2m) of a spring balance, as shown in figure. Pan is in equillibrium before collision. Spring constant is k and speed of the particle before collision is v0 . Answer the following three questions regarding this collision.
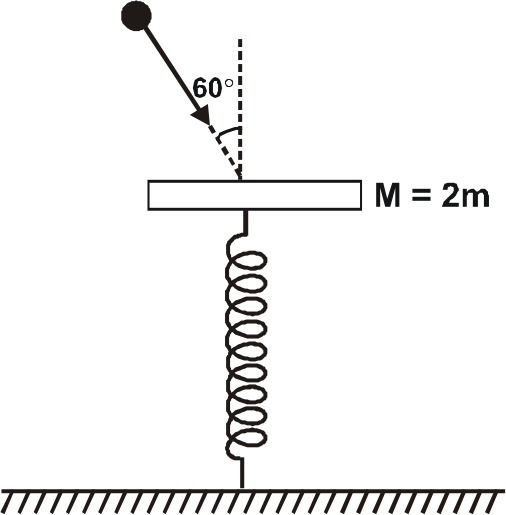
Maximum compression in the spring after the collision the
√2mkv03
√2m3kv0
√m3kv0
√mkv0

v1+v2=V0/2
2mv2−mv1=mv02⇒v1=v06,v2=v03
Maximum compression =v2√K2m=√2mkv03
Maximum height =v212g=v2072g
minimum kinetic energy =12m(√3v02)2=3mv208
Topper's Solved these Questions
Similar Questions
A particle of mass m and speed u collides elastically with the end of a uniform rod of mass M and length L as shown. If the particle comes to rest after collision, find mM
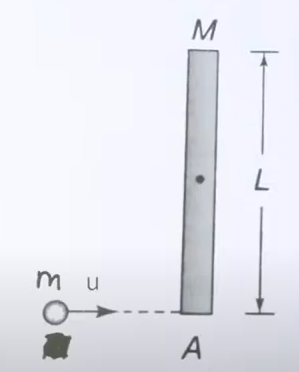 View Solution
View SolutionA particle of mass m strikes a disc of radius R and mass m with a speed u as shown in the figure. What is the speed of the particle just after the collision if the collision is perfectly inelastic ?
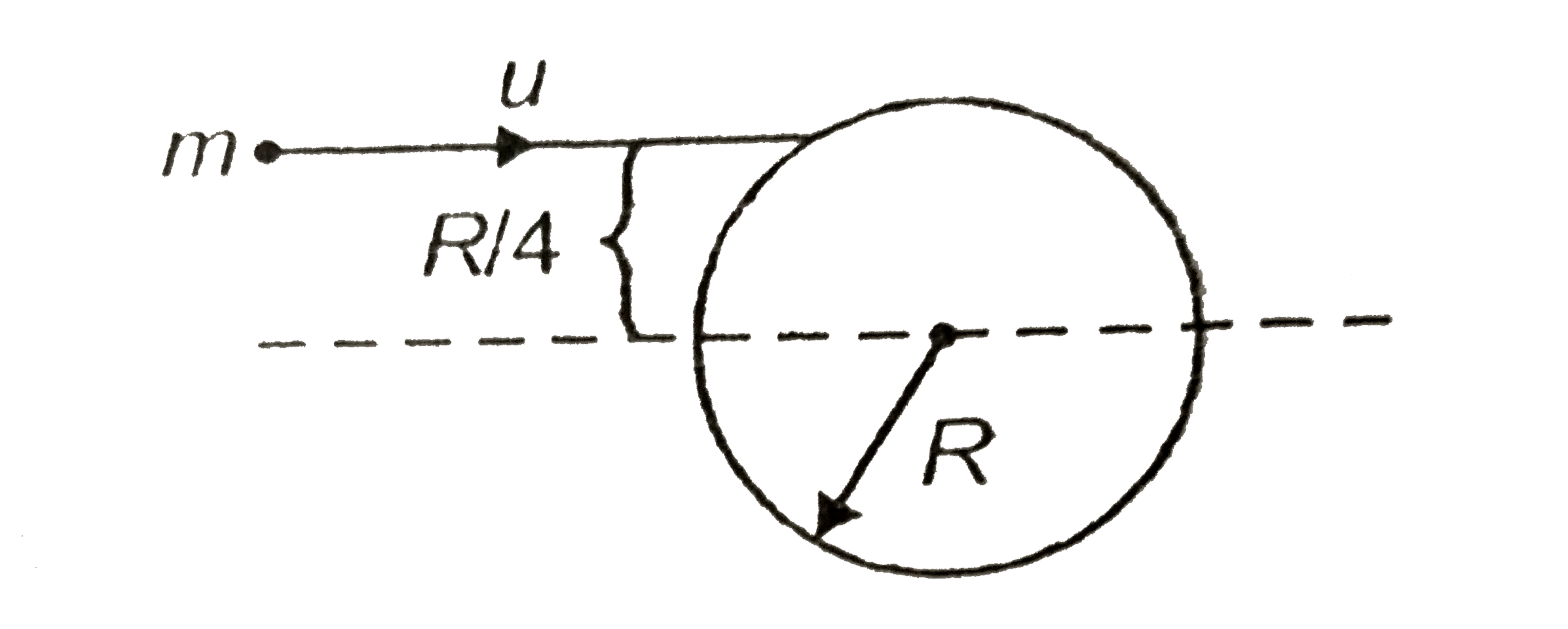 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A particle of mass m collides elastically with the pan of mass (M = 2m) of a spring balance, as shown in figure. Pan is in equillibrium before collision. Spring constant is k and speed of the particle before collision is v0 . Answer the following three questions regarding this collision.
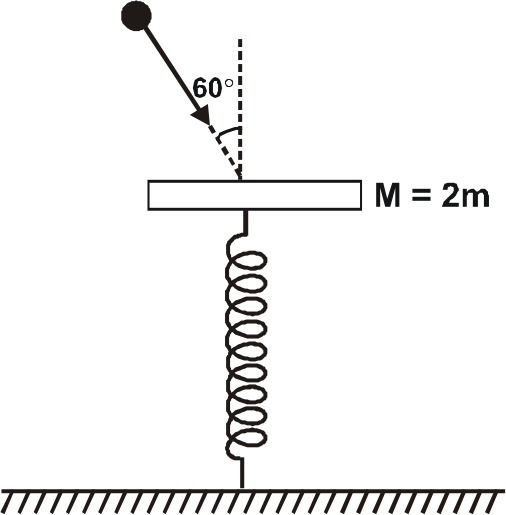
Minimum kinetic energy of the particle after collision isAmv208B3mv208C3mv204Dmv202 - Question 2 - Select One
A particle of mass m collides elastically with the pan of mass (M = 2m) of a spring balance, as shown in figure. Pan is in equillibrium before collision. Spring constant is k and speed of the particle before collision is v0 . Answer the following three questions regarding this collision.
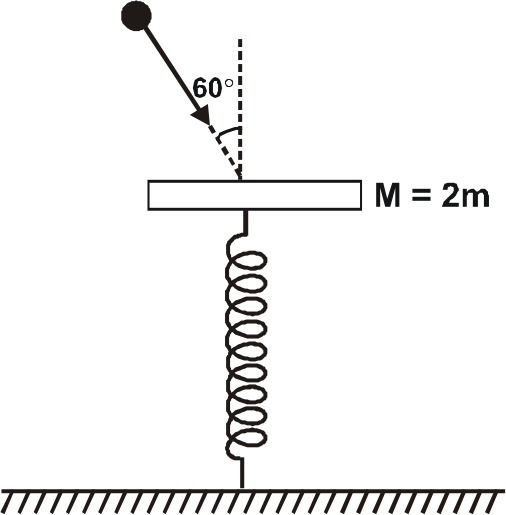
Maximum height attained by the particle from the point of collision after collision isAv2016gBv208gCv2036gDv2072g - Question 3 - Select One
A particle of mass m moving with speed V collides eleastically with another particle of mass 2mFind speed of smaller mass after head on collision
Av3B−v3C2v3D−2v3
A particle of mass m1 collides elastically with a stationary particle of mass m2(m1>m2). Find the maximum angle through which the striking particle may deviate as a result of the collision.
View SolutionA particle of mass m moving with horizontal speed 6m/s as shown in the figure. If m<<M then for one - dimensional elastic collision , the speed of lighter particle after collision will be
 View Solution
View SolutionA particle of mass m moving with horizontal speed 6m/s as shown in the figure. If m<<M then for one - dimensional elastic collision , the speed of lighter particle after collision will be
 View Solution
View SolutionA particle of mass m is attached to three identical massless springs of spring constant k as shown in the m 135 figure. The time period of vertical oscillation of the particle is
 View Solution
View SolutionA particle of mass m, collides with another stationary particle of mass M. If the particle m stops just after collision, then the coefficient of restitution for collision is equal to
View Solution

