There are two ideal springs of force constants K1 and K2 respectively. When both springs are relaxed the separation between free ends is L. Now the particle of mass m attached to free end of left spring is displaced by distance 2L towards left and then released. assuming the surface to be frictionless. (K1K2=43). (Neglect size of the block)
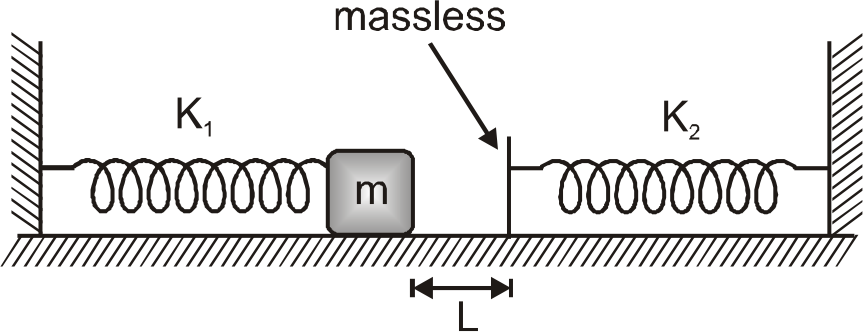
Suppose mass m hits the right spring and sticks to it. The extension in left spring when mass ‘m’ is in equilibrium position during its motion is :
4L/7
3L/7
L/3
L/2
Time taken by particle to go from
x=0 to x=A/2 is T/12
∴ time interval =T2+T12=7T12
=712.2π√mK1=7π6√mK1
Assume, maximum compression in right spring is x. hence
12K1(2L)2=12K1(L+x)2+12K2x2
Put K2=34K1, we get x=6L7
When mass m in equilibrium both spring will be in extended state.
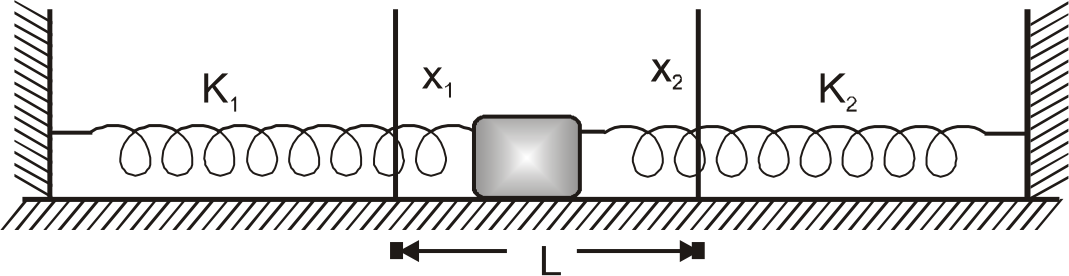
K1x1=K2x2 and x1+x2=L
x1=3L7.
Topper's Solved these Questions
Similar Questions
A radioactive element decays by β−emission. A detector records n beta particles in 2s and in next 2s it records 0.75n beta particles. Find mean life correct to nearest whole number. Given ln |2|=0.6931, ln |3|=1.0986.
05:37View SolutionWhen p−n junction diode is forward biased then
05:37View SolutionThere are two ideal springs of force constants K_1 and K_2 respectively. When both springs are relaxed the separation between free ends is L. Now the particle of mass m attached to free end of left spring is displaced by distance 2L towards left and then released. assuming the surface to be frictionless. ((K_(1))/(K_(2))=4/3) . (Neglect size of the block) The time interval after which mass 'm' hits the right spring will be
03:45View SolutionThere are two ideal springs of force constants K_1 and K_2 respectively. When both springs are relaxed the separation between free ends is L. Now the particle of mass m attached to free end of left spring is displaced by distance 2L towards left and then released. assuming the surface to be frictionless. ((K_(1))/(K_(2))=4/3) . (Neglect size of the block) The maximum compression produced in right spring will be
02:21View Solutionspring of force constant k clamped rigidly at onoe end and a mass m attached to its free end. A force F applied at the free end stretches the spring. Figure shows the same spring with both ends free and attached to a mass m at either end. Each end of the spring in figure is stretched by the same force F.
(a) What is the maximum extension of the spring in the two cases ?
(b) If the mass in figure and the two masses in figure are released free, what is the period of oscillation in each case?
 03:43View Solution
03:43View SolutionA block is placed on a frictionless horizontal table. The mass of the block is m and springs are attached on either side with force constants K_(1) and K_(2) . If the block is displaced a little and left to oscillate, then the angular frequency of oscillation will be
03:34View Solutionचित्र 15.53 में एक द्रव्यमान M को दो स्प्रिंगों A तथा B से लटकाया गया है | स्प्रिंगों के बल नियतांक क्रमश : K1 तथा K2 हैं | दोनों स्प्रिंगों के खिंचावों का योग होगा :
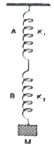 03:23View Solution
03:23View Solutionएक ऊर्ध्व स्प्रिंग मेज से खड़ा जुड़ा हुआ है । इसका बल नियतांक k है । m द्रव्यमान के एक गोले को स्प्रिंग के मुक्त सिरे के ठीक ऊपर से h ऊँचाई से गिराने पर स्प्रिंग d दूरी से पिचक जाता है इस प्रक्रम में हुआ शुद्ध कार्य होगा -
02:49View SolutionFigure a) shows a spring of force constant k clamped rigidly at once end and a mass m attached to its free end. A force F applied at the free end stretches the spring. Figure b) shows the same spring with both ends free and attached to a mass m at either end. Each end of the spring in figure is stretched by the same force F.
(a) What is the maximum extension of the spring in the two cases ?
(b) If the mass in figure and the two masses in figure are released free, what is the period of oscillation in each case?
 View Solution
View SolutionFigure 14.26 (a) shows a spring of force constant k clamped rigidly at one end and a mass m attached to its free end. A force F applied at the free end stretches the spring. Figure 14.26 (b) shows the same spring with both ends free and attached to a mass m at either end. Each end of the spring in Fig. 14.26(b) is stretched by the same force F.

What is the maximum extension of the spring in the two cases ?04:05View SolutionTwo springs are joined and connected to mass m as shown. If the springs separately have force constants K1 and K2 , then frequency of oscillation of mass m is
 03:08View Solution
03:08View Solution6kg तथा 3kg द्रव्यमान के दो ब्लॉक 2π2N/m स्प्रिंग नियतांक की द्रव्यमानहीन स्प्रिंग के दोनों सिरो से जड़े हुए हैं। यदि स्प्रिंग संपीडीत की जाती है तथा घर्षणरहित क्षैतिज सतह पर छोड़ी जाती है तो प्रत्येक ब्लॉक का आवर्त काल (सैकण्ड में) ज्ञात करो।
04:35View SolutionTwo light spring of force constants k1andk2 and a block of mass m are in the line AB on a smooth horizontal table such that one end of each spring is fixed on right supports and the other end is free as shown in figure
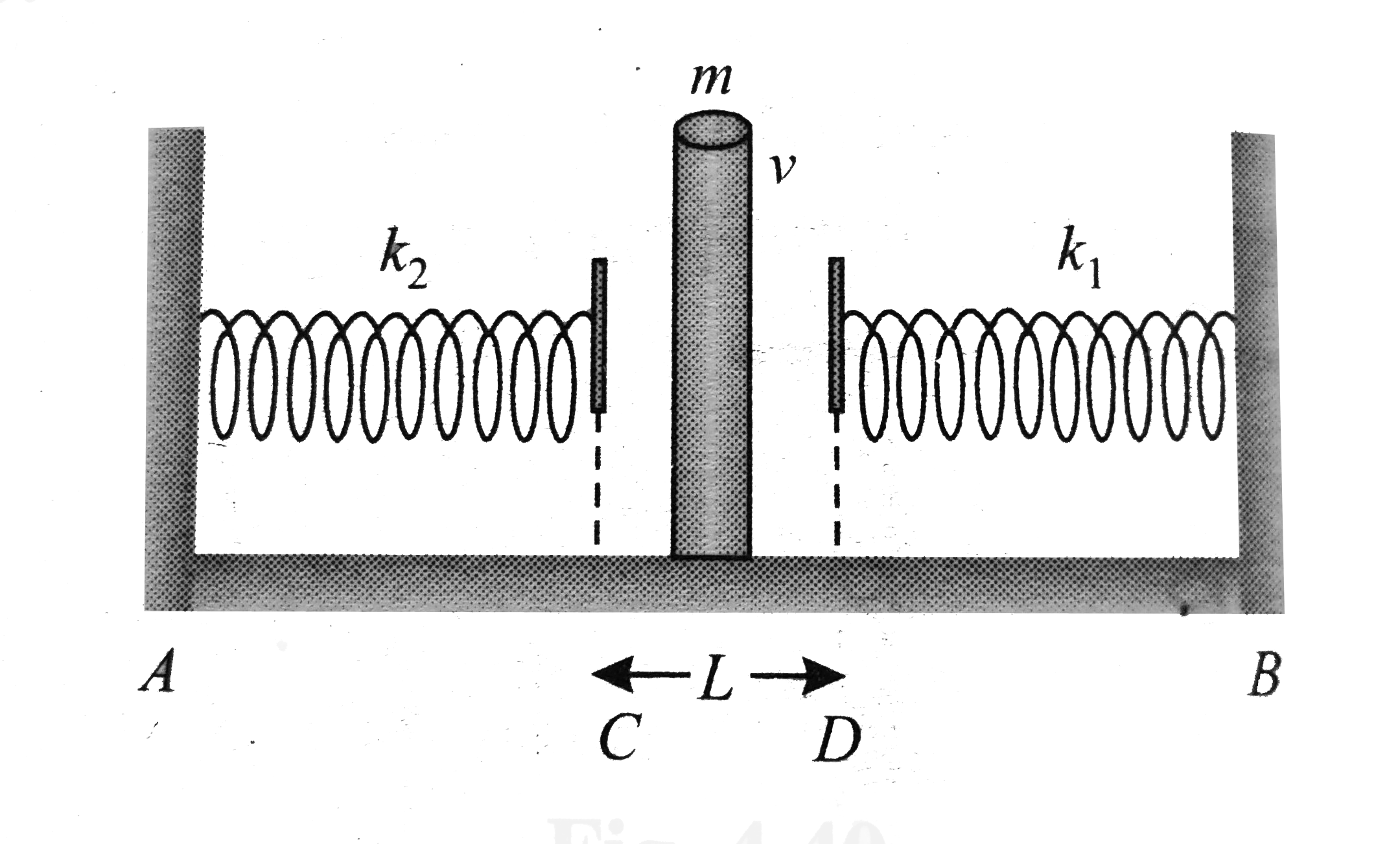
The distance CD between the free ends of the spring is 60cm. If the block (k1=1.8N/m,k2=3.2N/mandm=200g). Is the motion simple harmonic?04:56View SolutionThere are two ideal springs of force constants K_1 and K_2 respectively. When both springs are relaxed the separation between free ends is L. Now the particle of mass m attached to free end of left spring is displaced by distance 2L towards left and then released. assuming the surface to be frictionless. ((K_(1))/(K_(2))=4/3) . (Neglect size of the block) The time interval after which mass 'm' hits the right spring will be
04:39View SolutionThere are two ideal springs of force constants K_1 and K_2 respectively. When both springs are relaxed the separation between free ends is L. Now the particle of mass m attached to free end of left spring is displaced by distance 2L towards left and then released. assuming the surface to be frictionless. ((K_(1))/(K_(2))=4/3) . (Neglect size of the block) The maximum compression produced in right spring will be
04:00View Solution

