A rod is formed by joining two cylinders each having a length l and cross sectional area S. The densities of cylinder are ρ and 2ρ respectively. The rod is now horizontally suspended in a liquid of density 4ρ with help of two string as shown in the figure. The entire setup is kept inside a lift. For the quantities given in List I select the correct value from those mentioned in List II.
List-IList-II(P)Tension in string 1 if the lift is moving upwards with constant velocity(1)118ρSlg(Q)Tension in string 2 if the lift is moving upwards with constant velocity(2)98ρSlg(R)Tension is string 1 if lift is moving downwards with an acceleration of g/2(3)114ρSlg(S)Tension in string 2 if the lift is moving downwards with an acceleration of g/2(4)94ρSlg
Choose the correct option :

P-3,Q-4,R-1,S-2
P-1,Q-2,R-3,S-4
P-4,Q-3,R-2,S-1
P-2,Q-1,R-4,S-3
Consider the FBD shown in the figure
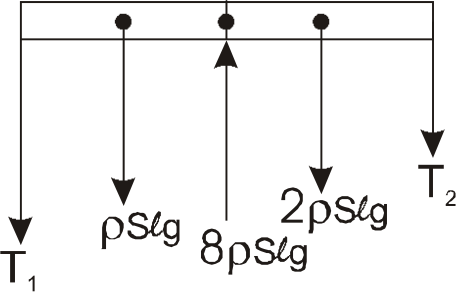
force balance
⇒T1+T2=5ρSlg
Torque balance about point P
⇒T1×2l+ρSlg×3−8ρSlg×l/2=0
T1=11/4ρSlg
⇒T2=9/4ρSlg
geff=g−g/2=g/2
Topper's Solved these Questions
Similar Questions
A radioactive element decays by β−emission. A detector records n beta particles in 2s and in next 2s it records 0.75n beta particles. Find mean life correct to nearest whole number. Given ln |2|=0.6931, ln |3|=1.0986.
06:02View SolutionWhen p−n junction diode is forward biased then
05:24View SolutionThe velocity of the liquid coming out of a small hole of a vessel containing two different liquids of densities 2ρ and ρ as shown in the figure is
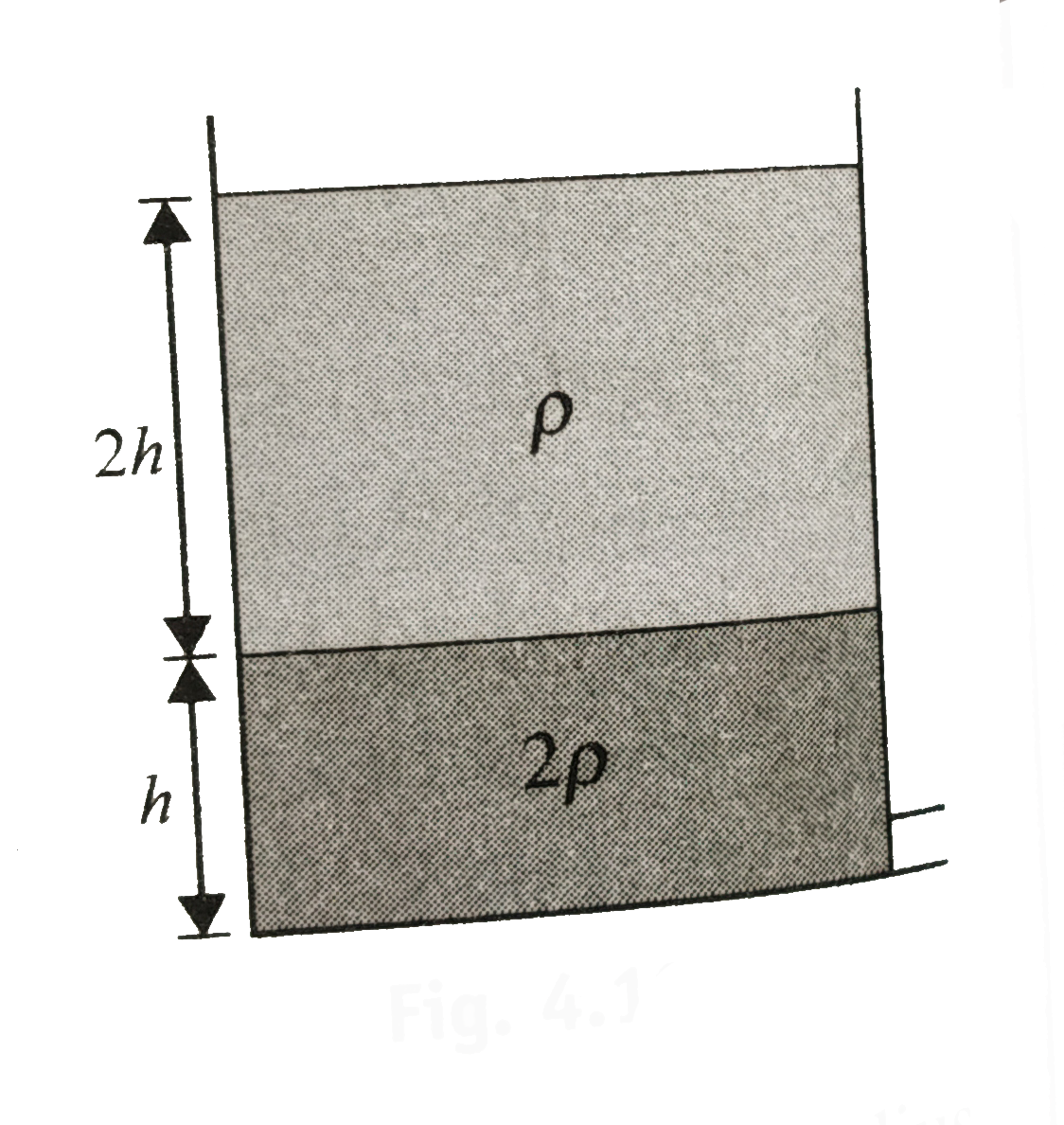 01:59View Solution
01:59View SolutionIn the arrangement as shown, A and B arc two cylinders each of length L and density d_1 and d_(2)(d_(1)ltd_(2)) respectively. They are joined together and submerged in the liquid of density d with length L//2 of A outside the liquid. If the difference in densities of two cylinders is equal to density of the liquid, find d_(1) .
02:08View SolutionA cylinder stands vertical in two immiscible liquids of densities rho and 2rho as shown. Find the difference in pressure at point A and B:
08:43View SolutionA cylinder of mass m and density ρ hanging from a string is lowered into a vessel of cross-section area s containing a liquid of density σ(<ρ) unit it is fully immersed. The increase in pressure at the bottom of the vessel is
View SolutionA liquid of density rho_0 is filled in a wide tank to a height h. A solid rod of length L, cross-section A and density rho is suspended freely in the tank. The lower end of the rod touches the base of the tank and h=L/n (where n gt 1). Then the angle of inclination theta of the rod with the horizontal in equilibrium position is
View SolutionA rod of length L, cross sectional area A and density ρ is hanging from a rigid support by spring of stiffries k. A very small sphere of mass m is rigidly attached at the bottom of the rod. The rod is partially immersed in a liquid of density ρ. Find the period of small oscillations.
View SolutionTwo non-mixing liquids of densities ρ and n ρ(n>1) are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats with its axis vertical and length PL (P<1) in the denser liquid. The density dis equal to
07:12View SolutionTwo non-mixing liquids of densities ρ and n ρ(n>1) are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats with its axis vertical and length PL (P<1) in the denser liquid. The density dis equal to
03:51View SolutionA liquid of density rho_0 is filled in a wide tank to a height h. A solid rod of length L, cross-section A and density rho is suspended freely in the tank. The lower end of the rod touches the base of the tank and h=L/n (where n gt 1). Then the angle of inclination theta of the rod with the horizontal in equilibrium position is
02:42View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscous and incompressible liquids of densities d and 2d each of height H//2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P_(0) . A homogeneous solid cylinder of length L(LltH//2) and cross-sectional area A//5 is immersed such that it floats with its axis vertical at the liquid-liquid interface with length L//4 in the denser liquid, The density D of the material of the floating cylinder is
04:01View SolutionThe velocity of the liquid coming out of a small hole of a vessel containing two different liquids of densities 2rho and rho as shown in the figure is
02:56View SolutionThe velocity of the liquid coming out of a small hole of a vessel containing two different liquids of densities 2ρ and ρ as shown in the figure is
 View Solution
View SolutionIn the arrangement as shown, A and B arc two cylinders each of length L and density d1 and d2(d1<d2) respectively. They are joined together and submerged in the liquid of density d with length L/2 of A outside the liquid. If the difference in densities of two cylinders is equal to density of the liquid, find d1.
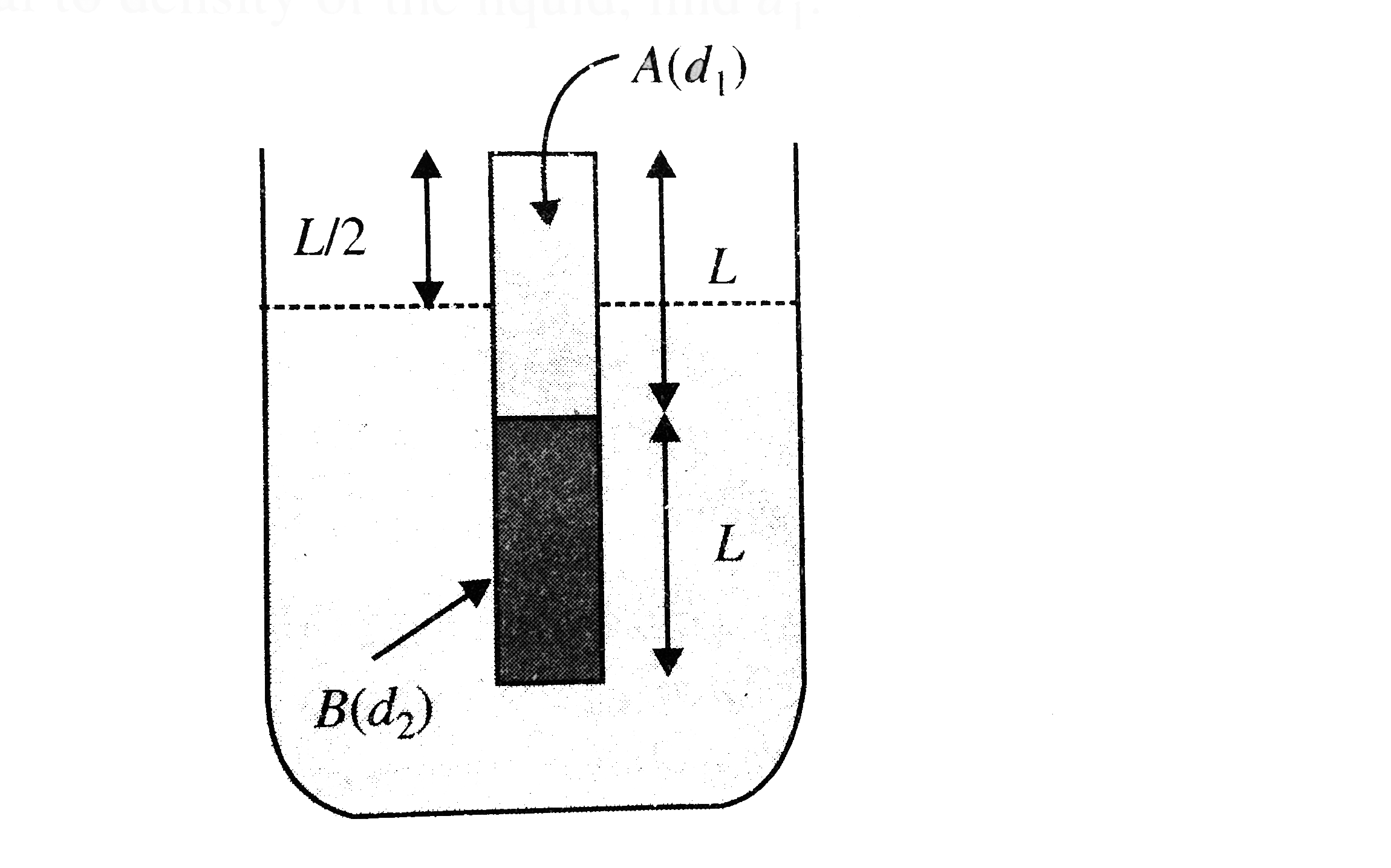 04:05View Solution
04:05View Solution

