A body is weighed with a spring balance in a train at rest, shown a weight W. When the train begins to move with a velocity υ around the equator from west to east and if the angular velocity of the train is ω then the weight shown by spring balance is
W
W(1−2υωg)
W(1−2υωg)
W(1−υ2/R)
The correct Answer is:C
Observed weight of body on equator due to rotation of earth =mg−mrω2.
Observed weight of body due to motion of train from west to east with velocity υ (as centrifugal forces will be acting) will be
W'=(mg−mrω2)−mυ2/r
=mg−mυω−mυω=mg−2mυω
=mg(1−2υω/g)
=W(1−2υω/g)
Similar Questions
Two 2 kg weight are attached to spring balance as shown in figure-2.122. What is the reading of the scale ?
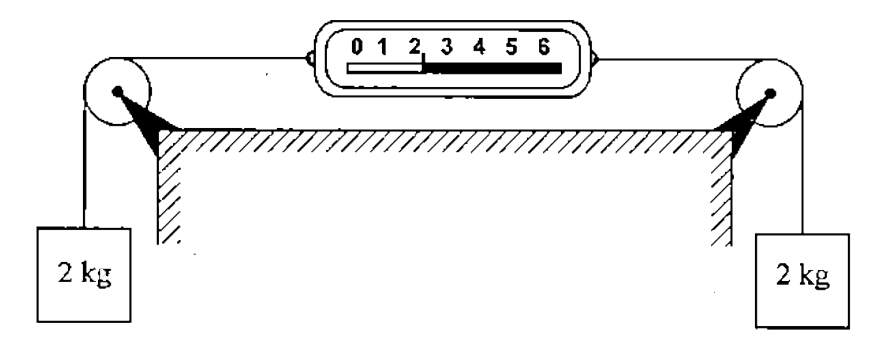 View Solution
View SolutionParticles AandB move with constant and equal speeds in a circle as shown in (Fig. 5.164). Find the angular velocity of the particle A with respect to B, if the angular velocity of particle Aw.r.t.O is ω.
 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
A train of mass m moves with a velocity υ on the equator from east to west. If ω is the angular speed of earth about its axis and R is the radius of the earth then the normal reaction acting on the train is
Amg[1−(ωR−2υ)ωg−υ2Rg]Bmg[1−2(ωR−υ)ωg−υ2Rg]Cmg[1−(ωR−2υ)ωg−υ2Rg]Dmg[1−2(ωR−υ)ωg−υ2Rg] - Question 2 - Select One
Two identical trains A and B move with equal speeds on parallel tracks along the equator. A moves from east to west and B moves from west to east. Which train will exert greater force on the track?
AABBCthey will exert equal forceDthe mass and the speed of each train must be known to reach a conclusion. - Question 3 - Select One
A bird is flying towards south with a velocity 40km/hr and a train is moving with a velocity 40km/hr towards east. What is the velocity of the bird w.r.t. an obserber in train:-
A40√2 km/hr. North-EastB40√2 km/hr. South-EastC40√2 km/hr. South -WestD40√2 km/hr. North-West
A body is suspended on a spring balance in a ship sailing along the equator with a speed v. Show that the scale reading will be very close to W0(1±2ωvg), where ω is the angular speed of the earth and W0 is the reading of spring balance when the ship is at rest. Explain the plus or minus sign
[Hint : W0=mg−mω2R,W=mg−m(mω+v)2R when the ship is moving from west to east]View SolutionA particle is moving with constant speed in a circle as shown in (Fig. 5.163). Find the angular velocity of the particle A with respect to fixed point BandC if angular velocity with respect to Oisω.
 .View Solution
.View SolutionA body suspended on a spring balance in a ship weighs W0 when the ship is at rest. When the ship begins to move along the equator with a speed v, show that the scale reading is very close to W0(1±2ωV/g), where ω is the angular speed of the earth.
View SolutionA bird is flying towards south with a velocity 40km/h and a train is moving with a velocity 40 km/h towards east. What is the velocity of the bird w.r.t. an obserber in the train ?
View SolutionAt time t = 0, two bodies A and B at the same point. A moves with constant velocity υ and B starts from rest and moves with constant acceleration. Relative velocity of B w.r.t. A when the bodies meet each other is
View Solution

