A hydraulic press with the larger piston of diameter 35 cm at a heigth of 1.5 m relative to the smaller piston of diameter 10 cm. The mass on the smaller piston is 20 kg. What is the force exerted on the load placed. On the larger piston ? The density of oil in the press is 750kgm−3
The correct Answer is:1.34×103N
To solve the problem step by step, we will use the principles of hydraulics and the relationship between pressure, force, and area.
Step 1: Calculate the Area of the Smaller Piston
The area A1 of the smaller piston can be calculated using the formula for the area of a circle:
A1=π(D12)2
Where D1=10cm=0.1m.
Calculating:
A1=π(0.12)2=π(0.05)2=π×0.0025≈0.00785m2
Step 2: Calculate the Force on the Smaller Piston
The force F1 exerted by the mass on the smaller piston is given by:
F1=m⋅g
Where m=20kg and g≈10m/s2.
Calculating:
F1=20⋅10=200N
Step 3: Calculate the Area of the Larger Piston
The area A2 of the larger piston can be calculated similarly:
A2=π(D22)2
Where D2=35cm=0.35m.
Calculating:
A2=π(0.352)2=π(0.175)2=π×0.030625≈0.0962m2
Step 4: Calculate the Pressure at the Smaller Piston
The pressure P at the smaller piston is given by:
P=F1A1
Calculating:
P=2000.00785≈25464.5Pa
Step 5: Calculate the Pressure at the Larger Piston
The pressure at the larger piston must account for the height difference due to the oil. The pressure at the larger piston P2 is given by:
P2=P+ρgh
Where ρ=750kg/m3, g=10m/s2, and h=1.5m.
Calculating:
P2=25464.5+(750⋅10⋅1.5)=25464.5+11250=36714.5Pa
Step 6: Calculate the Force on the Larger Piston
Now we can find the force F2 exerted on the larger piston using the pressure:
F2=P2⋅A2
Calculating:
F2=36714.5⋅0.0962≈3525.2N
Final Answer
The force exerted on the load placed on the larger piston is approximately 3525.2N.
---
The area A1 of the smaller piston can be calculated using the formula for the area of a circle:
A1=π(D12)2
Where D1=10cm=0.1m.
A1=π(0.12)2=π(0.05)2=π×0.0025≈0.00785m2
The force F1 exerted by the mass on the smaller piston is given by:
F1=m⋅g
Where m=20kg and g≈10m/s2.
F1=20⋅10=200N
The area A2 of the larger piston can be calculated similarly:
A2=π(D22)2
Where D2=35cm=0.35m.
A2=π(0.352)2=π(0.175)2=π×0.030625≈0.0962m2
The pressure P at the smaller piston is given by:
P=F1A1
P=2000.00785≈25464.5Pa
The pressure at the larger piston must account for the height difference due to the oil. The pressure at the larger piston P2 is given by:
P2=P+ρgh
Where ρ=750kg/m3, g=10m/s2, and h=1.5m.
P2=25464.5+(750⋅10⋅1.5)=25464.5+11250=36714.5Pa
Now we can find the force F2 exerted on the larger piston using the pressure:
F2=P2⋅A2
F2=36714.5⋅0.0962≈3525.2N
The force exerted on the load placed on the larger piston is approximately 3525.2N.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions-II14videos
PROPERTIES OF BULK MATTER
- BOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions-I173videos
PROPERTIES OF BULK MATTER
- BOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Fill in the Blanks E10videos
PROPERTIES OF BULK MATTER
- BOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance18videos
PHYSICAL WORLD AND MEASUREMENT
- BOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)25videos
RAY OPTICS
Similar Questions
Shows a hydraulic press with the larger piston of diameter 35cm at a height of 1.5m relative to the smaller piston of diameter 10cm. The mass on the smaller piston is 20gk. What is the force exerted on the load by the lerger piston ? The density of oil in the press is 750kgm−3.
View SolutionShows a hydraulic press with the larger piston of diameter 35cm at a height of 1.5m relative to the smaller piston of diameter 10cm. The mass on the smaller piston is 20gk. What is the force exerted on the load by the lerger piston ? The density of oil in the press is 750kgm−3.
View Solution
Knowledge Check
- Question 1 - Select One
The hydraulic press shown in the figure is used to raise the mass M through a height of 0.5cm by performing 500J of work at the small piston. The diameter of the large piston is 10cm, while that of the smaller one is 2cm. The mass M is
 A100kgB106kgC103kgDNone of these
A100kgB106kgC103kgDNone of these - Question 2 - Select One

The hydraulic press shown in the figure is used to raise the mass M through a height of 5.0 mm by performing 500 J of work at the small piston. The diameter of the large piston is 10 cm while that of the smaller one is 2 cm. The mass M isA104kgB103kgC100kgD105kg
Figure shown a hydraulic press with the larger piston if diameter 35 cm at a height of 1.5 cm at a height of 1.5 m relative to the smaller piston of diameter 10cm. The mass on the smaller piston is 20 kg. What is the force exerted on the load by the larger piston? The density of oil in the press is 750kh/m3 . (Take g=9.8m/s2)
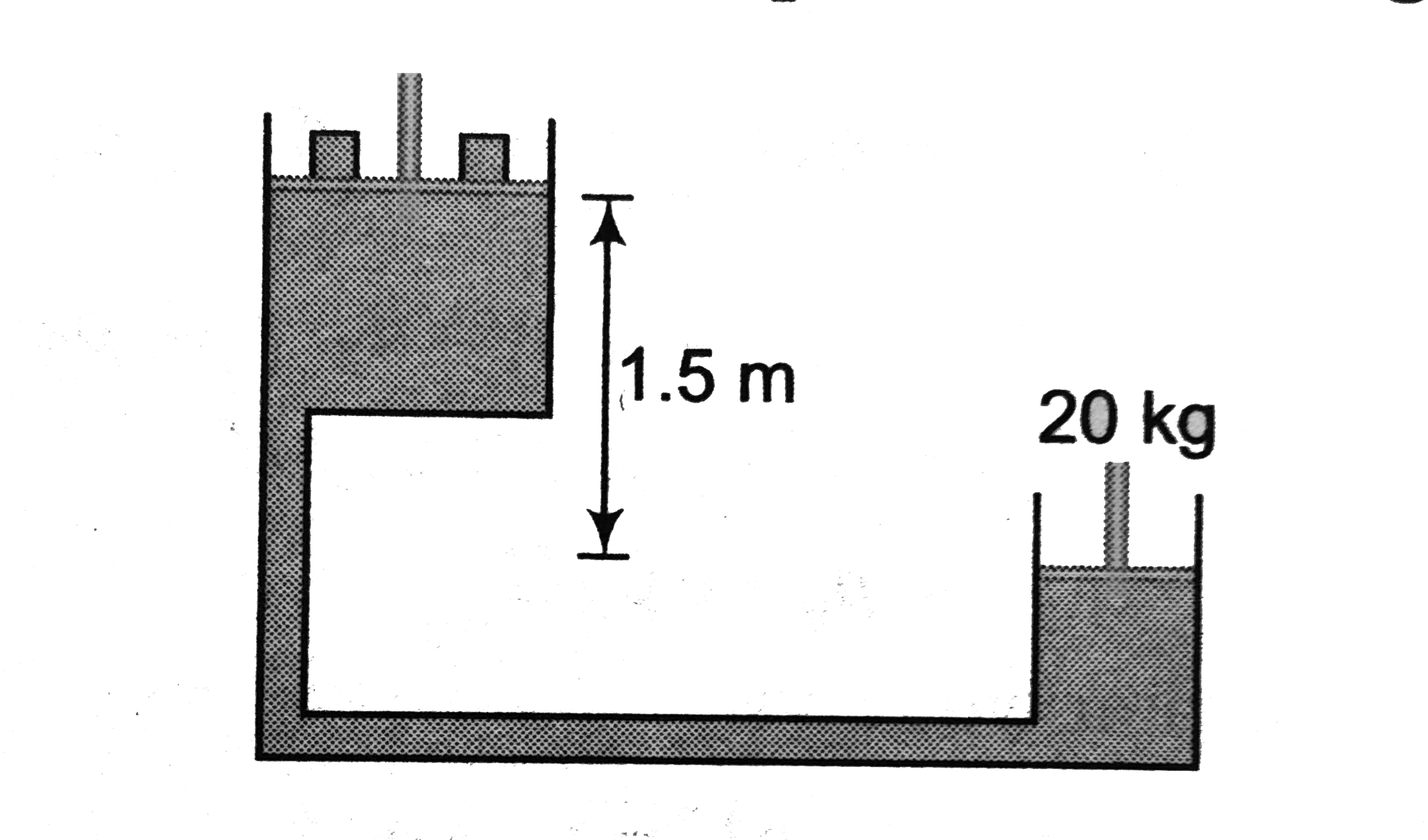 .View Solution
.View SolutionA car is lifted by a hydraulic jack that consists of two pistons. The diameter of the larger piston is 2 m and that of the smaller piston is 50 cm. If the force applied on the smaller piston is 240 N, find the weight of the car.
View SolutionA car is lifted by a hydraulic jack that consists of two pistons. The diameter of the larger piston is 2 m and that of the smaller piston is 50 cm. If the force applied on the smaller piston is 240 N, find the weight of the car.
View SolutionThe area of the smaller piston of a hydraulic press is 1cm2 and that of larger piston is 22cm2. How much weight can be raised on the larger piston by a 200kg f exerted on the smaller piston?
View SolutionIn a hydraulic press, the area of smaller piston is 5 cm2. The weight raised by larger piston is 900 kgf if a force of 150 kgf is applied on smaller piston. Calculate the area of larger piston.
View SolutionThe area of the smaller piston of a hydraulic press is 1cm2 and that of larger piston of a hydralic press is 1cm2.How much weight can be raised on the larger piston by a 200 kg f exerted on the smaller piston?
View Solution

