A block rests on a horizontal table which is executing SHM in the horizontal plane with an amplitude A. What will be the frequency of oscillation, the block will just start to slip? Coefficient of friction=μ.
To solve the problem, we need to find the frequency of oscillation at which the block will just start to slip on a table executing simple harmonic motion (SHM). Here’s a step-by-step solution:
Step 1: Understand the forces acting on the block
When the table executes SHM, the block experiences a restoring force due to the motion of the table. The block will start to slip when the maximum restoring force exceeds the maximum static frictional force acting on it.
Step 2: Identify the restoring force
The restoring force Frestoring acting on the block when the table is at its maximum displacement (amplitude A) can be expressed as:
Frestoring=mω2A
where m is the mass of the block and ω is the angular frequency of the SHM.
Step 3: Identify the frictional force
The maximum static frictional force Ffriction that can act on the block is given by:
Ffriction=μmg
where μ is the coefficient of friction and g is the acceleration due to gravity.
Step 4: Set the forces equal
At the point of slipping, the maximum restoring force equals the maximum static frictional force:
mω2A=μmg
Step 5: Simplify the equation
We can cancel m from both sides (assuming m≠0):
ω2A=μg
Step 6: Solve for ω
Rearranging the equation gives us:
ω2=μgA
Taking the square root of both sides, we find:
ω=√μgA
Step 7: Relate angular frequency to frequency
The angular frequency ω is related to the frequency f by the formula:
ω=2πf
Substituting for ω:
2πf=√μgA
Step 8: Solve for frequency f
Now we can solve for f:
f=12π√μgA
Final Answer
Thus, the frequency of oscillation at which the block will just start to slip is:
f=12π√μgA
When the table executes SHM, the block experiences a restoring force due to the motion of the table. The block will start to slip when the maximum restoring force exceeds the maximum static frictional force acting on it.
The restoring force Frestoring acting on the block when the table is at its maximum displacement (amplitude A) can be expressed as:
Frestoring=mω2A
where m is the mass of the block and ω is the angular frequency of the SHM.
The maximum static frictional force Ffriction that can act on the block is given by:
Ffriction=μmg
where μ is the coefficient of friction and g is the acceleration due to gravity.
At the point of slipping, the maximum restoring force equals the maximum static frictional force:
mω2A=μmg
We can cancel m from both sides (assuming m≠0):
ω2A=μg
Rearranging the equation gives us:
ω2=μgA
Taking the square root of both sides, we find:
ω=√μgA
The angular frequency ω is related to the frequency f by the formula:
ω=2πf
Substituting for ω:
2πf=√μgA
Now we can solve for f:
f=12π√μgA
Thus, the frequency of oscillation at which the block will just start to slip is:
f=12π√μgA
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Long Answer Questions33videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Advanced Problems For Competitions20videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Very Short Answer Questions99videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks5videos
MATHEMATICAL TOOLS
- BOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance18videos
PHYSICAL WORLD AND MEASUREMENT
Similar Questions
An object kept on the platform a horizontal platform executing SHM, up and down with amplitude 80 cm. At what frequency will the object just lose contact with the platform?
View SolutionA block is kept on a horizontal table that is executing simple harmonic motion of frequency v in the horizontal plane. The coefficient of static friction between the block and the table is μ. Determine the maximum amplitude of the table for which the block does not slip on the surface of the table.
View Solution
Knowledge Check
- Question 1 - Select One
A block resting on the horizontal surface executes S.H.M. in horizontal plane with amplitude 'A'. The frequency of oscillation for which the block just starts to slip is (μ = coefficient of friction, g = gravitational acceleration)
A12π√μgAB14π√μgAC2π√AμgD4π√Aμg - Question 2 - Select One
In the given system, a block of mass m is resting on a board which makes an angle θ with the horizontal. What should be the minimum value of θ, so that the block just starts moving downwards? The coefficient of friction between the surfaces of the board and the block is μ.
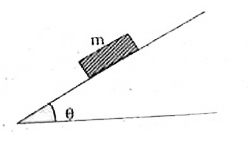 Atan−1(1/μ)Btan−1(μ)Csin−1μDnone
Atan−1(1/μ)Btan−1(μ)Csin−1μDnone - Question 3 - Select One
A block on a horizontal slab is moving horizontally with a simple harmonic motion of frequency two oscillations per second. If the coefficient of static friction between block and slab is 0.5, then the amplitude of the oscillation will be (if the block does not slip along the slab)
A3.3 cmB3.5 cmC3.15 cmD7 cm
A block lying on a horizontal table executes SHM of period 1 second, horizontally. What is the maximum amplitude for which the block does not slide if coeff. Of friction between block and surface is 0.4,π2=10.
View SolutionA block is on horizontal slab which is moving horizontally and excuting S.H.M. The coefficient of static frication between block and slab is μ. If block is not separated from slab then determine range of angular frequency of oscillation.
View SolutionA block is on a horizontal surface (a shake table) that is moving back and forth horizontally with simple harmonic motion of frequency 2.0 Hz. The coefficient of static friction between block and surface is 0.45. How great can the amplitude of the SHM be if the block is not to slip along the surface?
View SolutionA block of mass M rests on a rough horizontal table. A steadily increasing horizontal force is applied such that the block starts to slide on the table without toppling. The force is contained even after sliding has started. Assume the coefficients of static end kinetic friction between the table and block to be equal. The correct representation of the variation of the frictional force , f1 exerted by the table on the block with time t is given by -
View SolutionA block of mass 4kg rests on a rough horizontal plane. The plane is gradually inclined until at an angle θ=60∘ , with the vertical, the mass just begins to side. What is the coefficient of static friction between the block and the plane?
View Solution

