An object kept on the platform a horizontal platform executing SHM, up and down with amplitude 80 cm. At what frequency will the object just lose contact with the platform?
The correct Answer is:0.56
To solve the problem of determining the frequency at which an object just loses contact with a platform executing simple harmonic motion (SHM) with an amplitude of 80 cm, we can follow these steps:
1. Understanding the Problem:
- The object is on a platform that is oscillating vertically with a certain amplitude (A = 80 cm = 0.8 m).
- We need to find the frequency (f) at which the object will lose contact with the platform.
2. Condition for Losing Contact:
- The object will lose contact with the platform when the acceleration of the platform exceeds the acceleration due to gravity (g).
- The maximum acceleration (a_max) of the platform in SHM is given by the formula:
amax=Aω2
where ω is the angular frequency.
3. Relating Angular Frequency to Frequency:
- The angular frequency ω is related to the frequency f by the equation:
ω=2πf
- Substituting this into the acceleration formula gives:
amax=A(2πf)2=4π2Af2
4. Setting Up the Equation:
- For the object to just lose contact, we set the maximum acceleration equal to the acceleration due to gravity:
4π2Af2=g
5. Solving for Frequency:
- Rearranging the equation to solve for f:
f2=g4π2A
- Taking the square root gives:
f=√g4π2A
6. Substituting Values:
- Given g=10m/s2 and A=0.8m:
f=√104π2×0.8
7. Calculating the Frequency:
- First, calculate 4π2:
4π2≈39.478
- Now substitute this value into the equation:
f=√1039.478×0.8=√1031.5824≈√0.316≈0.56Hz
Final Answer:
The frequency at which the object just loses contact with the platform is approximately 0.56 Hz.
- The object is on a platform that is oscillating vertically with a certain amplitude (A = 80 cm = 0.8 m).
- We need to find the frequency (f) at which the object will lose contact with the platform.
- The object will lose contact with the platform when the acceleration of the platform exceeds the acceleration due to gravity (g).
- The maximum acceleration (a_max) of the platform in SHM is given by the formula:
amax=Aω2
where ω is the angular frequency.
- The angular frequency ω is related to the frequency f by the equation:
ω=2πf
- Substituting this into the acceleration formula gives:
amax=A(2πf)2=4π2Af2
- For the object to just lose contact, we set the maximum acceleration equal to the acceleration due to gravity:
4π2Af2=g
- Rearranging the equation to solve for f:
f2=g4π2A
- Taking the square root gives:
f=√g4π2A
- Given g=10m/s2 and A=0.8m:
f=√104π2×0.8
- First, calculate 4π2:
4π2≈39.478
- Now substitute this value into the equation:
f=√1039.478×0.8=√1031.5824≈√0.316≈0.56Hz
The frequency at which the object just loses contact with the platform is approximately 0.56 Hz.
Topper's Solved these Questions
Similar Questions
A horizontal platform vibrate up and down with a simple harmonic motion of amplitude 20 c.m. At what frequency will an object kept on the platform remain just in contact with the platform ? Take g=9.81m/s2.
View SolutionA particle performs linear SHM such that it is placed on plat- form & platform along with particles oscillate vertically up and down with amplitude A = 1cm. If the particle does not loose contact with platform anywhere and mass of particle is 1 kg, find :
The minimum, possible time period (take π=√g)
View Solution
Knowledge Check
A block resting on the horizontal surface executes S.H.M. in horizontal plane with amplitude 'A'. The frequency of oscillation for which the block just starts to slip is (μ = coefficient of friction, g = gravitational acceleration)
A12π√μgAB14π√μgAC2π√AμgD4π√AμgA horizontal platform with a small block of mass 1 kg kept on it performs vertical SHM of amplitude 1 cm. The block does not lose contact with the platform anywhere. The minimum possible time period of the platform
A0.1 sB0.2 sC0.3 sD0.4 sA man weighing 60kg stands on the horizontal platform of a spring balance. The platform starts executing simple harmonic motion of amplitude 0.1m and frequency 2πHz. Which of the following statement is correct ?
 AThe spring balance reads the weight of man as 60kgBThe spring balance reading fluctuates between 60kg and 70kgCThe spring balance reading fluctuates bettween 50kg and 60kgDThe spring balance reading fluctuates between 50kg and 70kg
AThe spring balance reads the weight of man as 60kgBThe spring balance reading fluctuates between 60kg and 70kgCThe spring balance reading fluctuates bettween 50kg and 60kgDThe spring balance reading fluctuates between 50kg and 70kg
A particle performs linear SHM such that it is placed on plat- form & platform along with particles oscillate vertically up and down with amplitude A = 1cm. If the particle does not loose contact with platform anywhere and mass of particle is 1 kg, find :
For minimum time period condition average potential energy between t = 0 to t = 0.05 sec("Take" " "g=10m//s^(2))
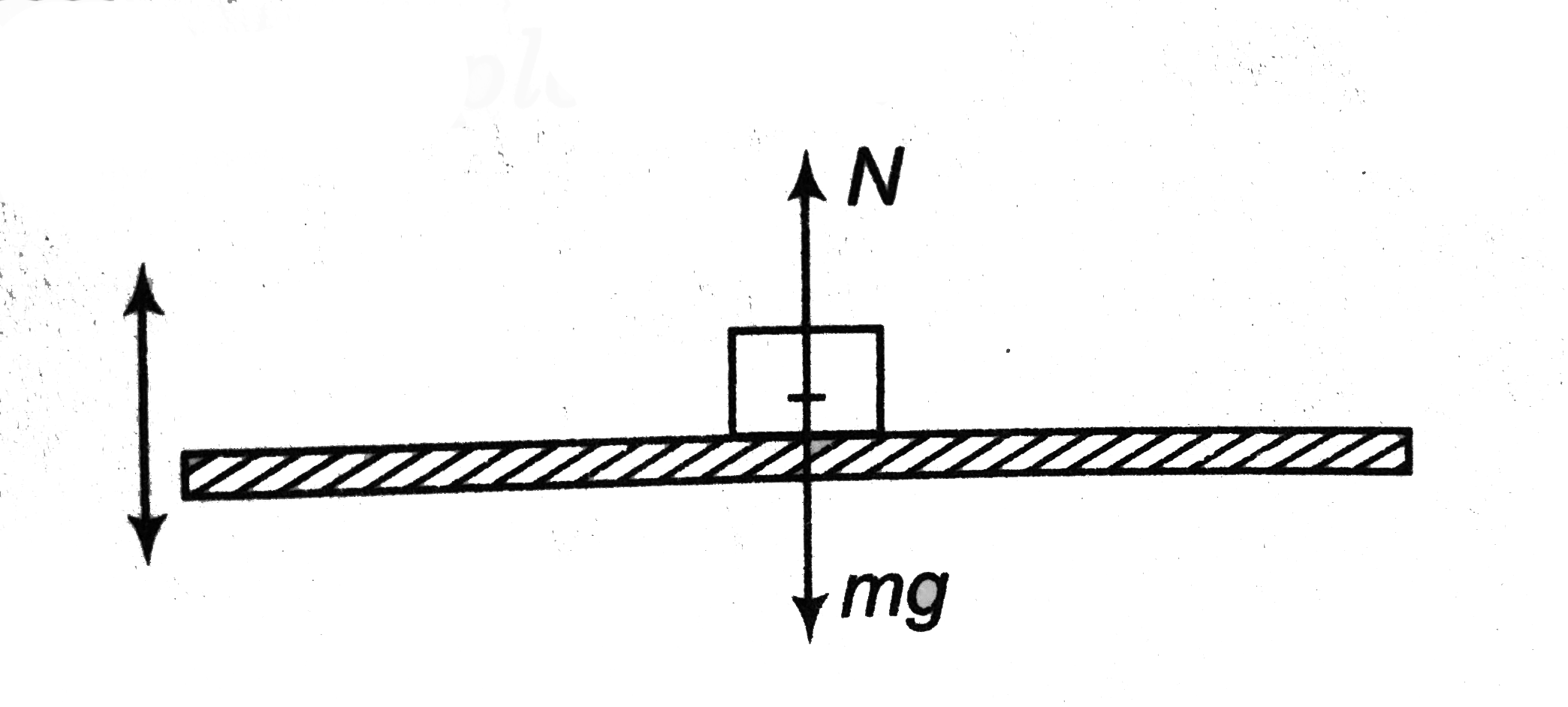
View SolutionA block is kept is kept over a horizontal platform, executing vertical SHM of angular frequency ω. Find maximum amplitude of oscillations, so that the block does not leave contact with the platform.
View SolutionA block rests on a horizontal table which is executing SHM in the horizontal plane with an amplitude A. What will be the frequency of oscillation, the block will just start to slip? Coefficient of friction=μ.
View SolutionWhat is the maximum acceleration of a platform that oscillates at amplitude 2.50cm and frequency 6.60Hz?
View SolutionA train approaching a platform at a speed of 54kmh−1 sounds a whistle. An observer on the platform finds its frequency to be 1620 Hz. The train passes the platform keeping the whistle on and without slowing down. What frequency will the observer hear after the train has crossed the platfrom ? The speed of sound in air =332ms−1
View Solution
