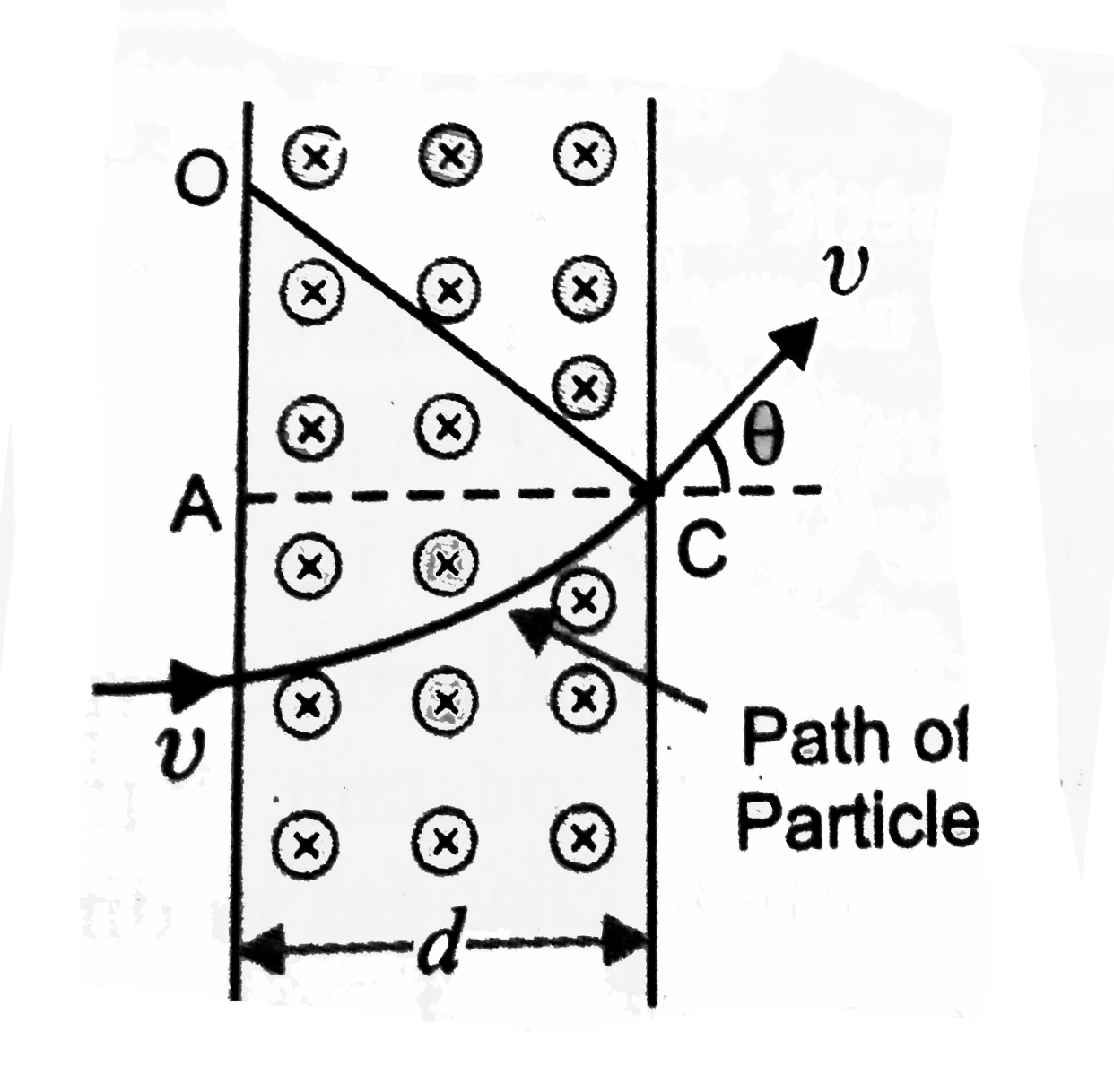
A proton and an alpha particle are projected in a perpendicular magnetic field B which exists in a region of width d, figure. Find the ratio of the sines of angles of deviation suffered by the proton and the alpha particle if before entering the magnetic field both the particles (i) have the same momentum (ii) have the same kinetic energy.

Video Solution

Text Solution

Verified by Experts

If $m_{p}$ , $m_{α}$ are the masses of proton and $α$ -particle then
$m_{α} = 4 m_{p}$ .
If $q_{p}$ , $q_{α}$ are the charges of proton and $α$ -particle.
then $q_{α} = 2 q_{p}$ .
When a charged particle describes a circular path in the magnetic field, then radius of the path is $r = \frac{m v}{B q} = \frac{p}{B q} = \frac{\sqrt{2 m K}}{B q}$
where p is momentum and K is the kinetic energy of the particle. From figure, it is clear that $θ$ is the angle of deviation (i.e., angle between direction of final velocity and initial velocity) of the charged particle on leaving the magnetic field.
In $Δ O A C$ ,
$sin θ = \frac{d}{r} = \frac{B q d}{m v} = \frac{B q d}{p} = \frac{B q d}{\sqrt{2 m K}}$
Case (i). When $p_{α} = p_{p}$ then $sin θ \propto q$
$∴ \frac{{sin θ}_{p}}{{sin θ}_{α}} = \frac{q_{p}}{q_{α}} = \frac{1}{2}$
Case (ii). When $K_{α} = K_{p}$ , then $sin θ \propto \frac{q}{\sqrt{m}}$
$\frac{{sin θ}_{p}}{{sin θ}_{q}} = \frac{q_{p}}{q_{α}} \times \sqrt{\frac{m_{α}}{m_{p}}} = \frac{1}{2} \times \sqrt{\frac{4}{1}} = 1$

Updated on:21/07/2023