A proton and an alpha particle, both are moving with same initial velocity, perpendicular to uniform magnetic field of 1 T. Find the ratio of radii of circles described by the alpha particle and the proton.
To find the ratio of the radii of the circles described by a proton and an alpha particle moving in a magnetic field, we can follow these steps:
Step 1: Understand the motion in a magnetic field
When a charged particle moves perpendicular to a magnetic field, it experiences a magnetic force that causes it to move in a circular path. The radius of this circular path can be determined using the formula derived from the balance of forces.
Step 2: Write the formula for the radius of circular motion
The radius R of the circular path of a charged particle in a magnetic field is given by the formula:
R=mvqB
where:
- m is the mass of the particle,
- v is the velocity of the particle,
- q is the charge of the particle,
- B is the magnetic field strength.
Step 3: Identify the parameters for the proton and alpha particle
1. Proton:
- Mass (mp): mp
- Charge (qp): e (where e is the elementary charge)
2. Alpha Particle:
- Mass (mα): 4mp (since an alpha particle consists of 2 protons and 2 neutrons)
- Charge (qα): 2e
Step 4: Calculate the radius for both particles
Using the formula for the radius:
1. For the proton:
Rp=mpveB
2. For the alpha particle:
Rα=4mpv2eB=2mpveB
Step 5: Find the ratio of the radii
Now, we can find the ratio of the radii of the proton and the alpha particle:
RpRα=mpveB2mpveB=12
Thus, the ratio of the radii is:
Rp:Rα=1:2
Final Answer
The ratio of the radii of the circles described by the alpha particle and the proton is 2:1.
---
When a charged particle moves perpendicular to a magnetic field, it experiences a magnetic force that causes it to move in a circular path. The radius of this circular path can be determined using the formula derived from the balance of forces.
The radius R of the circular path of a charged particle in a magnetic field is given by the formula:
- m is the mass of the particle,
- v is the velocity of the particle,
- q is the charge of the particle,
- B is the magnetic field strength.
1. Proton:
- Mass (mp): mp
- Charge (qp): e (where e is the elementary charge)
- Mass (mα): 4mp (since an alpha particle consists of 2 protons and 2 neutrons)
- Charge (qα): 2e
Using the formula for the radius:
Rp=mpveB
Rα=4mpv2eB=2mpveB
Now, we can find the ratio of the radii of the proton and the alpha particle:
The ratio of the radii of the circles described by the alpha particle and the proton is 2:1.
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - PRACTICE PROBLEMS
59 Videos
- MOVING CHARGES AND MAGNETISMBOOK - MODERN PUBLICATIONCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Conceptual Questions
29 Videos
- MAGNETISM AND MATTERBOOK - MODERN PUBLICATIONCHAPTER - MAGNETISM AND MATTEREXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
16 Videos
- NUCLEIBOOK - MODERN PUBLICATIONCHAPTER - NUCLEIEXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION
15 Videos
Similar Questions
Knowledge Check
A proton an an α−particle, moving with the same velocity, enter a uniform magnetic field, acting normal to the plane of their motion. The ratio of the radii of the circular paths descirbed by the proton and α-particle is
A1:2B1:4C1:16D4:1A proton , a deuteron and an α-particle enter a magnetic field perpendicular to field with same velocity. What is the ratio of the radii of circular paths ?
A1:2:2B2:1:1C1:1:2D1:2:1A proton and an alpha particle enter the same magnetic field which is perpendicular to their velocity. If they have same kinetic energy then ratio of radii of their circular path is
A1:1B1:2C2:1D1:4
A proton and an alpha particle move in circular paths in a plane perpendicular to a uniform magnetic field. If the kinetic energy of the two particles is equal, the ratio of the radii of their paths is _________.
View SolutionA proton and an α- particle is projected with equal kinetic energy in transverse uniform magnetic field B. The ratio of radii of proton to that of alpha particle is
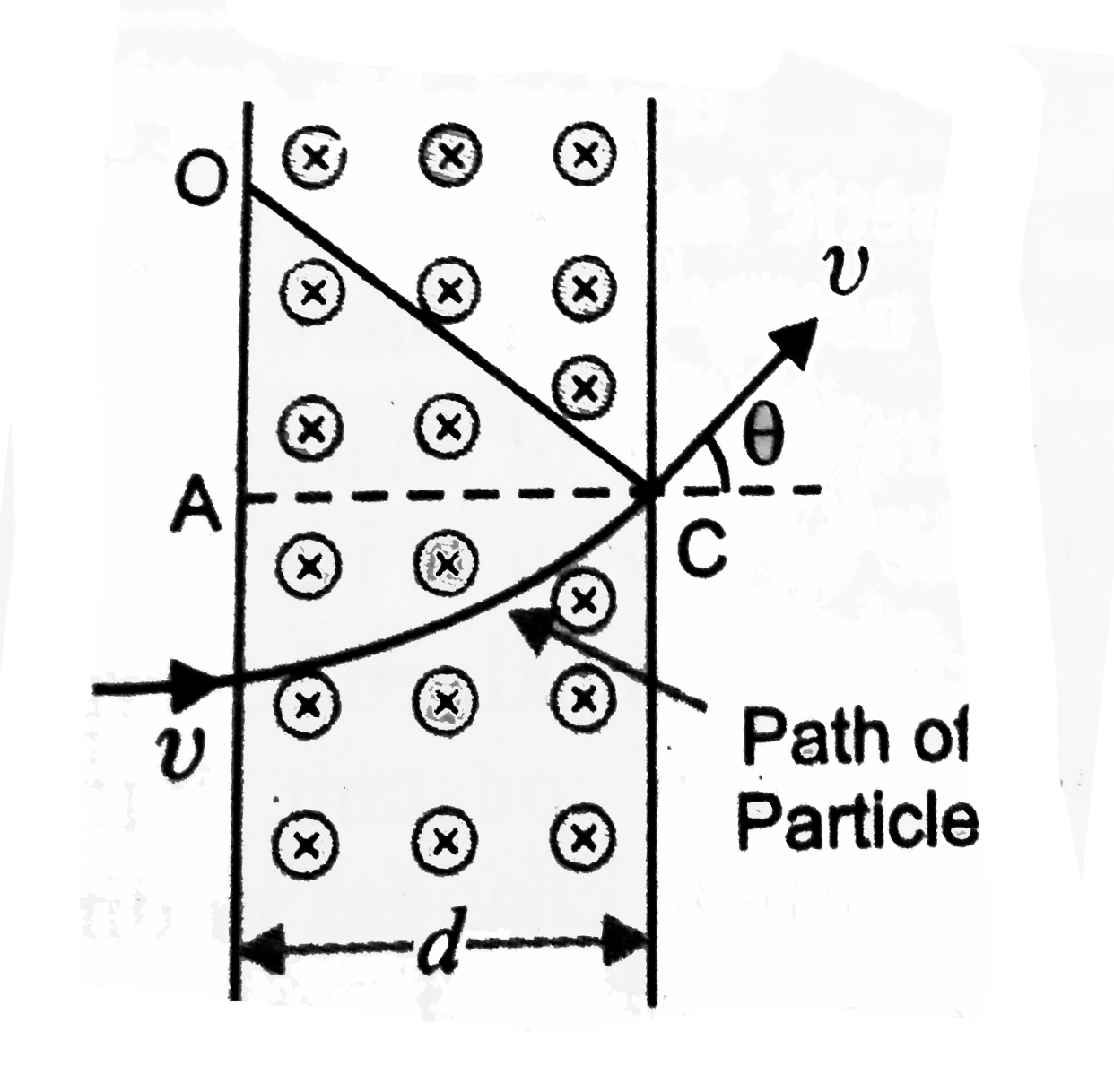
View SolutionA proton and an alpha particle are projected in a perpendicular magnetic field B which exists in a region of width d, figure. Find the ratio of the sines of angles of deviation suffered by the proton and the alpha particle if before entering the magnetic field both the particles (i) have the same momentum (ii) have the same kinetic energy.
View SolutionA proton, a deuteron and an α-particle with the same KE enter a region of uniform magnetic field, moving at right angles to B. What is the ratio of the radii of their circular paths ?
View SolutionA protn and an alpha particle enter into a uniform magnetic field with the same velocity. The period of rotation of the alpha particle will be
View Solution
