A plane meets the coordinates axes respectively at A, B and C such that the centroid of the triangle ABC is (3,3,3). If the equation of the plane is x+y+z=p. Then p is equal to Solution in Bengali
The correct Answer is:9
Step by step video, text & image solution for A plane meets the coordinates axes respectively at A, B and C such that the centroid of the triangle ABC is ( 3, 3, 3). If the equation of the plane is x+y+z=p. Then p is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PLANE BOOK - CHHAYA PUBLICATIONCHAPTER - PLANE EXERCISE - Sample Question for Competitive Examination( C Matrix Match Type )
1 Videos
- PLANE BOOK - CHHAYA PUBLICATIONCHAPTER - PLANE EXERCISE - Sample Question for Competitive Examination( D Comprehension Type )
7 Videos
- PLANE BOOK - CHHAYA PUBLICATIONCHAPTER - PLANE EXERCISE - Sample Question for Competitive Examination
5 Videos
- PERMUTATION AND COMBINATION BOOK - CHHAYA PUBLICATIONCHAPTER - PERMUTATION AND COMBINATION EXERCISE - Sample Questions for Competitive Exams E Assertion -Reason Type
2 Videos
- PROBABILITYBOOK - CHHAYA PUBLICATIONCHAPTER - PROBABILITYEXERCISE - SAMPLE QUESTIONS FOR COMPETITIVE EXAMS
18 Videos
Similar Questions
Match the following Column I and Column II
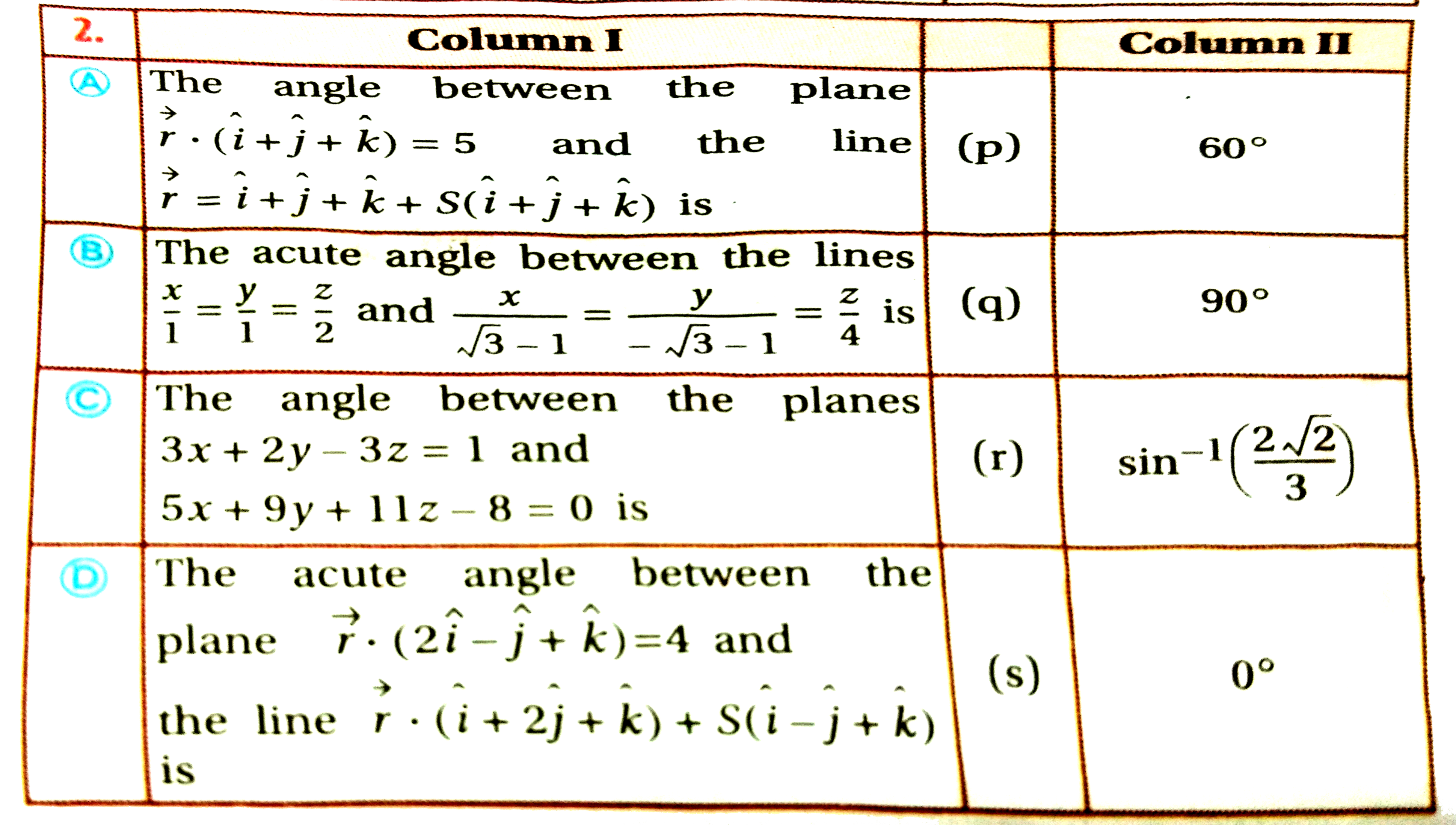
View SolutionThe distance of the point P from the plane p from the plane p is __
View SolutionConsider the plane 3x−6y+2z+5=0 and 4x−12y+3z=3. The plane 67x−162y+47z+44=0 bisects the angle between the given planes which__
View SolutionStatement -I : Number of terms in the expansion of (x+y+z+w)50 is .53C3.
Statement -II : Number of non- negative solution of the equation p+q+r+s=50 is .53C3.
View SolutionIf A and B are two independent events of a random experiment and P(A)=12/,P(B)=15, then -
View SolutionA plane meets the coordinate axes at A,BandC respectively such that the centroid of triangle ABC is (1,−2,3). Find the equation of the plane.
View SolutionA plane meets the coordinate axes in A,B,C such that eh centroid of triangle ABC is the point (p,q,r). Show that the equation of the plane is xp+yq+zr=3.
View SolutionA plane meets the coordinate axes in A,B,C such that the centroid of triangle ABC is the point (p,q,r). If the equation of the plane is xp+yq+zr=k then k=
View SolutionA plane meets the coordinates axes in A, B, C such that the centroid of the triangle ABC is the point (a,a,a) .Then the equation of the plane is x+y+z=p, where p is ___
View SolutionIF the plane meets the co-ordinate axes in A,B,C such that the centroid of the triangle ABC is the point (p,q,r) then show that the equation of the plane is xp+yq+zr=3.
View SolutionThe plane xa+yb+zc=3 meets the co-ordinate axes in A,B,C. The centroid of the triangle ABC is
View SolutionIf a plane meets the coordinate axes in A, B, C such that the centroid of the triangle ABC is the point (p,q, r) then the equation of the plane is
View SolutionA plane meets the coordinate axes at A, B, C such that the centroid of the triangle ABC is at
G(a, b, c). The equation of the plane ABC is
View SolutionA plane P meets the coordinate axes at A B and C respectively . The centroid of ΔABC is give to be (1,1,2) . Then the equation of the line through this centroid and perpendicular to the plane P is ,
View SolutionA plane cuts the coordinate axes X,Y,Z at A,B,C respectively such that the centroid of the ΔABC is (6,6,3). Then the equation of that plane is
View Solution
