If the prependicular distance of a plane from the origin is 1 and direction cosines of normal vector of the plane satisfies 1x2+1y2+1z2=K where (x,y,z ) is the centroid of the triangle make by intercept point of axis then K equals__Solution in Bengali
The correct Answer is:9
Step by step video, text & image solution for If the prependicular distance of a plane from the origin is 1 and direction cosines of normal vector of the plane satisfies (1)/(x^(2))+(1)/(y^(2))+(1)/(z^(2))=K where (x,y,z ) is the centroid of the triangle make by intercept point of axis then K equals__ by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PLANE Book:CHHAYA PUBLICATIONChapter:PLANE Exercise:Sample Question for Competitive Examination( C Matrix Match Type )
Explore 1 Video
- PLANE Book:CHHAYA PUBLICATIONChapter:PLANE Exercise:Sample Question for Competitive Examination( D Comprehension Type )
Explore 7 Videos
- PLANE Book:CHHAYA PUBLICATIONChapter:PLANE Exercise:Sample Question for Competitive Examination
Explore 5 Videos
- PERMUTATION AND COMBINATION Book:CHHAYA PUBLICATIONChapter:PERMUTATION AND COMBINATION Exercise:Sample Questions for Competitive Exams E Assertion -Reason Type
Explore 2 Videos
- PROBABILITYBook:CHHAYA PUBLICATIONChapter:PROBABILITYExercise:SAMPLE QUESTIONS FOR COMPETITIVE EXAMS
Explore 18 Videos
Similar Questions
Match the following Column I and Column II
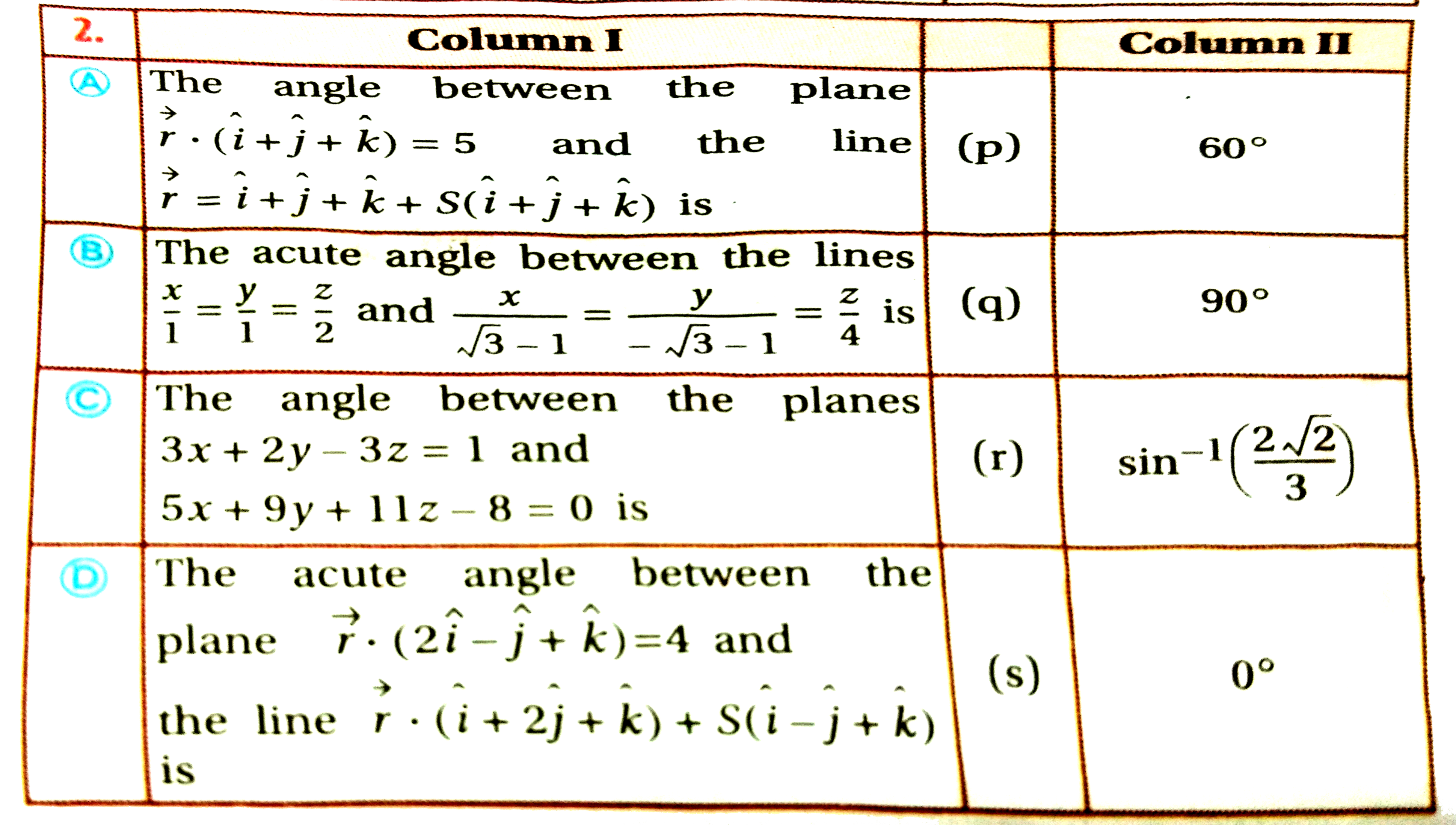
View SolutionThe distance of the point P from the plane p from the plane p is __
View SolutionConsider the plane 3x−6y+2z+5=0 and 4x−12y+3z=3. The plane 67x−162y+47z+44=0 bisects the angle between the given planes which__
View SolutionStatement -I : Number of terms in the expansion of (x+y+z+w)50 is .53C3.
Statement -II : Number of non- negative solution of the equation p+q+r+s=50 is .53C3.
View SolutionIf A and B are two independent events of a random experiment and P(A)=12/,P(B)=15, then -
View SolutionA variable plane at a distance of 1 unit from the origin cuts the axes at A, B and C. If the centroid D(x,y,z) of △ABC satisfies the relation 1x2+1y2+1z2=K, then the value of K is
View Solutionएक चर तल xa+yb+zc=1, मूलबिंदु से इकाई दुरी पर स्तिथ है तथा निर्देशाक्षों को A,B तथा C पर प्रतिछेदित करता है| केन्द्रक (x,y,z) समीकरण 1x2+1y2+1z2=k को संतुष्ट करता है, तब k का मान होगा-
View Solutionनिम्नलिखित प्रश्नो में से प्र्त्येक में समतल के अभिलम्ब की दिक् कोसाइन और मूल बिंदु से दूरी ज्ञात कीजिए -
x+y+z=1
View Solutionएक चर समतल xa+yb+zc=1, मूलबिन्दु से इकाई दूरी पर है। यह निर्देशांक अक्षों को A,B पर C प्रतिच्छेद करता है। केन्द्रक (x,y,z) समीकरण 1x2+1y2+1z2=k को सन्तुष्ट करता है। k का मान है
View Solutionएक चर समतल xa+yb+zc=1 मूलबिन्दु से इकाई दूरी पर है। यह निर्देशांक अक्षो को A,B,C पर काटता है। केन्द्रक (x,y,z) समीकरण 1x2+1y2+1z2=k को सन्तुष्ट करता है, तो k का मान ज्ञात कीजिये।
View SolutionA variable plane at a distance of 1 unit from the origin cuts the coordinate axes at A,B and C satisfies the relation 1x2+1y2+1z2=k, then the value of k is :
View SolutionDirection cosines of normal to the plane containing lines x=y=z and x−1=y−1=z−1−1 (where d∈R−{1} ,are
View Solutionযদি মূলবিন্দু থেকে কোনো সমতলের লম্বদুরত্ব 1 একক এবং ঐ সমতলের অভিলম্ব ভেক্টরের দিক কোসাইন 1x2+1y2+1z2=K সমীকরণকে সিদ্ধ করে,যেখানে (x, y, z) হল অক্ষের ছেদক বিন্দু দ্বারা তৈরি ত্রিভুজের ভরকেন্দ্র ,তবে K-এর মান হবে-
View Solutionमूलबिन्दु से एक इकाई दूरी पर स्थित चर समतल, निर्देशांक अक्षों को बिन्दु A.B तथा C पर प्रतिच्छेद करता है। यदि ΔABCका केन्द्रक D(x,y,z) सम्बन्ध 1x2+1y2+1z2=k को सन्तुष्ट करे, तो k का मान है
View SolutionA plane is at a distance of 1 unit from the origin cuts the axes in A, B, C. If the centroid D (x, y, z) of triangle ABC satisfies x−2+y−2+z−2=k find k.
View Solution