A sphere of mass m and radius r is pushed onto the fixed horizontal surface such that it rolls without slipping from the beginning. Determine the minimum speed v of its mass centre at the bottom so that it rolls completely around the loop of radius (R+r) without leaving the track in between -
 .
.
The correct Answer is:v=√277gR
at A
mg+N=mv'2R
For minimum velocity N=0
v'=√gR ….(1)
From energy conservation
12mv2(1+K2R2)=mg(2R)+12mv'2(1+K2R2)
12mv2(1+25)=mg(2R)+12mv'2(1+25)
710mv2=mg(2R)+710mv'2 ....(2)
from (1) & (2)
710v2=(2gR)+710×gR
710v2=27gR10,v=√27gR7.
 .
.
Similar Questions
A ring of mass m and radius R is placed on a rough inclined plane so that it rolls without slipping . Match the following table.


 View Solution
View SolutionA small solid marble of mass M and radius r rolls down along the loop track, without slipping. Find the height h above the base, from where it has to start rolling down the incline such that the sphere just completes the vertical circular loop of radius R.
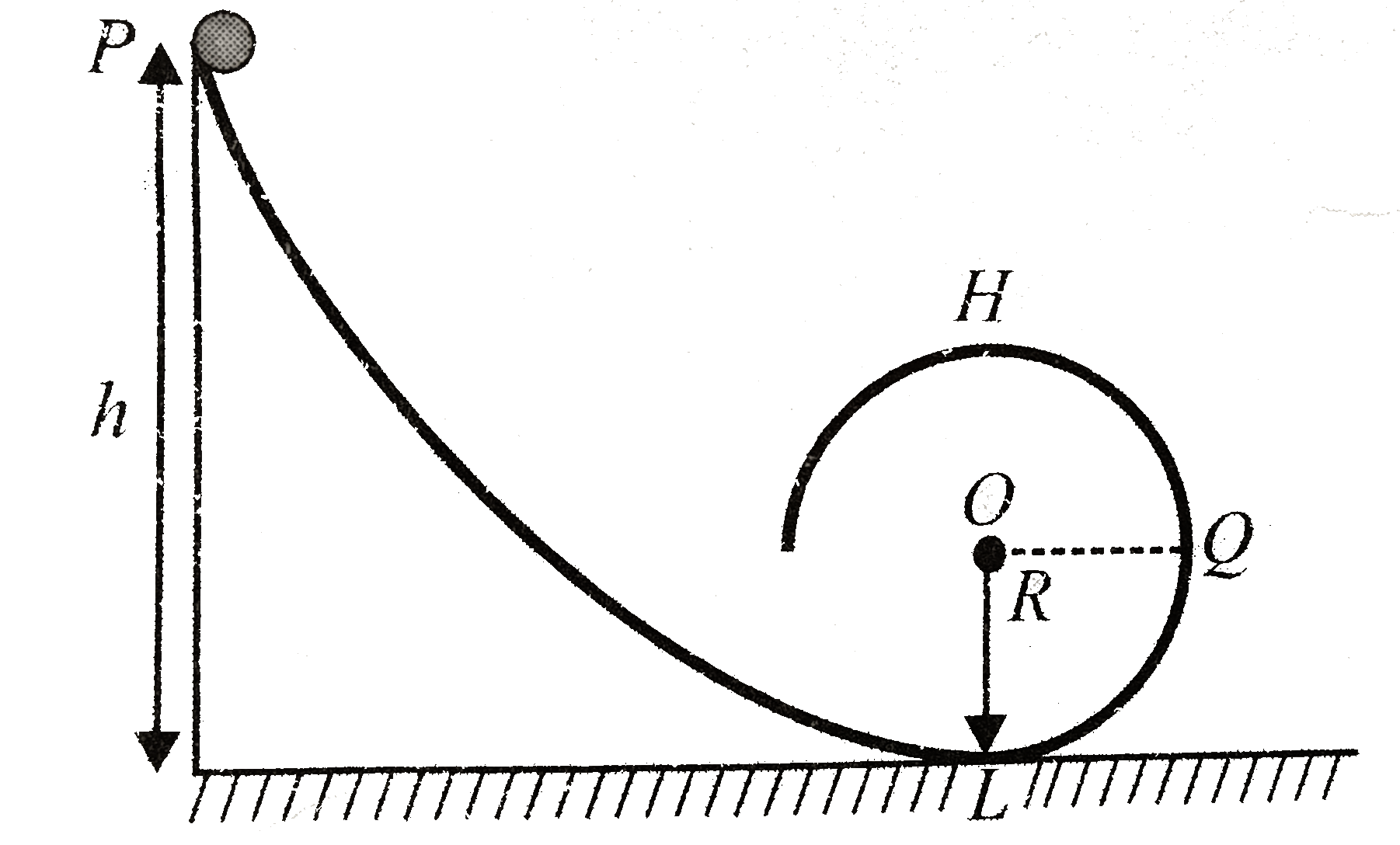 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
AThe kinetic energy of rotation is 15mv20.BThe total kinetic energy is 710mv20.CThe mechanical energy (assume the ground as reference) is mgR+710mv20.DAll options are correct. - Question 2 - Select One
A loop of mass M and radius R is rolling on a smooth horizontal surface with speed 'v'. It's total kinetic energy is
A1/2Mv2B3/2Mv2CMv2D1/2MR2ω2 - Question 3 - Select One
A ring of mass m and radius R rolls on a horizontal roudh surface without slipping due to an applied force 'F'. The frcition force acting on ring is :−
 AF3B2F3CF4DZero
AF3B2F3CF4DZero
A sphere of mass m and radius r rolls on a horizontal plane without slipping with a speed u. Now it rolls up vertically, then maximum height it would be attain will be
View SolutionA sphere of mass m and radius r rolls on a horizontal plane without slipping with the speed u . Now if it rolls up vertically , the maximum height it would attain will be
View SolutionA symmetrical body of mass M and radius R is rolling without slipping on a horizontal surface with linear speed v. Then its angular speed is
View SolutionA ring of mass M and radius R is at rest at the top of an incline as shown. The ring rolls down the plane without slipping. When the ring reaches bottom, its angular momentum about its centre of mass is:
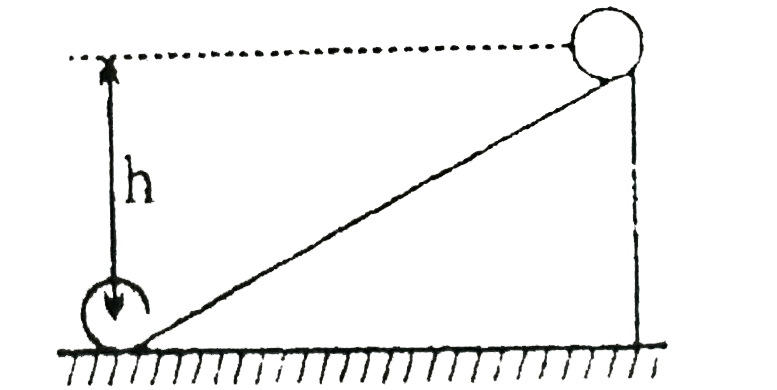 View Solution
View SolutionA solid cylinder of mass M and radius R rolls down an inclined plane of height h without slipping. The speed of its centre when it reaches the bottom is.
View Solution

