A(nonrotating) star collaps onto from an initial radius Ri with its mass remaining unchanged. Which curve in figure best gives the gravitational acceleration ag on the surface of the star as a function of the radius of the star during the collapse?
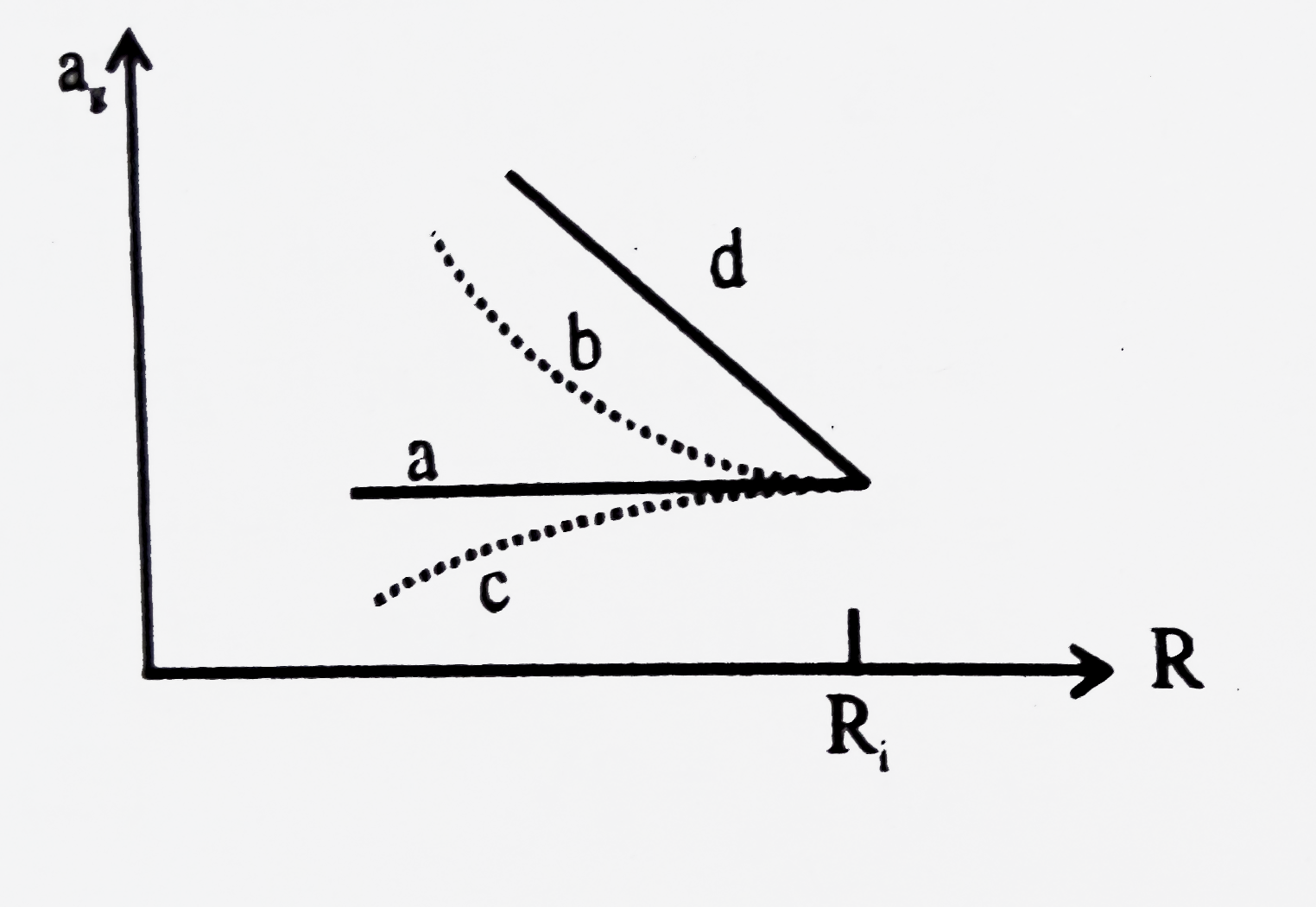
a
b
c
d
The correct Answer is:b
g=GMR2
Similar Questions
Two stars of mass M1&M2 are in circular orbits around their centre of mass The star of mass M1 has an orbit of radius R1 the star of mass M2 has an orbit of radius R2 (assume that their centre of mass is not acceleration and distance between starts is fixed)
(a) Show that the ratio of orbital radii of the two stars equals the reciprocal of the ratio of their masses, that is R1/R2=M2/M1
(b) Explain why the two stars have the same orbital period and show that the period
T=2π(R1+R2)3/2√G(M1+M2) .View SolutionA satellite of mass m revolves around the earth of radius R at a height x from its surface. If g is the acceleration due to gravity on the surface of the earth, the orbital speed of the satellite is
View Solution
Knowledge Check
- Question 1 - Select One
A spherical hole of radius R/2 is excavated from the asteroid of mass M as shown in the figure the gravitational acceleration at a point on the surface of the asteroid just above the excavation is
 AGMR2BGM2R2CGM8k2D7GM8R2
AGMR2BGM2R2CGM8k2D7GM8R2 - Question 2 - Select One
A uniform solid sphere of radius R produces a gravitational acceleration ag on its surface. At what two distances from the centre of the sphere the acceleration due to gravity is ag/4 ?
A4R, 0.50RB2R, 0.25RC3R, 0.33RD2R, 0.50R - Question 3 - Select One
The kinetic energy needed to project a body of mass m from the earth's surface to infinity is (R is radius of the earth, g is gravitational acceleration on the surface of the earth)
A14mgRB12mgRCmg RD2 mg R
A satellite of mass m revolves around the earth of radius R at a hight x from its surface. If g is the acceleration due to gravity on the surface of the earth, the orbital speed of the satellite is
View SolutionIf the density of a planet is constant, then the curve between value of g on its surface and its radius r will be-
View SolutionAssuming the earth to be a homogencous sphere of radius R. its density in terms of G (constant of gravitation) and g (acceleration due to gravity on the surface of the earth )is
View SolutionA star of mass M and radius R is made up of gases. The average gravitational pressure compressing the star due to gravitational pull of the gases making up the star depends on R as
View SolutionAssuming the earth to be a homogeneous sphere of radius R, its density in terms of G (constant of gravitation) and g (acceleration due to gravity on the surface of the earth)
View Solution

